Skip to main content
SpringerLink
Log in

Legendre spectral collocation in space and time for PDEs

  • Published:
Numerische Mathematik Aims and scope Submit manuscript

Abstract

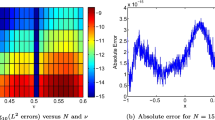
Spectral methods solve partial differential equations numerically with errors bounded by an exponentially decaying function of the number of modes when the solution is analytic. For time dependent problems, almost all focus has been on low-order finite difference schemes for the time derivative and spectral schemes for spatial derivatives. Spectral methods which converge spectrally in both space and time have appeared recently. This paper shows that a Legendre spectral collocation method of Tang and Xu for the heat equation converges exponentially quickly when the solution is analytic. We also derive a condition number estimate of the method. Another space-time spectral scheme which is easier to implement is proposed. Numerical experiments verify the theoretical results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  1. Bartels, R.H., Stewart, G.W.: A solution of the equation \(AX+XB=C\). Commun. ACM 15, 820–826 (1972)

    Article  Google Scholar 

  2. Bernardi, C., Maday, Y.: Spectral methods. In: Ciarlet, P.G., Lions, J.L. (eds.) Handbook of Numerical Analysis. pp, vol. 5, pp. 209–485. North-Holland, Amsterdam (1997)

    Google Scholar 

  3. Boyd, J.P.: Chebyshev and Fourier Spectral Methods, 2nd Rev. Ed. Dover, Mineola (2001)

  4. Brugnano, L., Iavernaro, F., Trigiante, D.: Analysis of Hamiltonian boundary value methods (HBVMs): a class of energy-preserving Runge-Kutta methods for the numerical solution of polynoial Hamiltonian systems. Commum. Nonlinear Sci. Numer. Simul. 20, 650–667 (2015)

    Article  MATH  Google Scholar 

  5. Canuto, C., Hussaini, M.Y., Quarteroni, A., Zang, T.A.: Spectral Methods-Fundamentals in Single Domains. Springer, New York (2006)

    MATH  Google Scholar 

  6. Carpenter, M.H., Gottlieb, D.: Spectral methods on arbitrary grids. J. Comput. Phys. 129, 74–86 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  7. Christlieb, A.J., Haynes, R.D., Ong, B.W.: A parallel space-time algorithm. SIAM J. Sci. Stat. Comput. 34, C233–C248 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  8. Dai, X., Maday, Y.: Stable parareal in time method for first- and second-order hyperbolic systems. SIAM J. Sci. Comput. 35, A52–A78 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  9. Dutt, A., Greengard, L., Rokhlin, V.: Spectral deferred correction methods for ordinary differential equations. BIT 40, 241–266 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  10. Falgout, R.D., Friedhoff, S., Kolev, Tz.V., Maclachlan, S.P., Schroder, J.B.: Parallel time integration with multigrid. SIAM J. Sci. Comput. 36, C625–C661 (2014)

  11. Fornberg, B.: A Practical Guide to Pseudospectral Methods. Cambridge University Press, Cambridge (1996)

    Book  MATH  Google Scholar 

  12. Funaro, D.: Spectral Elements for Transport-Dominated Equations. Springer, Berlin (1997)

    Book  MATH  Google Scholar 

  13. Gander, M.J., Vandewalle, S.: Analysis of the parareal time-parallel time-integration method. SIAM J. Sci. Comput. 29, 556–578 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  14. Golub, G.H., Nash, S., Van Loan, C.F.: Hessenberg–Schur method for the problem \(ax+xb=c\). IEEE Trans. Autom. Control, AC-24, pp. 909–913 (1979)

  15. Gottlieb, D., Orszag, S.A.: Numerical Analysis of Spectral Methods. SIAM, Philadelphia (1977)

    Book  MATH  Google Scholar 

  16. Guo, B.Y.: Spectral Methods and Their Applications. World Scientific, Singapore (1998)

    Book  MATH  Google Scholar 

  17. Guo, B.-Y., Wang, Z.-Q.: Legendre-Gauss collocation methods for ordinary differential equations. Adv. Comput. Math. 30, 249–280 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  18. Hesthaven, J., Gottlieb, S., Gottlieb, D.: Spectral Methods for Time-Dependent Problems. Cambridge University Press, Philadelphia (2007)

    Book  MATH  Google Scholar 

  19. Horton, G., Vandewalle, S.: A space-time multigrid method for parabolic partial differential equations. SIAM J. Sci. Comput. 16, 848–864 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  20. Liu, W., Sun, J., Wu, B.: Galerkin–chebyshev spectral method and block boundary value methods for two-dimensional semilinear parabolic equations. Numer. Algorithms 71, 437–455 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  21. Liu, W., Wu, B., Sun, J.: Space-time spectral collocation method for the one-dimensional Sine–Gordon equation. Numer. Methods PDEs 31, 670–690 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  22. Lui, S.H.: Numerical Analysis of Partial Differential Equations. Wiley, Hoboken (2011)

    Book  MATH  Google Scholar 

  23. McDonald, E.G., Wathen, A.J.: A simple proposal for parallel computation over time of an evolutionary process with implicit time stepping. Technical report, The Mathematical Institute, University of Oxford Technical Report, vol. 1860 (2014)

  24. Shen, J., Tang, T., Wang, L.-L.: Spectral Methods. Springer, Berlin (2011)

    Book  MATH  Google Scholar 

  25. Shen, J., Wang, L.-L.: Fourierization of the Legendre–Galerkin method and a new space-time spectral method. Appl. Numer. Math. 57, 710–720 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  26. Tal-Ezer, H.: Spectral methods in time for hyperbolic equations. SIAM J. Numer. Anal. 23, 11–26 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  27. Tal-Ezer, H.: Spectral methods in time for parabolic problems. SIAM J. Numer. Anal. 26, 1–11 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  28. Tang, J.-G., Ma, H.-P.: Single and multi-interval Legendre \(\tau \)-methods in time for parabolic equations. Adv. Comput. Math. 17, 349–367 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  29. Tang, J.-G., Ma, H.-P.: A Legendre spectral method in time for first-order hyperbolic equations. Appl. Numer. Math. 57, 1–11 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  30. Tang, T., Xu, X.: Accuracy enhancement using spectral postprocessing for differential equations and integral equations. Commun. Comput. Phys. 5, 779–792 (2009)

    MathSciNet  Google Scholar 

  31. Trefethen, L.N.: Spectral Methods in Matlab. SIAM, Philadelphia (2000)

    Book  MATH  Google Scholar 

  32. Wang, L.-L., Samson, M.D., Zhao, X.: A well-conditioned collocation method using a pseudospectral integration matrix. SIAM J. Sci. Comput. 36, 907–929 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  33. Wu, S., Liu, X.: Convergence of spectral method in time for Burgers’ equation. Acta Math. Appl. Sin. 13, 314–320 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  34. Xie, Z., Wang, L.-L., Zhao, X.: On exponential convergence of Gegenbauer interpolation and spectral differentiation. Math. Comput. 82, 1017–1036 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  35. Yi, L., Wang, Z.: Legendre–Gauss-type spectral collocation algorithms for nonlinear ordinary/partial differential equations. Int. J. Comput. Math. 91, 1434–1460 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  36. Yi, L., Wang, Z.: Legendre spectral collocation method for second-order nonlinear ordinary/partial differential equations. Discrete Contin. Dyn. Syst. B 19, 299–322 (2014)

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgments

I am indebted to Professor Lijun Yi for discussions of this work and for his pointers to the literature. I also thank Tim Hoffman for commenting on an earlier draft of this paper. Finally, I am grateful to the referees for their careful reading of the manuscript and for their numerous suggestions which have improved this paper.

Author information

Authors and Affiliations

  1. Department of Mathematics, University of Manitoba, Winnipeg, MB, R3T 2N2, Canada

    S. H. Lui

Authors
  1. S. H. Lui
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to S. H. Lui.

Additional information

This work was in part supported by a Grant from NSERC.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Lui, S.H. Legendre spectral collocation in space and time for PDEs. Numer. Math. 136, 75–99 (2017). https://doi.org/10.1007/s00211-016-0834-x

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00211-016-0834-x

Mathematics Subject Classification

Search

Navigation