Suppression of vibration transmission in coupled systems with an inerter-based nonlinear joint

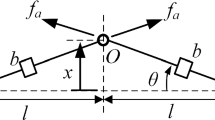
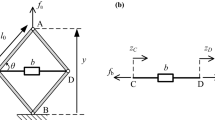
Abstract

This study proposes an inerter-based nonlinear passive joint device and investigates its performance in suppression of vibration transmission in coupled systems. The joint device comprises an axial inerter and a pair of lateral inerters creating geometric nonlinearity, with the nonlinear inertance force being a function of the relative displacement, velocity, and acceleration of the two terminals. Both analytical approximations based on the harmonic balance method and numerical integration are used to obtain the steady-state response amplitude. Force transmissibility and time-averaged energy flow variables are used as performance indices to evaluate the vibration transmission in the coupled system, with subsystems representing the dominant modes of interactive engineering structures. The effects of adding the proposed joint to the force-excited subsystem or to the coupling interface of subsystems on suppression performance are examined. It is found that the insertion of the inerter-based nonlinear joint can shift and bend response peaks to lower frequencies, substantially reducing the vibration of the subsystems at prescribed frequencies. By adding the joint device, the level of vibration force and energy transmission between the subsystems can be attenuated in the range of excitation frequencies of interest. It is shown that the inerter-based nonlinear joint can be used to introduce an anti-peak in the response curve and achieve substantially lower levels of force transmission and a reduced amount of energy transmission between subsystems. This work provides an in-depth understanding of the effects of inerter-based nonlinear devices on vibration attenuation and benefits enhanced designs of coupled systems for better dynamic performance.

Appendix: Property of the power transmission ratio

When the inerter-based joint is added at position Q, the power transmission ratio ${R}_{t}$ from subsystems S1 to S2 is not sensitive to changes in the inertance $\lambda_{0}$ and $\lambda_{1}$ as shown in Figs. 15b and 18b. Here, the reasons are demonstrated with mathematical derivations for the case when the inerter-based joint is added at position Q, and the lateral inerters are with $\lambda_{1} = 0$. Note that Eq. (6) can be rearranged as:

$$ - {\Omega }^{2} \tilde{X}_{1} + 2\zeta_{1} {{{\text{i}}\Omega }}\tilde{X}_{1} + \tilde{X}_{1} + \kappa \tilde{\Delta } - {\Omega }^{2} \lambda_{0} \tilde{X}_{1} = F_{0} \exp \left( {{\text{i}}\phi } \right), $$

(39a)

$$ - {\Omega }^{2} \mu \left( {\tilde{X}_{1} - \tilde{\Delta }} \right) + 2\zeta_{2} {{{\text{i}}\Omega }}\mu \left( {\tilde{X}_{1} - \tilde{\Delta }} \right) + \gamma \left( {\tilde{X}_{1} - \tilde{\Delta }} \right) - \kappa \tilde{\Delta } = 0, $$

(39b)

where $\tilde{X}_{1}$ and $\tilde{\Delta }$ denotes the complex amplitude of the response of mass $m_{1}$ and that of the relative displacement amplitude. According to Eq. (39a) and (39b), the expression of response amplitude can be derived as:

$$ \tilde{X}_{1} = \frac{{F_{0} \exp \left( {{\text{i}}\phi } \right)\left( { - {\Omega }^{2} \mu + 2\zeta_{2} {{{\text{i}}\Omega }}\mu + \gamma + \kappa } \right)}}{{\left( { - {\Omega }^{2} + 2\zeta_{1} {{{\text{i}}\Omega }} + 1 - {\Omega }^{2} \lambda_{0} } \right)\left( { - {\Omega }^{2} \mu + 2\zeta_{2} {{{\text{i}}\Omega }}\mu + \gamma + \kappa } \right) + \kappa \left( { - {\Omega }^{2} \mu + 2\zeta_{2} {{{\text{i}}\Omega }}\mu + \gamma } \right)}}, $$

(40a)

$$ \tilde{\Delta } = \frac{{F_{0} \exp \left( {{\text{i}}\phi } \right)\left( { - {\Omega }^{2} \mu + 2\zeta_{2} {{{\text{i}}\Omega }}\mu + \gamma } \right)}}{{\left( { - {\Omega }^{2} + 2\zeta_{1} {{{\text{i}}\Omega }} + 1 - {\Omega }^{2} \lambda_{0} } \right)\left( { - {\Omega }^{2} \mu + 2\zeta_{2} {{{\text{i}}\Omega }}\mu + \gamma + \kappa } \right) + \kappa \left( { - {\Omega }^{2} \mu + 2\zeta_{2} {{{\text{i}}\Omega }}\mu + \gamma } \right)}}, $$

(40b)

$$ \tilde{X}_{2} = \tilde{X}_{1} - \tilde{\Delta } = \frac{{F_{0} \exp \left( {{\text{i}}\phi } \right)\kappa }}{{\left( { - {\Omega }^{2} + 2\zeta_{1} {{{\text{i}}\Omega }} + 1 - {\Omega }^{2} \lambda_{0} } \right)\left( { - {\Omega }^{2} \mu + 2\zeta_{2} {{{\text{i}}\Omega }}\mu + \gamma + \kappa } \right) + \kappa \left( { - {\Omega }^{2} \mu + 2\zeta_{2} {{{\text{i}}\Omega }}\mu + \gamma } \right)}}. $$

(40c)

The transmitted force to subsystem S2 can be expressed by:

$$ \widetilde{{F_{t} }} = \kappa \tilde{\Delta }. $$

(41)

The time-averaged input power and transmitted power over a period of oscillation are:

$$ \overline{P}_{{{\text{in}}}} = \frac{1}{T}\mathop \smallint \limits_{{t_{0} }}^{{t_{0} + T}} {\text{Re}}\left\{ {p_{in} } \right\}{\text{d}}t = \frac{1}{2}{\text{Re}}\left\{ {\left( {F_{0} \exp \left( {{\text{i}}\phi } \right)} \right)^{*} X_{1} {{{\text{i}}\Omega }}} \right\}, $$

(42a)

$$ \overline{P}_{{\text{t}}} = \frac{1}{T}\mathop \smallint \limits_{{t_{0} }}^{{t_{0} + T}} {\text{Re}}\left\{ {p_{t} } \right\}{\text{d}}t = \frac{1}{2}{\text{Re}}\left\{ {\widetilde{{F_{t} }}^{*} X_{2} {{{\text{i}}\Omega }}} \right\}, $$

(42b)

where ${*}$ denotes the complex conjugate. The power transmission ratio from subsystem one to subsystem two is defined as:

$$ R_{t} = \frac{{\overline{P}_{{\text{t}}} }}{{\overline{P}_{{{\text{in}}}} }}. $$

(43)

Based on Eqs. (40)–(43), the power transmission ratio $R_{t}$ can be calculated:

$$ R_{t} = \frac{{\overline{P}_{{\text{t}}} }}{{\overline{P}_{{{\text{in}}}} }} = \frac{{\kappa^{2} 2\zeta_{2} {\Omega }\mu }}{{\left( { - {\Omega }^{2} \mu + \gamma + \kappa } \right)\left( { - {\Omega }^{2} \mu + \gamma + \kappa } \right)2\zeta_{1} {\Omega } + 2\kappa \zeta_{2} {\Omega }\mu \kappa }}, $$

(44)

where $\lambda_{0}$ is eliminated suggesting that the change in ${\lambda }_{0}$ will not affect the power transmission ratio from subsystem S1 to subsystem S2.