Skip to main content
SpringerLink
Log in

Serendipity Virtual Elements for General Elliptic Equations in Three Dimensions

  • Published:
Chinese Annals of Mathematics, Series B Aims and scope Submit manuscript

Abstract

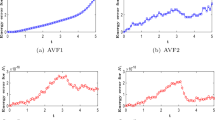
The authors study the use of the virtual element method (VEM for short) of order k for general second order elliptic problems with variable coefficients in three space dimensions. Moreover, they investigate numerically also the serendipity version of the VEM and the associated computational gain in terms of degrees of freedom.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Ahmad, B., Alsaedi, A., Brezzi, F., et al., Equivalent projectors for virtual element methods, Comput. Math. Appl., 66(3), 2013, 376–391.

    Article  MathSciNet  MATH  Google Scholar 

  2. Antonietti, P. F., Beirão da Veiga, L., Scacchi, S. and Verani, M., A C 1 virtual element method for the Cahn-Hilliard equation with polygonal meshes, SIAM J. Numer. Anal., 54(1), 2016, 34–57.

    Article  MathSciNet  MATH  Google Scholar 

  3. Artioli, E., de Miranda, C., Lovadina, C. and Patruno, L., A stress/displacement virtual element method for plane elasticity problems, Computer Methods in Applied Mechanics and Engineering, 325, 2017, 155–174.

    Article  MathSciNet  Google Scholar 

  4. Ayuso, B., Lipnikov, K. and Manzini, G., The nonconforming virtual element method, ESAIM: M2AN, 50(3), 2016, 879–904.

    Article  MathSciNet  MATH  Google Scholar 

  5. Beirão da Veiga, L., Brezzi, F., Cangiani, A., et al., Basic principles of virtual element methods, Math. Models Methods Appl. Sci., 23(1), 2013, 199–214.

    Article  MathSciNet  MATH  Google Scholar 

  6. Beirão da Veiga, L., Brezzi, F., Dassi, F., et al., Virtual element approximation of 2d magnetostatic problems, Comput. Methods Appl. Mech. Engrg., 327, 2017, 173–195.

    Article  MathSciNet  Google Scholar 

  7. Beirão da Veiga, L., Brezzi, F., Marini, L. D. and Russo, A., The hitchhiker’s guide to the virtual element method, Math. Models Methods Appl. Sci., 24(8), 2014, 1541–1573.

    Article  MathSciNet  MATH  Google Scholar 

  8. Beirão da Veiga, L., Brezzi, F., Marini, L. D. and Russo, A., Virtual element methods for general second order elliptic problems on polygonal meshes, Math. Models Methods Appl. Sci., 26(4), 2016, 729–750.

    Article  MathSciNet  MATH  Google Scholar 

  9. Beirão da Veiga, L., Brezzi, F., Marini, L. D. and Russo, A., Serendipity nodal VEM spaces, Comp. Fluids, 141, 2016, 2–12.

    Article  MathSciNet  MATH  Google Scholar 

  10. Beirão da Veiga, L., Dassi, F. and Russo, A., High-order virtual element method on polyhedral meshes, Computers & Mathematics with Applications, 74(5), 2017, 1110–1122.

    Article  MathSciNet  Google Scholar 

  11. Beirão da Veiga, L., Lovadina, C. and Russo, A., Stability analysis for the virtual element method, Math. Models Methods Appl. Sci., 27(13), 2017, 2557–2594.

    Article  MathSciNet  MATH  Google Scholar 

  12. Beirão da Veiga, L., Lovadina, C. and Vacca, G., Divergence free Virtual Elements for the Stokes problem on polygonal meshes, ESAIM Math. Model. Numer. Anal., 51, 2017, 509–535.

    Article  MathSciNet  MATH  Google Scholar 

  13. Benedetto, M. F., Berrone, S., Borio, A., et al., A hybrid mortar virtual element method for discrete fracture network simulations, Journal of Computational Physics, 306, 2016, 148–166.

    Article  MathSciNet  MATH  Google Scholar 

  14. Berrone, S. and Borio, A., Orthogonal polynomials in badly shaped polygonal elements for the virtual element method, Finite Elem. Anal. Des., 129, 2017, 14–31.

    Article  MathSciNet  Google Scholar 

  15. Brenner, S., Guan, Q. and Sung, L., Some estimates for virtual element methods, Computational Methods in Applied Mathematics, 17, 2017, 553–574.

    Article  MathSciNet  Google Scholar 

  16. Brezzi, F. and Marini, L. D., Virtual element methods for plate bending problems, Comput. Methods Appl. Mech. Engrg., 253, 2013, 455–462.

    Article  MathSciNet  MATH  Google Scholar 

  17. Cáceres, E. and Gatica, G. N., A mixed virtual element method for the pseudostress-velocity formulation of the Stokes problem, IMA J. Numer. Anal., 37(1), 2017, 296–331.

    Article  MathSciNet  Google Scholar 

  18. Cangiani, A., Georgoulis, E. H., Pryer, T. and Sutton, O. J., A posteriori error estimates for the virtual element method, Numerische Mathematik, 137, 2017, 857–893.

    Article  MathSciNet  MATH  Google Scholar 

  19. Chi, H., Beirão da Veiga, L. and Paulino, G. H., Some basic formulations of the virtual element method (VEM) for finite deformations, Computer Methods in Applied Mechanics and Engineering, 318, 2017, 148–192.

    Article  MathSciNet  Google Scholar 

  20. Ciarlet, P. G., The finite element method for elliptic problems, Studies inMathematics and Its Applications, 4, North-Holland Publishing Co., Amsterdam, New York, Oxford, 1978.

    Google Scholar 

  21. Du, Q., Faber, V. and Gunzburger, M., Centroidal voronoi tessellations: Applications and algorithms, SIAM Rev., 41(4), 1999, 637–676.

    Article  MathSciNet  MATH  Google Scholar 

  22. Gain, A. L., Paulino, G. H., Leonardo, S. D. and Menezes, I. F. M., Topology optimization using polytopes, Comput. Methods Appl. Mech. Engrg., 293, 2015, 411–430.

    Article  MathSciNet  Google Scholar 

  23. Gain, A. L., Talischi, C. and Paulino, G. H., On the Virtual Element Method for three-dimensional linear elasticity problems on arbitrary polyhedral meshes, Comput. Methods Appl. Mech. Engrg., 282, 2014, 132–160.

    Article  MathSciNet  Google Scholar 

  24. Mascotto, L., A therapy for the ill-conditioning in the virtual element method, ArXiv: 1705.10581.

  25. Mascotto, L., Beirão da Veiga, L., Chernov, A. and Russo, A., Exponential convergence of the hp virtual element method with corner singularities, Numer. Math., DOI: 10.1007/s00211-017-0921-7.

  26. Mascotto, L. and Dassi, F., Exploring high-order three dimensional virtual elements: Bases and stabilizations, 2017, arXiv: 1709.04371.

    Google Scholar 

  27. Mora, D., Rivera, G. and Rodríguez, R., A virtual element method for the Steklov eigenvalue problem, Math. Models Methods Appl. Sci., 25(8), 2015, 1421–1445.

    Article  MathSciNet  MATH  Google Scholar 

  28. Rycroft, C. H., Voro++: A three-dimensional voronoi cell library in C++, Chaos, 19(4), 2009, 041111.

    Article  Google Scholar 

  29. Schatz, A. H., An observation concerning Ritz-Galerkin methods with indefinite bilinear forms, Math. Comp., 28, 1974, 959–962.

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Dipartimento di Matematica e Applicazioni, Università di Milano-Bicocca, Via Cozzi 53, I-20153, Milano, Italy

    Lourenço Beirão Da Veiga, Franco Dassi & Alessandro Russo

  2. IMATI CNR, Via Ferrata 1, I-27100, Pavia, Italy

    Lourenço Beirão Da Veiga, Franco Brezzi & Luisa Donatelia Marini

  3. Dipartimento di Matematica, Università di Pavia, Via Ferrata 5, I-27100, Pavia, Italy

    Luisa Donatelia Marini

Authors
  1. Lourenço Beirão Da Veiga
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Franco Brezzi
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Franco Dassi
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Luisa Donatelia Marini
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Alessandro Russo
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Lourenço Beirão Da Veiga.

Additional information

Dedicated to Philippe G. Ciarlet on the occasion of his 80th birthday

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Beirão Da Veiga, L., Brezzi, F., Dassi, F. et al. Serendipity Virtual Elements for General Elliptic Equations in Three Dimensions. Chin. Ann. Math. Ser. B 39, 315–334 (2018). https://doi.org/10.1007/s11401-018-1066-4

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11401-018-1066-4

Keywords

2000 MR Subject Classification

Search

Navigation