Skip to main content
SpringerLink
Log in

Hybrid-Mixed Low-Order Finite Elements for Geometrically Exact Shell Models: Overview and Comparison

  • Review article
  • Published:
Archives of Computational Methods in Engineering Aims and scope Submit manuscript

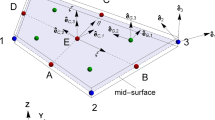
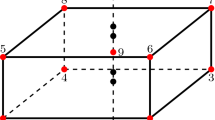
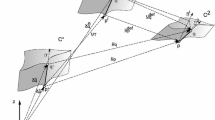
Abstract

We critically review and compare fifteen mixed and mixed-hybrid nonlinear shell finite element formulations with 4 nodes in order to identify those that are closest to the “optimal” one. We consider formulations that are based either on Assumed Natural Strain concept, Enhanced Assumed Strain concept, Hellinger–Reissner functional or Hu–Washizu functional, and those that effectively combine several of the mentioned approaches. Most of the formulations are state-of-the-art, but some are also presented for the first time. We show that the flexibility of mixed-hybrid formulations requires careful consideration of theoretical and numerical aspects in the process of design of a high-performance element. We make extensive numerical experiments in order to assess convergence properties, mesh distortion sensitivity, and computational speed (that is associated with the ability to achieve large steps during the solution search) of the considered formulations. We show that the application of the idea to combine mixed-hybrid formulations of the Hellinger–Reissner and Hu–Washizu type with the Assumed Natural Strain and/or the Enhanced Assumed Strain concepts generates the formulations that perform excellent. We also identify the formulations that are currently closest to the “optimal” one.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24
Fig. 25
Fig. 26
Fig. 27
Fig. 28
Fig. 29

Similar content being viewed by others

References

  1. Abaqus (2018) Abaqus Manuals. Dassoult Systems, Providence

    Google Scholar 

  2. Andelfinger U, Ramm E (1993) EAS-elements for two-dimensional, three-dimensional, plate and shell structures and their equivalence to HR-elements. Int J Numer Methods Eng 36:1311–1337. https://doi.org/10.1002/nme.1620360805

    Article  MATH  Google Scholar 

  3. Basar Y, tskov M, Eckstein A (2000) Composite laminates: nonlinear interlaminar stress analysis by multi-layer shell elements. Comput Methods Appl Mech Eng 185(2–4):367–397. https://doi.org/10.1016/S0045-7825(99)00267-4

    Article  MATH  Google Scholar 

  4. Bathe KJ (2014) Finite Element Procedures, 2nd edn. KJ Bathe, Watertown

    MATH  Google Scholar 

  5. Bathe KJ, Dvorkin EN (1986) A formulation of general shell elements—the use of mixed interpolation of tensorial components. Int J Numer Meth Eng 22(3):697–722. https://doi.org/10.1002/nme.1620220312

    Article  MATH  Google Scholar 

  6. Bathe KJ, Iosilevich A, Chapelle D (2000) An evaluation of the MITC shell elements. Comput Struct 75:1–30. https://doi.org/10.1016/S0045-7949(99)00214-X

    Article  Google Scholar 

  7. Baumann M, Schweizerhof K, Andrussow S (1994) An efficient mixed hybrid 4-node shell element with assumed stresses for membrane, bending and shear parts. Eng Comput 11(1):69–80. https://doi.org/10.1108/02644409410799164

    Article  Google Scholar 

  8. Benson D, Bazilevs Y, Hsu M, Hughes TJ (2010) Isogeometric shell analysis: the Reissner-Mindlin shell. Comput Methods Appl Mech Eng 199(5–8):276–289. https://doi.org/10.1016/J.CMA.2009.05.011

    Article  MathSciNet  MATH  Google Scholar 

  9. Benson D, Bazilevs Y, Hsu M, Hughes TJ (2011) A large deformation, rotation-free, isogeometric shell. Comput Methods Appl Mech Eng 200(13–16):1367–1378. https://doi.org/10.1016/j.cma.2010.12.003

    Article  MathSciNet  MATH  Google Scholar 

  10. Betsch P, Gruttmann F, Stein E (1996) A 4-node finite shell element for the implementation of general hyperelastic 3D-elsticity at finite strains. Comput Methods Appl Mech. Engrg 130(1–2):57–79. https://doi.org/10.1016/0045-7825(95)00920-5

    Article  MathSciNet  MATH  Google Scholar 

  11. Betsch P, Menzel A, Stein E (1998) On the parametrization of finite rotations in computational mechanics A classification of concepts with application to smooth shells. Comput Methods Appl Mech Engrg 155:273–305. https://doi.org/10.1016/S0045-7825(97)00158-8

    Article  MathSciNet  MATH  Google Scholar 

  12. Betsch P, Stein E (1995) An assumed strain approach avoiding artificial thickness straining for a nonlinear 4-node shell element. Commun Numer Methods Eng 11:899–909. https://doi.org/10.1002/cnm.1640111104

    Article  MATH  Google Scholar 

  13. Bischoff M, Ramm E (1997) Shear deformable shell elements for large strains and rotations. Int J Numer Meth Eng 40(23):4427–4449. https://doi.org/10.1002/(SICI)1097-0207(19971215)40:23%3c4427:AID-NME268%3e3.0.CO;2-9

    Article  MATH  Google Scholar 

  14. Bischoff M, Ramm E, Braess D (1999) A class of equivalent enhanced assumed strain and hybrid stress finite elements. Comput Mech 22:443–449. https://doi.org/10.1007/s004660050378

    Article  MATH  Google Scholar 

  15. Brank B (2008) Assessment of 4-node EAS-ANS shell elements for large deformation analysis. Comput Mech 42:39–51. https://doi.org/10.1007/s00466-007-0233-3

    Article  MATH  Google Scholar 

  16. Brank B, Ibrahimbegovic A (2001) On the relation between different parametrizations of finite rotations for shells. Eng Comput 18:950–973. https://doi.org/10.1108/02644400110403984

    Article  MATH  Google Scholar 

  17. Brank B, Korelc J, Ibrahimbegovic A (2002) Nonlinear shell problem formulation accounting for through-the-thickness stretching and its finite element implementation. Comput Struct 80(9–10):699–717. https://doi.org/10.1016/S0045-7949(02)00042-1

    Article  Google Scholar 

  18. Brank B, Perić D, Damjanić FB (1997) On large deformations of thin elasto-plastic shells: implementation of a finite rotation model for quadrilateral shell element. Int J Numer Methods Eng 40:689–726. https://doi.org/10.1002/(SICI)1097-0207(19970228)40:4%3c689:AID-NME85%3e3.0.CO;2-7

    Article  MATH  Google Scholar 

  19. César de Sá JMA, Natal Jorge RM, Fontes Valente RA, Almeida Areias PM (2002) Development of shear locking-free shell elements using an enhanced assumed strain formulation. Int J Numer Methods Eng 53(7):1721–1750. https://doi.org/10.1002/nme.360

    Article  MATH  Google Scholar 

  20. Choi CK, Paik JG (1996) An effective four node degenerated shell element for geometrically nonlinear analysis. Thin-Walled Structures 24(3):261–283. https://doi.org/10.1016/0263-8231(95)00037-2

    Article  Google Scholar 

  21. Crisfield MA (1991) Non-linear finite element analysis of solids and structures, vol. 1: essentials. Wiley, Chichester

    MATH  Google Scholar 

  22. Crisfield MA (1996) Non-linear finite element analysis of solids and structures, vol. 2: advanced topics. Wiley, Chichester

    Google Scholar 

  23. Daszkiewicz K, Witkowski W, Burzyński S, Chróścielewski J (2019) Robust four-node elements based on Hu–Washizu principle for nonlinear analysis of Cosserat shells. Continuum Mech Thermodyn 31(6):1757. https://doi.org/10.1007/s00161-019-00767-1

    Article  MathSciNet  Google Scholar 

  24. Dujc J, Brank B (2012) Stress resultant plasticity for shells revisited. Comput Methods Appl Mech Eng 247:146–165. https://doi.org/10.1016/j.cma.2012.07.012

    Article  MathSciNet  MATH  Google Scholar 

  25. Dvorkin EN, Bathe KJ (1984) A continuum mechanics based four-node shell element for general nonlinear analysis. Eng Comput 1(1):77–88. https://doi.org/10.1108/eb023562

    Article  Google Scholar 

  26. Echter R, Oesterle B, Bischoff M (2012) A hierarchic family of isogeometric shell finite elements. Comput Methods Appl Mech Eng 254:170–180. https://doi.org/10.1016/j.cma.2012.10.018

    Article  MathSciNet  MATH  Google Scholar 

  27. Euclidean space, Math - Conversion Matrix to Quaternion. http://www.euclideanspace.com/maths/geometry/rotations/conversions/matrixToQuaternion/. Visited on 21 Aug 2020

  28. Gee M, Ramm E, Wall WA (2005) Parallel multilevel solution of nonlinear shell structures. Comput. Methods Appl Mech Engrg 194:2513–2533. https://doi.org/10.1016/j.cma.2004.07.043

    Article  MATH  Google Scholar 

  29. Goto Y, Watanabe Y, Kasugai T, Obata M (1992) Elastic buckling phenomenon applicable to deployable rings. Int J Solids Struct 29(7):893–909. https://doi.org/10.1016/0020-7683(92)90024-N

    Article  Google Scholar 

  30. Greco L, Cuomo M, Contrafatto L (2018) A reconstructed local B formulation for isogeometric Kirchhoff-Love shells. Comput Methods Appl Mech Engrg 332:462–487. https://doi.org/10.1016/j.cma.2018.01.005

    Article  MathSciNet  MATH  Google Scholar 

  31. Gruttmann F, Wagner W (2005) A linear quadrilateral shell element with fast stiffness computation. Comp Methods Appl Mech Engrg 194:4279–4300. https://doi.org/10.1016/j.cma.2004.11.005

    Article  MATH  Google Scholar 

  32. Gruttmann F, Wagner W (2006) Structural analysis of composite laminates using a mixed hybrid shell element. Comput Mech 37:479–497. https://doi.org/10.1007/s00466-005-0730-1

    Article  MATH  Google Scholar 

  33. Ibrahimbegovic A (1997) On the choice of finite rotation parameters. Comput Methods Appl Mech Eng 149(1–4):49–71. https://doi.org/10.1016/S0045-7825(97)00059-5

    Article  MathSciNet  MATH  Google Scholar 

  34. Ibrahimbegović A (2009) Nonlinear solid mechanics. Springer, Netherlands, Dordrecht

    Book  Google Scholar 

  35. Ibrahimbegović A, Brank B, Courtois P (2001) Stress resultant geometrically exact form of classical shell model and vector-like parameterization of constrained finite rotations. Int J Numer Meth Eng 52(11):1235–1252. https://doi.org/10.1002/nme.247

    Article  MATH  Google Scholar 

  36. Intel MKL Pardiso solver cookbook. Available at: https://software.intel.com/content/www/us/en/develop/documentation/mkl-cookbook/top.html. visited on 27 Aug 2020

  37. Iwicki P, Tejchman J, Chróścielewski J (2014) Dynamic FE simulations of buckling process in thin-walled cylindrical metal silos. Thin-Walled Struct 84:344–359. https://doi.org/10.1016/j.tws.2014.07.011

    Article  Google Scholar 

  38. Klinkel S, Gruttmann F, Wagner W (2007) A mixed shell formulation accounting for thickness strains and finite strain 3d material models. Int J Numer Methods Eng 74:945–970. https://doi.org/10.1002/nme.2199

    Article  MATH  Google Scholar 

  39. Knight NF (1997) Raasch challenge for shell elements. AIAA J 35(2):375–381. https://doi.org/10.2514/2.104

    Article  MATH  Google Scholar 

  40. Ko Y, Lee PS, Bathe KJ (2016) The MITC4 + shell element and its performance. Comput Struct 169:57–68. https://doi.org/10.1016/j.compstruc.2016.03.002

    Article  Google Scholar 

  41. Ko Y, Lee PS, Bathe KJ (2017) A new MITC4 + shell element. Comput Struct 182:404–418. https://doi.org/10.1016/j.compstruc.2016.11.004

    Article  Google Scholar 

  42. Ko Y, Lee PS, Bathe KJ (2017) The MITC4 + shell element in geometric nonlinear analysis. Comput Struct 185:1–14. https://doi.org/10.1016/j.compstruc.2017.01.015

    Article  Google Scholar 

  43. Ko Y, Lee Y, Lee PS, Bathe KJ (2017) Performance of the MITC3 + and MITC4 + shell elements in widely-used benchmark problems. Comput Struct 193:187–206. https://doi.org/10.1016/j.compstruc.2017.08.003

    Article  Google Scholar 

  44. Korelc J (2020) AceGen manual, AceFEM manual. http://symech.fgg.uni-lj.si/. Accessed 18 Jan 2021

  45. Korelc J, Wriggers P (2016) Automation of finite element methods. Springer, Berlin

    Book  Google Scholar 

  46. Kulikov GM, Plotnikova SV (2010) A family of ANS four-node exact geometry shell elements in general convected curvilinear coordinates. Int J Numer Methods Eng 83(10):1376–1406. https://doi.org/10.1002/nme.2872

    Article  MATH  Google Scholar 

  47. Lavrenčič M, Brank B (2018) Simulation of shell buckling by implicit dynamics and numerically dissipative schemes. Thin-Walled Struct 132:682–699. https://doi.org/10.1016/j.tws.2018.08.010

    Article  Google Scholar 

  48. Lavrenčič M, Brank B (2019) Hybrid-mixed shell quadrilateral that allows for large solution steps and is low-sensitive to mesh distortion. Comput Mech. https://doi.org/10.1007/s00466-019-01759-3

    Article  MATH  Google Scholar 

  49. Lavrenčič M, Brank B, Brojan M (2020) Multiple wrinkling mode transitions in axially compressed cylindrical shell-substrate in dynamics. Thin-Walled Struct 150:106700. https://doi.org/10.1016/j.tws.2020.106700

    Article  Google Scholar 

  50. Lee PS, Bathe KJ (2002) On the asymptotic behavior of shell structures and the evaluation in finite element solutions. Comput Struct 80:235–255. https://doi.org/10.1016/s0045-7949(02)00009-3

    Article  Google Scholar 

  51. Macneal RH, Harder RL (1985) A proposed standard set of problems to test finite element accuracy. Finite Elem Anal Des 1(1):3–20. https://doi.org/10.1016/0168-874X(85)90003-4

    Article  Google Scholar 

  52. Magisano D, Leonetti L, Garcea G (2017) Advantages of the mixed format in geometrically nonlinear analysis of beams and shells using solid finite elements. Int J Numer Methods Eng 109:1237–1262. https://doi.org/10.1002/nme.5322

    Article  MathSciNet  Google Scholar 

  53. Mäkinen J (2008) Rotation manifold SO(3) and its tangential vectors. Comput Mech 42(6):907–919. https://doi.org/10.1007/s00466-008-0293-z

    Article  MathSciNet  MATH  Google Scholar 

  54. McAuliffe C, Waisman H (2014) A Pian-Sumihara type element for modeling shear bands at finite deformation. Comput Mech 53:925–940. https://doi.org/10.1007/s00466-013-0940-x

    Article  MathSciNet  MATH  Google Scholar 

  55. Nguyen CU, Ibrahimbegovic A (2020) Hybrid-stress triangular finite element with enhanced performance for statics and dynamics. Comput Methods Appl Mech Eng (In editorial process)

  56. Oesterle B, Sachse R, Ramm E, Bischoff M (2017) Hierarchic isogeometric large rotation shell elements including linearized transverse shear parametrization. Comput Methods Appl Mech Eng 321:383–405. https://doi.org/10.1016/j.cma.2017.03.031

    Article  MathSciNet  MATH  Google Scholar 

  57. Pian THH, Sumihara K (1984) Rational approach for assumed stress finite elements. Int J Numer Methods Eng 20(9):1685–1695. https://doi.org/10.1002/nme.1620200911

    Article  MATH  Google Scholar 

  58. Polat C (2010) A parametric study for four node bilinear EAS shell elements. J Mech 26(04):431–438. https://doi.org/10.1017/S1727719100004639

    Article  Google Scholar 

  59. Porenta L, Brank B, Dujc J, Brojan M, Tušek J (2020) A shell finite element model for superelasticity of shape memory alloys. In: Altenbach H, Chinchaladze N, Kienzler R, Müller W (eds) Analysis of shells, plates, and beams. Advanced structured materials, vol 134. Springer, Cham. https://doi.org/10.1007/978-3-030-47491-1_20

    Chapter  MATH  Google Scholar 

  60. Rafiee R, Moghadam RM (2014) On the modeling of carbon nanotubes: a critical review. Compos Part B Eng 56:435–449. https://doi.org/10.1016/j.compositesb.2013.08.037

    Article  Google Scholar 

  61. Simo JC, Armero F (1992) Geometrically non-linear enhanced strain mixed methods and the method of incompatible modes. Int J Numer Methods Eng 33(7):1413–1449. https://doi.org/10.1002/nme.1620330705

    Article  MATH  Google Scholar 

  62. Simo JC, Fox DD (1989) On a stress resultant geometrically exact shell model. Part I: formulation and optimal parametrization. Comput Methods Appl Mech Eng 72:267–304. https://doi.org/10.1016/0045-7825(89)90002-9

    Article  MATH  Google Scholar 

  63. Simo JC, Fox DD, Rifai MS (1989) On a stress resultant geometrically exact shell model. Part II: the linear theory; computational aspects. Comput Methods Appl Mech Eng 73:53–92. https://doi.org/10.1016/0045-7825(89)90098-4

    Article  MATH  Google Scholar 

  64. Simo JC, Fox DD, Rifai MS (1990) On a stress resultant geometrically exact shell model. Part III: computational aspects of the nonlinear theory. Comput Methods Appl Mech Eng 79:21–70. https://doi.org/10.1016/0045-7825(90)90094-3

    Article  MATH  Google Scholar 

  65. Simo JC, Hughes TJR (1986) On the variational foundations of assumed strain methods. J Appl Mech 53(1):51–54. https://doi.org/10.1115/1.3171737

    Article  MathSciNet  MATH  Google Scholar 

  66. Simo JC, Rifai MS (1990) A class of mixed assumed strain methods and the method of incompatible modes. Int J Numer Methods Eng 29:1595–1638. https://doi.org/10.1002/nme.1620290802

    Article  MathSciNet  MATH  Google Scholar 

  67. Simo JC, Rifai MS, Fox DD (1990) On a stress resultant geometrically exact shell model. Part IV: variable thickness shells with through-the-thickness stretching. Comput Methods Appl Mech Eng 81:91–126. https://doi.org/10.1016/0045-7825(90)90143-A

    Article  MATH  Google Scholar 

  68. Stanić A, Brank B, Korelc J (2016) On path following methods for structural failure problems. Comput Mech 58:281–306. https://doi.org/10.1007/s00466-016-1294-y

    Article  MathSciNet  MATH  Google Scholar 

  69. Sze KY, Liu XH, Lo SH (2004) Popular benchmark problems for geometric nonlinear analysis of shells. Finite Elem Anal Des 40:1551–1569. https://doi.org/10.1016/j.finel.2003.11.001

    Article  Google Scholar 

  70. Veldin T, Lavrenčič M, Brank B, Brojan M (2020) A comparison of computational models for wrinkling of pressurized core-shell systems (In editorial process)

  71. Veldin T, Brank B, Brojan M (2019) Computational finite element model for surface wrinkling of shells on soft substrates. Commun Nonlinear Sci Numer Simul 79(104863):1–117. https://doi.org/10.1016/j.cnsns.2019.104863

    Article  MathSciNet  MATH  Google Scholar 

  72. Vu-Quoc L, Tan XG (2003) Optimal solid shells for nonlinear analyses of multilayer composites. I: statics. Comput Methods Appl Mech Eng 192(9–10):975–1016. https://doi.org/10.1016/S0045-7825(02)00435-8

    Article  MATH  Google Scholar 

  73. Wagner W, Gruttmann F (2005) A robust non-linear mixed hybrid quadrilateral shell element. Int J Numer Methods Eng 64:635–666. https://doi.org/10.1002/nme.1387

    Article  MATH  Google Scholar 

  74. Wagner W, Gruttmann F (2020) An improved quadrilateral shell element based on the Hu–Washizu functional. Adv Model Simul Eng Sci 7:28. https://doi.org/10.1186/s40323-020-00162-5

    Article  Google Scholar 

  75. Washizu K (1982) Variational methods in elasticity and plasticity, 3rd edn. Pergamon Press, Oxford

    MATH  Google Scholar 

  76. Wiśniewski K (2010) Finite rotation shells, basic equations and finite elements for Reissner kinematics. Springer Netherlands, Dordrecht

    MATH  Google Scholar 

  77. Wisniewski K, Turska E (2008) Improved four-node Hellinger-Reissner elements based on skew coordinates. Int J Numer Methods Eng 76:798–836. https://doi.org/10.1002/nme.2343

    Article  MathSciNet  MATH  Google Scholar 

  78. Wisniewski K, Turska E (2009) Improved 4-node Hu–Washizu elements based on skew coordinates. Comput Struct 87:407–424. https://doi.org/10.1016/j.compstruc.2009.01.011

    Article  Google Scholar 

  79. Wisniewśki K, Turska E (2017) Selected topics on mixed/enhanced four-node shell elements with drilling rotation. In: Altenbach H, Eremeyev V (eds) Shell-like structures advanced theories and applications. Springer Nature, Switzerland

    Google Scholar 

  80. Wisniewski K, Wagner W, Turska E, Gruttmann F (2010) Four-node Hu–Washizu elements based on skew coordinates and contravariant assumed strain. Comput Struct 88:1278–1284. https://doi.org/10.1016/j.compstruc.2010.07.008

    Article  Google Scholar 

  81. Wolfram Research Inc. (2019) Mathematica Version 11.3. Champaign, IL

  82. Wriggers P (2008) Nonlinear finite element methods. Springer, New York

    MATH  Google Scholar 

  83. Yu G, Xie X, Carstensen C (2011) Uniform convergence and a posteriori error estimation for assumed stress hybrid finite element methods. Comput Methods Appl Mech Eng 200:2421–2433. https://doi.org/10.1016/j.cma.2011.03.018

    Article  MathSciNet  MATH  Google Scholar 

  84. Yu G, Xie X, Carstensen C (2011) Uniform convergence and a posteriori error estimation for assumed stress hybrid finite element methods. Comput Methods Appl Mech Eng 200(29–32):2421–2433. https://doi.org/10.1016/j.cma.2011.03.018

    Article  MathSciNet  MATH  Google Scholar 

  85. Yuan KY, Huang YS, Pian THH (1993) New strategy for assumed stresses for 4-node hybrid stress membrane element. Int J Numer Methods Eng 36:1747–1763. https://doi.org/10.1002/nme.1620361009

    Article  MATH  Google Scholar 

  86. Zupan E, Saje M, Zupan D (2009) The quaternion-based three-dimensional beam theory. Comput Methods Appl Mech 198(49–52):3944–3956. https://doi.org/10.1016/j.cma.2009.09.002

    Article  MathSciNet  MATH  Google Scholar 

Download references

Funding

This study was funded by the Slovenian Research Agency (Grant Number J2-1722).

Author information

Authors and Affiliations

  1. Faculty of Civil and Geodetic Engineering, University of Ljubljana, Jamova c. 2, 1000, Ljubljana, Slovenia

    Marko Lavrenčič & Boštjan Brank

Authors
  1. Marko Lavrenčič
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Boštjan Brank
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Boštjan Brank.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Lavrenčič, M., Brank, B. Hybrid-Mixed Low-Order Finite Elements for Geometrically Exact Shell Models: Overview and Comparison. Arch Computat Methods Eng 28, 3917–3951 (2021). https://doi.org/10.1007/s11831-021-09537-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11831-021-09537-2

Search

Navigation