Skip to main content
SpringerLink
Log in

New Results on the Study of Z q -Equivariant Planar Polynomial Vector Fields

  • Published:
Qualitative Theory of Dynamical Systems Aims and scope Submit manuscript

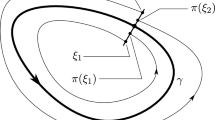
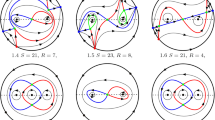
Abstract

In this paper, we introduce some new results on the study of Z q -equivariant planar polynomial vector fields. The main conclusions are as follows. (1) For the Z 2-equivariant planar cubic systems having two elementary foci, the characterization of a bi-center problem and shortened expressions of the first six Lyapunov constants are completely solved. The necessary and sufficient conditions for the existence of the bi-center are obtained. All possible first integrals are given. Under small Z 2-equivariant cubic perturbations, the conclusion that there exist at most 12 small-amplitude limit cycles with the scheme \({\langle 6\amalg 6\rangle}\) is proved. (2) On the basis of mentioned work in (1), by considering the bifurcation of a global limit cycle from infinity, we show that under small Z 2-equivariant cubic perturbations, such bi-center cubic system has at least 13 limit cycles with the scheme \({\langle 1 \langle 6\amalg 6\rangle\rangle}\), i.e., we obtain that the Hilbert number H(3) ≥ 13. (3) For the Z 5-equivariant planar polynomial vector field of degree 5, we shown that such system has at least five symmetric centers if and only if it is a Hamltonian vector field. The characterization of a center problem is completely solved. The shortened expressions of the first four Lyapunov constants are given. Under small Z 5-equivariant perturbations, the conclusion that perturbed system has at least 25 limit cycles with the scheme \({\langle 5\amalg 5\amalg 5\amalg 5\amalg 5\rangle}\) is rigorously proved. (4) For the Z 6-equivariant planar polynomial vector field of degree 5, we proved that such system has at least six symmetric centers if and only if it is a Hamltonian vector field. The characterization of a center problem is completely solved. The shortened expressions of the first four Lyapunov constants are given. Under small Z 6-equivariant perturbations, the conclusion that perturbed system has at least 24 limit cycles with the scheme \({\langle 4\amalg 4\amalg 4\amalg 4\amalg 4\amalg4\rangle}\) is rigorously proved. Two schemes of distributions of limit cycles are given.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Devlin J., Lloyd N.G., Pearson J.M.: Cubic systems and Abel equations. J. Differ. Eqn. 147, 435–454 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  2. Gasull A., Guillamon A., Manosa V.: A explicit expression of the first Liapunov and period constants with application. J. Math. Anal. Appl. 211, 190–212 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  3. Gasull A., Torregrosa J.: Center problem for several differential equations via Cherkas’ method. J. Math. Anal. Appl. 228, 322–343 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  4. Li J.: Hilbert’s 16th problem and bifurcations of planar polynomial vector fields. Int. J. Bifurc. Chaos 13(1), 47–106 (2003)

    Article  MATH  Google Scholar 

  5. Li J., Zhao X.: Rotation symmetry groups of planar Hamiltonian systems. Ann. Differ. Equ. 5, 25–33 (1989)

    MATH  Google Scholar 

  6. Li J., Lin Y.: Global bifurcations in a perturbed cubic system with Z 2-symmetry. Acta Math. Appl. Sin. (English Ser.) 8(2), 131–143 (1992)

    Article  MATH  MathSciNet  Google Scholar 

  7. Li J., Chan H.S.Y., Chung K.W.: Bifurcations of limit cycles in a Z 6-equivariant planar vector field of degree 5. Sci. China Ser. A 45(7), 817–826 (2002)

    MATH  MathSciNet  Google Scholar 

  8. Li J., Chan H.S.Y., Chung K.W.: Investigations of bifurcations of limit cycles in a Z 2-equivariant planar vector field of degree 5. Int. J. Bifurc. Chaos 12(10), 2137–2157 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  9. Li J., Zhang M.: Bifurcations of limit cycles of Z 8-equivariant planar vector field of degree 7. J. Dyn. Differ. Equ. 16(4), 1123–1139 (2004)

    Article  MATH  Google Scholar 

  10. Li J., Zhou H.: On the control of parameters of distributions of limit cycles for a Z 2-equivariant perturbed planar Hamiltonian polynomial vector field. Int. J. Bifurc. Chaos 15(1), 137–155 (2004)

    Google Scholar 

  11. Li J., Zhang M.: Bifurcations of limit cycles in a Z 2-equivariant planar polynomial vector field of degree 7. Int. J. Bifurc. Chaos 16, 925–943 (2006)

    Article  MATH  Google Scholar 

  12. Li J., Huang Q.: Bifurcations of limit cycles forming compound eyes in the cubic system. Chin. Ann. Math. 8B, 391–403 (1987)

    Google Scholar 

  13. Liu Y.: Theory of center-focus in a class of high order singular points and infinity. Sci China 31(1), 37–48 (2001)

    Google Scholar 

  14. Liu Y., Chen H.B.: Formulas of singular point quantities and the first 10 saddle quantities of a class of cubic system. Acta Math. Appl. Sin. (Chin. Ser.) 25(2), 295–302 (2002)

    MATH  Google Scholar 

  15. Liu Y., Huang W.: A cubic system with twelve small amplitude limit cycles. Bull. Sci. Math. 129, 83–98 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  16. Liu Y., Li J.: Center problem and multiple Hopf bifurcation for the Z 6-equivariant planar polynomial vector fields of degree 5. Int. J. Bifurc. Chaos 19, 1741–1749 (2009)

    Article  MATH  Google Scholar 

  17. Liu Y., Li J., Huang W.: Singular Point Values, Center Problem and Bifurcations of Limit Cycles of Two Dimensional Differential Autonomous Systrms. Science Prees, Beijing (2008)

    Google Scholar 

  18. Romanovski V.G., Marko R.: The centre and isochronicity problems for some cubic system. J. Phys. A Math. Gen. 34, 10267–10292 (2001)

    Article  MATH  Google Scholar 

  19. Sadovskii A.P.: Centers and foci for a class of cubic systems. Differ. Eqn. 36(12), 1652–1657 (2000)

    MathSciNet  Google Scholar 

  20. Schlomiuk D., Guckenheimer J., Rand R.: Integrability of plane quadratic vector fields. Expo. Math. 8, 3–25 (1990)

    MATH  MathSciNet  Google Scholar 

  21. Sibirskii K.S.: The number of limit cycles in the neighborhood of a singular point. Differ. Eqn. 1, 36–47 (1965)

    Google Scholar 

  22. Sibirskii, K.S.: Algebraic Invariants of Diffrential Equations and Matrices. Shtiinsta, Kishinev (1976) (in Russian)

  23. Yu P., Han M.A.: Twelve limit cycles in 3rd-planar system with Z 2 symmetry. Commun. Appl. Pure Anal. 3, 515–526 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  24. Yu P., Han M.A.: Small limit cycles bifurcating from fine focus points in cubic order Z 2-equivariant vector fields. Chaos Solitons Fractals 24, 329–348 (2005)

    MATH  MathSciNet  Google Scholar 

  25. Yu P., Han M.: Twelve limit cycles in a cubic case of the 16th Hilbert problem. Int. J. Bifurc. Chaos 15, 2192–2205 (2005)

    MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Zhejiang Normal University, Jinhua, 321004, Zhejiang, People’s Republic of China

    Jibin Li & Yirong Liu

  2. School of Science, Kunming University of Science and Technology, Kunming, 650093, Yunnan, People’s Republic of China

    Jibin Li

Authors
  1. Jibin Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yirong Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jibin Li.

Additional information

This research was partially supported by the National Natural Science Foundation of China (10671179 and 10771196).

Rights and permissions

Reprints and permissions

About this article

Cite this article

Li, J., Liu, Y. New Results on the Study of Z q -Equivariant Planar Polynomial Vector Fields. Qual. Theory Dyn. Syst. 9, 167–219 (2010). https://doi.org/10.1007/s12346-010-0024-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12346-010-0024-7

Keywords

Mathematics Subject Classification (2000)

Search

Navigation