Damped vibration analysis of cracked Timoshenko beams with restrained end conditions

Abstract

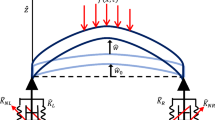
Damped vibration of a cracked Timoshenko beam with ends supported with damper, linear and rotational springs is investigated. Frequencies in complex forms have been obtained for both cracked Euler–Bernoulli and Timoshenko beams. Depending upon the crack-depth and crack-location, frequencies have been tabulated in each case. The results have also been compared in terms of the ratio of the beam depth to the beam length. Modal shapes for various conditions have also been plotted.

Appendix

The first of Eq. (25) is

$$\begin{aligned} {{{{\varPsi _{1} }'}}}(\xi ) &= {{{\overline{m}}}_{1}}{{C}_{1}}\sin {{\beta }_{1}}\xi +{{C}_{2}}{{{\overline{m}}}_{1}}\cos {{\beta }_{1}}\xi +{{C}_{3}}{{{\overline{m}}}_{2}}\sinh {{\beta }_{2}}\xi \\& \quad+\,{{C}_{4}}{{{\overline{m}}}_{2}}\cosh {{\beta }_{2}}\xi \, . \end{aligned}$$

Integration and derivation of $\varPsi _{1}'$ in terms of $\xi$ yields

$$\begin{aligned} \varPsi _{1}(\xi ) &= -{{m}_{1}}{{C}_{1}}\cos {{\beta }_{1}}\xi +{{m}_{1}}{{C}_{2}}\sin {{\beta }_{1}}\xi +{{m}_{2}}{{C}_{3}}\cosh {{\beta }_{2}}\xi \\& \quad+\,{{m}_{2}}{{C}_{4}}\sinh {{\beta }_{2}}\xi +C_5 \, ,\\ \varPsi _{1}''(\xi ) &= \left( \overline{m}_1 C_1\beta _{1}\cos {\beta _{1}\xi }-\overline{m}_1 C_2\beta _{1}\sin {\beta _{1}\xi }\right. \\& \quad\left. +\overline{m}_2 C_3\beta _{2}\cosh {\beta _{2}\xi }+\overline{m}_2 C_4\beta _{2}\sinh {\beta _{2}\xi }\right) \, . \end{aligned}$$

The first of Eq. (23) is

$$\begin{aligned} {{Y}_{1}}(\xi )={{C}_{1}}\sin {{\beta }_{1}}\xi +{{C}_{2}}\cos {{\beta }_{1}}\xi +{{C}_{3}}\sinh {{\beta }_{2}}\xi +{{C}_{4}}\cosh {{\beta }_{2}}\xi \, . \end{aligned}$$

Differentiating ${{Y}_{1}}(\xi )$ with respect to $\xi$ gives

$$\begin{aligned} Y_1'(\xi ) &= C_1 \beta _{1}\cos {\beta _{1}\xi }-C_2 \beta _{1}\sin {\beta _{1}\xi }+C_3 \beta _{2}\cosh {\beta _{2}\xi }\\& \quad+\,C_4 \beta _{2}\sinh {\beta _{2}\xi }=0 \, . \end{aligned}$$

Inserting these expressions into the second of Eq. (19) yields

$$\begin{aligned}&s^2\left( \overline{m}_1 C_1\beta _{1}\cos {\beta _{1}\xi }-\overline{m}_1 C_2\beta _{1}\sin {\beta _{1}\xi }+\overline{m}_2 C_3\beta _{2}\cosh {\beta _{2}\xi }\right. \\&\quad \left. +\,\overline{m}_2 C_4\beta _{2}\sinh {\beta _{2}\xi }\right) -\left( 1-\lambda ^2 r^2 s^2\right) \left( -{{m}_{1}}{{C}_{1}}\cos {{\beta }_{1}}\xi \right. \\&\quad \left. +\,{{m}_{1}}{{C}_{2}}\sin {{\beta }_{1}}\xi +{{m}_{2}}{{C}_{3}}\cosh {{\beta }_{2}}\xi +{{m}_{2}}{{C}_{4}}\sinh {{\beta }_{2}}\xi +C_5\right) \\&\quad +\,C_1 \beta _{1}\cos {\beta _{1}\xi }-C_2 \beta _{1}\sin {\beta _{1}\xi }+C_3 \beta _{2}\cosh {\beta _{2}\xi }\\&\quad +\,C_4 \beta _{2}\sinh {\beta _{2}\xi }=0 \, , \end{aligned}$$

where $C_5$ is a constant. In order that the equality is satisfied, $C_5$ must surely be zero since the all remaining terms involve trigonometric or hyperbolic functions.