Introduction

This paper is devoted to the study of the propagation of elastic waves along the axisymmetric cylindrical cavity with only one interface. The directional propagation of elastic waves in extended bodies of various profiles has been studied for a long time, but the researchers of elastic waveguides were initially attracted not by the shell, but by bodies with one surface. In 1876, Pochgammer obtained the dispersion equation for waves in an extended circular cross section, and in 1885 J.V. Strett (Lord Rayleigh) described an elastic wave propagating along the flat interface between an elastic half-space and a vacuum, later named after him. Thus, historically the first to be considered simple cross-sectional shapes—a line and a circle corresponding to an elastic half-space with a flat boundary and an extended rod in a vacuum. Wave propagation along the border was discovered.

A feature of the Rayleigh wave is its localization near flat surface half-space. In the absence of losses, Rayleigh waves are continuous, without dispersion waves (their phase velocity along the surface does not depend on frequency) and exist in the entire frequency range in which the elastic medium filling the half-space can be considered uniform and isotropic. The oscillation equations of an elastic rod with a circular cross section were presented to the world in the classical article by Pochgammer 1876, he also analyzed extreme cases of low (ω → 0) and high (ω → ∞) frequencies [1]. Since then, many effects have been devoted to these effects, wave characteristics have been studied in more complex structures [2], including shells, and attacks in systems filled with anisotropic media [3]. Separately, it should be noted the work of V.V. Krylov, for example [4], is devoted to surface acoustic waves in bodies of complex topology, including such elastic waveguides as the surfaces of grooves and rods of various profiles. The study of cylindrical waveguides is hampered by the presence of special functions in the resulting dispersion equations. A detailed study of their mode structure, therefore, began only from the 1950s–1960s, when it became possible to solve transcendental equations numerically on a computer [5, 6]. Interest even to the simplest systems with axial symmetry has not weakened so far, as evidenced, for example, in [7, 8], where when considering a rather complicated case of so-called helical waves on cylindrical surfaces, the authors needed a powerful numerical simulation tool using cumbersome software packages.

In this paper, we consider an important special case of axisymmetric eigen (normal) waves localized near the surface of a cylindrical cavity filled with vacuum in an infinite elastic medium. The fact of propagation without attenuation of waves over the surface of a cylindrical cavity is overlooked in well-known books [9, 10] and in large review articles [11, 12], and articles [13, 14] and a number of others create the impression that surface waves in such a system is fundamentally damped. In this case, only nonaxisymmetric waves traveling along the surface of the cavity across the generator are considered. Such components are present, obviously, in the above-mentioned helical waves, in spite of the decaying character, sometimes called the highest modes of the cylindrical cavity in the literature [15]. Further, we will speak only of a propagating mode, a brief description of which on the cavity is given only in the book [16] with reference to the article [15], where it was studied experimentally, but the cut-off effect is described only qualitatively, and the equations are given without output.

Thus, the study of axisymmetric elastic waves in multilayer cylindrical systems with the highest possible the use of analytical methods is relevant for the development of a new generation of well sounding techniques.

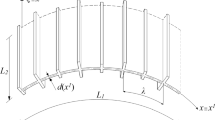
Statement of the Problem and Method of Solution

The basic equations of motion of a viscoelastic medium, having cylindrical cavities with radius R, take the form in displacements

$$\tilde{\mu }\nabla^{2} \vec{u} + (\tilde{\lambda } + \tilde{\mu }){\text{grad}}\,{\text{div}}\,\vec{u} = \rho \frac{{\partial^{2} \vec{u}}}{{\partial t^{2} }},$$
(1)

where

$$\begin{aligned} & \tilde{\lambda }\phi \left( t \right) = \lambda_{01} \left[ {\varphi \left( t \right) - \int\limits_{0}^{t} {R_{\lambda } \left( {t - \tau } \right)\phi \left( t \right){\text{d}}\tau } } \right]; \\ & \tilde{\mu }\varphi \left( t \right) = \mu_{01} \left[ {\varphi \left( t \right) - \int\limits_{0}^{t} {R_{\mu } \left( {t - \tau } \right)\varphi \left( t \right){\text{d}}\tau } } \right]. \\ \end{aligned}$$
(2)

\(\overrightarrow {u} (u_{r} ,u_{\theta } ,u_{z} )\)—displacement vector, \(\rho\)—material density,\(\sigma_{ik}\)—stress tensor, \(\hat{\varepsilon }_{ik}\)—tensor strain, \(\theta\)—volumetric deformation, \(\tilde{\lambda }\) and \(\tilde{\mu }\)—operator module of elasticity [17, 18], \(\varphi \left( t \right)\)—arbitrary function of time; \(R_{\lambda } \left( {t - \tau } \right)\) and \(R_{\mu } \left( {t - \tau } \right)\)—relaxation cores and \(\lambda_{01} ,\mu_{01}\)—instantaneous modulus of elasticity.

We accept the integral terms in (2) small, then the functions \(\varphi \left( t \right) = \psi \left( t \right)e^{{ - i\omega_{R} t}}\), where \(\psi \left( t \right)\)—slowly changing function of time, \(\omega_{R}\)—real constant.

Next, applying the freezing procedure [19], we note the relations (2) by approximate

$$\begin{aligned} \bar{\lambda}\varphi& = \lambda_{01} \left[{1 - \varGamma_{\lambda}^{C} \left({\omega_{R}} \right) - i\varGamma_{\lambda}^{S} \left({\omega_{R}} \right)} \right];\quad\\ \bar{\mu}\varphi &= \mu_{01} \left[{1 - \varGamma_{\mu}^{C} \left({\omega_{R}} \right) - i\varGamma_{\mu}^{S} \left({\omega_{R}} \right)} \right]\varphi, \end{aligned}$$

where

$$\begin{aligned} \varGamma_{\lambda }^{C} \left( {\omega_{R} } \right) = \int\limits_{0}^{\infty } {R_{\lambda } \left( \tau \right)\cos \omega_{R} \tau \,{\text{d}}\tau ;} \quad \varGamma_{\lambda }^{S} \left( {\omega_{R} } \right) = \int\limits_{0}^{\infty } {R_{\lambda } \left( \tau \right)\sin \omega_{R} \tau \,{\text{d}}\tau } , \hfill \\ \varGamma_{\mu }^{C} \left( {\omega_{R} } \right) = \int\limits_{0}^{\infty } {R_{\mu } \left( \tau \right)\cos \omega_{R} \tau \,{\text{d}}\tau } ,\quad \varGamma_{\mu }^{S} \left( {\omega_{R} } \right) = \int\limits_{0}^{\infty } {R_{\mu } \left( \tau \right)\sin \omega_{R} \tau \,{\text{d}}\tau } , \hfill \\ \end{aligned}$$

—the cosine and sine Fourier images of the relaxation core of the material, respectively. As an example of a viscoelastic material we take three parametric relaxation core \(R_{\lambda } \left( t \right) = R_{\mu } \left( t \right) = Ae^{ - \beta \,t} /t^{1 - \alpha } .\) Influence function \(R\left( {t - \tau } \right)\) the usual requirements of integrability, continuity (except for \(t = \tau\)), sign certainty, and monotony:

$$R\,\,\rangle \,\,0,\,\,\,\,\frac{{{\text{d}}R(t)}}{{{\text{d}}t}} \le 0\,,\,\,0\langle \,\int\limits_{0}^{\infty } {R(t){\text{d}}t\langle 1} .$$

The relationship between stresses and strains is as follows

$$\hat{\varepsilon }_{ik} = \frac{1}{2}\left( {\frac{{\partial u_{i} }}{{\partial x_{k} }} + \frac{{\partial u_{k} }}{{\partial x_{i} }}} \right),\quad \sigma_{ik} = \,\tilde{\lambda }\theta \delta_{ik} + 2\tilde{\mu }\hat{\varepsilon }_{ik}$$
(3)

We set the boundary conditions in the form:

$$r = R;\quad \sigma_{rr} = 0;\quad \sigma_{r\theta } = 0;\quad \sigma_{rz} = 0.$$
(4)

Corresponding motion of the surface of the cylindrical cavity satisfies the functions of longitudinal and transverse potentials:

$$\begin{aligned} & u_{r} = \frac{\partial \varphi }{\partial r} + \frac{1}{r}\frac{{\partial \psi_{z} }}{\partial \theta } - \frac{{\partial \psi_{\theta } }}{\partial z}; \\ & u_{\theta } = \frac{1}{r}\frac{\partial \varphi }{\partial \theta } + \frac{{\partial \psi_{r} }}{\partial z} - \frac{{\partial \psi_{z} }}{\partial r}; \\ & u_{z} = \frac{\partial \varphi }{\partial z} + \frac{{\partial \psi_{\theta } }}{\partial r} + \frac{{\psi_{\theta } }}{r} - \frac{1}{r}\frac{{\partial \psi_{r} }}{\partial \theta } \\ & \nabla^{2} \varphi - \frac{1}{{\bar{c}_{p}^{2} }}\frac{{\partial^{2} \varphi }}{{\partial t^{2} }} = 0; \\ & \nabla^{2} \psi_{Z} - \frac{1}{{\bar{c}_{s}^{2} }}\frac{{\partial^{2} \psi_{Z} }}{{\partial t^{2} }} = 0; \\ & \nabla^{2} \psi_{\theta } - \frac{{\psi_{\theta } }}{{r^{2} }} + \frac{2}{{r^{2} }}\frac{{\partial \psi_{r} }}{\partial \theta } - \frac{1}{{\bar{c}_{s}^{2} }}\frac{{\partial^{2} \psi_{\theta } }}{{\partial t^{2} }} = 0; \\ & \nabla^{2} \psi_{r} - \frac{{\psi_{r} }}{{r^{2} }} - \frac{2}{{r^{2} }}\frac{{\partial \psi_{\theta } }}{\partial \theta } - \frac{1}{{\bar{c}_{s}^{2} }}\frac{{\partial^{2} \psi_{r} }}{{\partial t^{2} }} = 0. \\ \end{aligned}$$
(5)

Here \(\bar{c}_{s}^{2} = c_{s}^{2} \varGamma;\bar{c}_{p}^{2} = c^{2} \varGamma .\)

The solution of Eq. (5) is sought in the form

$$\left. \begin{aligned} \varphi_{{}} \left( {r,\theta ,z,t} \right) = \sum\limits_{n = 0}^{\infty } {\varphi_{n} } \left( {\alpha r} \right)\left\{ \begin{aligned} \cos \,n\theta \hfill \\ - \sin \,n\theta \hfill \\ \end{aligned} \right\}e^{{ \pm i\gamma_{p} z}} e^{ - i\omega t} ; \hfill \\ \psi_{r} \left( {r,\theta ,z,t} \right) = \sum\limits_{n = 0}^{\infty } {\psi_{nr} \left( {\beta r} \right)\left\{ \begin{aligned} \sin \,n\theta \hfill \\ - \cos \,n\theta \hfill \\ \end{aligned} \right\}e^{{ \pm i\gamma_{p} z}} e^{ - i\omega t} ;} \hfill \\ \psi_{\theta } \left( {r,\theta ,z,t} \right) = \sum\limits_{n = 0}^{\infty } {\psi_{n\theta } \left( {\beta r} \right)\left\{ \begin{aligned} \cos \,n\theta \hfill \\ - \sin \,n\theta \hfill \\ \end{aligned} \right\}e^{{ \pm i\gamma_{p} z}} e^{ - i\omega t} ;} \hfill \\ \psi_{z} \left( {r,\theta ,z,t} \right) = \sum\limits_{n = 0}^{\infty } {\psi_{nz} \left( {\beta r} \right)\left\{ \begin{aligned} \sin \,n\theta \hfill \\ \cos \,n\theta \hfill \\ \end{aligned} \right\}e^{{ \pm i\gamma_{p} z}} e^{ - i\omega t} ;} \hfill \\ \end{aligned} \right\}$$
(6)

where \(n\)—integer; \(\gamma_{p}\)—dimensionless propagation constant; \(\omega\)—complex frequency; \(r = \frac{{r_{1} }}{{a_{0} }},z = \frac{{z_{1} }}{{a_{0} }}.\) Substituting (6) into (5), we obtain the following ordinary differential equations:

$$\begin{aligned} & \frac{{{\text{d}}^{2} \varphi_{n} }}{{{\text{d}}r^{2} }} + \frac{1}{r}\frac{{{\text{d}}\varphi_{n} }}{{{\text{d}}r}} + \left( {\alpha_{{}}^{2} - \frac{{n^{2} }}{{r^{2} }}} \right)\varphi_{n} = 0; \\ & \frac{{{\text{d}}^{2} \psi_{zn} }}{{{\text{d}}r^{2} }} + \frac{1}{r}\frac{{{\text{d}}\psi_{zn} }}{{{\text{d}}r}} + \left( {\beta_{{}}^{2} - \frac{{n^{2} }}{{r^{2} }}} \right)\psi_{zn} = 0; \\ & \frac{{d^{2} \psi_{\theta n} }}{{dr^{2} }} + \frac{1}{r}\frac{{d\psi_{\theta n} }}{dr} + \frac{1}{{r^{2} }}\left( { - n^{2} \psi_{\theta n} + 2n\psi_{\theta n} - \psi_{\theta n} } \right) + \beta^{2} \psi_{\theta n} = 0; \\ & \frac{{d^{2} \psi_{rn} }}{{dr^{2} }} + \frac{1}{r}\frac{{d\psi_{rn} }}{dr} + \frac{1}{{r^{2} }}\left( { - n^{2} \psi_{rn} + 2n\psi_{\theta n} - \psi_{rn} } \right) + \beta^{2} \psi_{rn} = 0; \\ \end{aligned}$$
(7)

Labeled here: \(\alpha_{{}}^{2} = \frac{{\bar{\varOmega }_{{}}^{2} }}{{\gamma_{{}}^{2} }} - \gamma_{p}^{2} \,;\quad \beta_{{}}^{2} = \bar{\varOmega }_{{}}^{2} - \gamma_{p}^{2} \,;\quad \bar{\varOmega }_{{}} = \frac{{\omega a_{{}} }}{{\bar{c}_{s} }}\,;\quad \gamma_{{}}^{2} = \frac{{2\left( {1 - \nu_{{}} } \right)}}{{1 - 2\nu_{{}} }}.\)

At infinity r → ∞ the potentials of the longitudinal and transverse waves at satisfy the condition of radiation Summerfield [18]:

$$\mathop {\lim }\limits_{r \to \infty } \varphi_{n} = 0,\quad \mathop {\lim }\limits_{r \to \infty } \left( {\sqrt r } \right)\left( {\frac{{\partial \varphi_{n} }}{\partial r} + i\alpha_{{}} \varphi_{n} } \right) = 0,$$
$$\mathop {\lim }\limits_{r \to \infty } \psi_{zn} = 0,\quad \mathop {\lim }\limits_{r \to \infty } \left( {\sqrt r } \right)\left( {\frac{{\partial \psi_{zn} }}{\partial r} + i\beta \psi_{zn} } \right) = 0.$$

The first two equations in (7) obviously have the following solutions for the case of a cylindrical cavity:

$$\begin{aligned} & \varphi_{n} \left( r \right) = F_{n}^{{}} H_{n}^{\left( 1 \right)} \left( {\alpha r} \right), \\ & \psi_{zn} \left( r \right) = M_{1n}^{{}} H_{n}^{\left( 1 \right)} \left( {\beta r} \right) \\ \end{aligned}$$

here \(H_{n}^{\left( 1 \right)} \left( {\beta_{k} r} \right)\)—Henkel function of the nth kind of the 1st order.

To construct solutions of the two remaining equations in (7), it is advisable to consider their sum and difference. This results in two equations whose solutions are expressed in terms of different order Henkel functions

$$\begin{aligned} & \psi_{rn} \left( r \right) = D_{1n}^{{}} H_{n - 1}^{\left( 1 \right)} \left( {\beta r} \right) + D_{2n}^{{}} H_{n + 1}^{\left( 2 \right)} \left( {\beta r} \right), \\ & \psi_{\theta n} \left( r \right) = D_{1n} H_{n - 1}^{\left( 1 \right)} \left( {\beta r} \right) - D_{2n}^{{}} H_{n + 1}^{\left( 2 \right)} \left( {\beta r} \right), \\ \end{aligned}$$

Henkel function of the nth kind of the 1st order. To construct solutions of the two remaining equations in (7), it is advisable to consider their sum and difference. This results in two equations whose solutions are expressed in terms of different order Henkel functions: \(D_{1n} = 0,\) then \(\psi_{rn} = - \psi_{\theta n} .\). This is convenient from the point of view of the subsequent satisfaction of the boundary conditions on the cylindrical surface. Thus, the components of the displacement vector in the vicinity of the cylindrical cavity are represented as

$$\begin{aligned} u_{r} &= \sum\limits_{n = 0}^{\infty } {\left[ {F_{n} \frac{{{\text{d}}H_{n}^{(1)} (\alpha r)}}{{{\text{d}}r}} + D_{2n} i\bar{\gamma }H_{n + 1}^{(1)} (\beta r) + M_{1n} nH_{n}^{(1)} (\beta r)/r} \right]} \\& \quad \times \left( {\begin{array}{*{20}c} {\cos n\theta } \\ { - \sin n\theta } \\ \end{array} } \right)e^{{i\left( { - \omega t + \bar{\gamma }z} \right)}} , \\ u_{\theta } &= \sum\limits_{n = 0}^{\infty } {\left[ { - F_{n} nH_{n}^{(1)} (\alpha r)/r + D_{2n} i\bar{\gamma }H_{n + 1}^{(1)} (\beta r) - M_{1n} \tfrac{{{\text{d}}H_{n}^{(1)} (\beta r)}}{{{\text{d}}r}}} \right]} \\ & \quad \times \left( {\begin{array}{*{20}c} {sinn\theta } \\ {\cos n\theta } \\ \end{array} } \right)e^{{i\left( { - \omega t + \bar{\gamma }z} \right)}} , \\ u_{z} &= \sum\limits_{n = 0}^{\infty } {\left[ { - F_{n} i\bar{\gamma }H_{n + 1}^{(1)} (\alpha r) - D_{2n} \left[ {\frac{{{\text{d}}H_{n + 1}^{(1)} (\beta r)}}{{{\text{d}}r}} + \frac{n + 1}{r}H_{n + 1}^{(1)} (\beta r)} \right]} \right]} \\& \quad \times\left( {\begin{array}{*{20}c} {\cos n\theta } \\ { - sinn\theta } \\ \end{array} } \right)e^{{i\left( { - \omega t + \bar{\gamma }z} \right)}} . \\ \end{aligned}$$
(8)

Expressions (4) contain three arbitrary constants. \(F_{n} ,D_{2n} ,M_{1n}\) and satisfy the equations of motion at arbitrary frequencies \(\omega\) and constant spread \(\bar{\gamma }\). In case of stress free cylindrical surface \(r = 1\), and if it is equal to zero normal \((\sigma_{rr} = 0)\) and tangential stresses \((\sigma_{r\theta } = \sigma_{rz} = 0)\), dispersion equation has the form

$$\left\| {c_{ij} } \right\| = 0,\quad i,j = 0,1,2,3 .$$
(9)

where

$$\begin{aligned} & c_{11}^{{}} = (n^{2} - 1 - \varOmega^{2} + \bar{\gamma }^{2} )H_{n}^{(1)} (\alpha ), \\ & c_{12}^{{}} = (n^{2} - 1 - \beta^{2} )H_{n}^{(1)} (\beta ),\, \\ & c_{13}^{{}} = 2(n^{2} - 1)\left[ {\beta H_{n - 1}^{(1)} (\beta ) - nH_{n}^{(1)} (\beta )} \right] - \beta^{2} H_{n}^{(1)} (\beta ), \\ & c_{21}^{{}} = \alpha H_{n - 1}^{(1)} (\alpha ) - (n + 1)H_{n}^{(1)} (\alpha ), \\ & c_{22}^{{}} = \beta H_{n - 1}^{(1)} (\beta ) - (n + 1)H_{n}^{(1)} (\beta ), \\ & c_{23}^{{}} = (2n^{2} + n - \bar{\gamma }^{2} )H_{n}^{(1)} (\beta ) - 2\beta H_{n - 1}^{(1)} (\beta ), \\ & c_{31}^{{}} = \alpha H_{n - 1}^{(1)} (\alpha ) - nH_{n}^{(1)} (\alpha ), \\ & c_{32}^{{}} = (1 - \varOmega^{2} /2\bar{\gamma }^{2} )(H_{n - 1}^{(1)} (\beta ) - nH_{n}^{(1)} (\beta )), \\ & c_{33}^{{}} = n^{2} H_{n}^{(1)} (\beta ). \\ \end{aligned}$$
(10)

Equation (9) for each value n = 1, 2, 3, … determines the relationship of the dimensionless frequency for \(\varOmega \,\) dimensionless constant distribution \(\bar{\gamma }\) at Poisson’s ratio. The arguments of the Henkel functions of the 1st kind of the nth order (10) are multivolume functions \(\alpha = (\varOmega /k^{2} - \bar{\gamma }^{2} )^{1/2} ,\,\,\beta = (\varOmega - \bar{\gamma }^{2} )^{1/2} .\)

The most effective way to solve Eq. (9) is the Muller method [18], which was used here. Without revealing the frequency to determine, and calculating at each step only its value for a fixed value ω, the indicated method finds its own complex frequencies \(\omega = \omega_{R} + i\omega_{I}\). The damping coefficients make it possible to judge the damping properties of the system under consideration. In the technique, another characteristic is used to estimate the damping rate of oscillatory processes, namely, the logarithmic decrement of oscillation damping. It is related to the damping coefficient by the following formula:

$$\delta = 2\pi \omega_{I} /\omega_{R} .$$

Complex series calculation up to 10−8. All expressions for stresses and displacements are:

$$\left( {R + i\text{Im} } \right)e^{ - iwt} = \left( {R^{2} + \text{Im}^{2} } \right)^{1/2} e^{{ - i\left( {wt - \gamma } \right)}} .$$

As you can see, the solution of the problem is expressed in terms of the special functions of Bessel and Henkel of the 1st and 2nd kind. With an increase in their argument, series (8) converges. Therefore, on the basis of numerical experiments, it was found that the accuracy of 5–6 members of the series accuracy reached 10−6–10−8. As a relaxation core of a viscoelastic material, let’s take a three-parameter core

$$R\left( t \right) = \frac{{Ae^{ - \beta t} }}{{t^{1 - \alpha } }}$$

Rzhanitsyn–Koltunov [20], having a weak singularity, where \(A,\alpha ,\beta\)—parameters materials [20]. Take the following parameters: \(A = 0.048;\quad \beta = 0.05;\quad \alpha = 0.1.\)

Oscillation of a Cylindrical Hole

First, consider the natural oscillations of a cylindrical hole (unsupported hole) in an elastic medium. At the boundary r = R, we insert a stress free condition, i.e.

$$\sigma_{rr} \left| {_{r = a} = \sigma_{r\theta } } \right|_{r = a} = 0.$$
(11)

After substituting (8) into the boundary conditions (11), we obtain a system of algebraically equations with complex coefficients. In order for a system of algebraic equations to have a non-trivial solution, it is necessary that the main determinant must be zero. The elements of the main determinant contain \(\omega .\) If the main determinant is equal to zero, the result is a transcendental equation for the frequency \(\omega ,\) which describes the natural oscillations of the cavity

$$\begin{aligned}& D_{\rho } = xH_{\rho - 1} \,\left[ {(\rho^{2} - 1)yH_{\rho - 1} (y) - (\rho^{3} - \rho \, + \,y^{2} /2)H_{\rho } (y)} \right] \\& \quad- H_{\rho } (x)\left[ {(\rho^{3} - \,\rho \, + \,y^{2} /2)yH_{\rho - 1} (y) - (\rho^{2} + \rho - y^{2} /4)y^{2} H_{\rho } (y)} \right], \end{aligned}$$
(12)

where \(x = \omega a(\rho/(\lambda + 2\mu))^{1/2};\;y = \omega a(\rho/\mu)^{1/2},\lambda\) and \(\mu\)—Lame coefficients; \(\rho\)—material density. Equation (12) after some transformations can be written as follows:

$$(\rho^{2} - 1)F(x)F(y) - (y^{2} /2)F(x) + F(y) + \rho^{2} - (\rho^{2} - y^{2} /2)^{2} = 0,$$

where \(F(x) = xH_{\rho }^{1} (x)/H_{\rho } (x),\;\rho = 1,2,3 \ldots .\)

Frequency Eq. (12) is solved numerically, i.e., Muller’s method [20]. Calculation results \((n \ge 0\,(v_{1} = 0.25))\) natural oscillations are shown in Table 1. As can be seen from the table, the corresponding complex frequencies increase with increasing number of waves around the circumference.

Table 1 The dependence of the complex natural frequencies of the cylindrical hole
Full size table

Complex frequencies consist of two parts, real (Re \(\varOmega\)) and imaginary parts

(Im \(\varOmega\)), which mean natural frequencies and damping coefficients [20]. Frequency Eq. (12) depend only on the parameter \(\nu\) (Poisson’s ratio). With an increase in Poisson’s ratio within \(0 \le \nu \le 0.4\) real and imaginary parts of the complex frequency vary up to 27%. With \(\nu_{1} = 0.5\) the environment becomes incompressible, the damping is naturally absent.

  1. 1.

    Axisymmetric waves in a cylinder

Representations of the components of the displacement vector for axisymmetric wave motions are obtained when n = 0. There are two different types of motions possible.

$$\begin{aligned} & u_{r} = \left[ {F_{0} \frac{{{\text{d}}H_{0}^{(1)} (\alpha r)}}{{{\text{d}}r}} + D_{20} i\bar{\gamma }H_{1}^{(1)} (\beta r) + M_{10n} nH_{n}^{(1)} (\beta r)/r} \right]e^{{i\left( { - \omega t + \bar{\gamma }z} \right)}} \\ & u_{z} = - F_{0} i\bar{\gamma }H_{1}^{(1)} (\alpha r) - D_{20} \left[ {\frac{{{\text{d}}H_{1}^{(1)} (\beta r)}}{{{\text{d}}r}} + \frac{1}{r}H_{1}^{(1)} (\beta r)} \right]e^{{i\left( { - \omega t + \bar{\gamma }z} \right)}} \\ & u_{\theta } = 0 \\ \end{aligned}$$
(13)

and

$$\begin{aligned} & u_{\theta } = D_{20} i\bar{\gamma }H_{1}^{(1)} (\beta r)e^{{i\left( { - \omega t + \bar{\gamma }z} \right)}} , \\ & u_{r} = u_{z} = 0. \\ \end{aligned}$$
(14)

The first of these is associated with the propagation of longitudinal waves, and the second with torsional waves in a cylindrical cavity. As an example, consider the propagation of free axisymmetric waves in a cylindrical cavity located in an elastic medium (8). Then the corresponding radial and tangential stresses in the displacement potentials take the form

$$\begin{aligned} & \sigma_{rr} = \rho_{1} \frac{\nu }{1 - \nu }\frac{{\partial^{2} \varphi }}{{\partial t^{2} }} + 2c_{s}^{2} \rho_{1} \left( {\frac{{\partial^{2} \varphi }}{{\partial r^{2} }} - \frac{{\partial^{2} \psi }}{\partial r\partial z}} \right), \\ & \sigma_{rz} = \rho_{1} \frac{{\partial^{2} \psi }}{{\partial t^{2} }} + 2c_{s}^{2} \rho_{1} \left( {\frac{{\partial^{2} \varphi }}{\partial r\partial z} - \frac{{\partial^{2} \psi }}{{\partial z^{2} }}} \right), \\ \end{aligned}$$
(15)

where \(\rho_{1}\)—medium density, \(c_{s}\)—speed of propagation of transverse waves.

The solution of the equation of motion (15), taking into account the conditions of Sommerfeld radiation at infinity, takes the form

$$\varphi = F_{0} H_{0} (\bar{\alpha}r)\exp (i(- \omega \,t + \gamma_{p} z));\,\,\psi = M_{0} H_{0} (\bar{\beta}r)\exp (i(- \omega t + \gamma_{p} z)),$$
(16)

where \(\bar{\alpha } = i\alpha ,\,\,\,\bar{\beta } = i\beta .\)

Then the corresponding radial and tangential stresses at the boundary of the cavity (\(r = R\)) are zero

$$\begin{aligned} & \rho_{1} \frac{\nu }{1 - \nu }\frac{{\partial^{2} \varphi }}{{\partial t^{2} }} + 2c_{s}^{2} \rho_{1} \left( {\frac{{\partial^{2} \varphi }}{{\partial r^{2} }} - \frac{{\partial^{2} \psi }}{\partial r\partial z}} \right) = 0, \\ & \rho_{1} \frac{{\partial^{2} \psi }}{{\partial t^{2} }} + 2c_{s}^{2} \rho_{1} \left( {\frac{{\partial^{2} \varphi }}{\partial r\partial z} - \frac{{\partial^{2} \psi }}{{\partial z^{2} }}} \right) = 0. \\ \end{aligned}$$
(17)

Substituting (16) into (17) we obtain the following homogeneous system of algebraic equations

$$\begin{aligned} M_{0}^{{}} H_{1}^{(1)} (\bar{\beta }a)(2c_{s}^{2} \gamma_{p}^{2} - \omega^{2} ) - 2c_{s}^{2} \bar{\alpha }\gamma_{p}^{{}} F_{0} H_{0}^{{(1){\prime }}} (\bar{\alpha }a) = 0, \hfill \\ - \rho_{1}^{{}} \omega^{2} bF_{0} H_{0}^{(1)} (\bar{\alpha }a) + 2c_{s}^{2} \rho_{1} \left[ {F_{0} \bar{\alpha }^{2} H_{0}^{{(1){\prime \prime }}} (\bar{\alpha }a) - M_{0}^{{}} \bar{\beta }\gamma_{p} H_{1}^{{(1)^{{\prime }} }} (\bar{\beta }a)} \right] = 0, \hfill \\ \end{aligned}$$
(18)

where \(b = \nu /(1 - \nu ).\)

If the system of homogeneous algebraic Eq. (18) has solutions, then the main determinant must be zero. After some transformations, we obtain the following dispersion equation

$$\begin{aligned} &4(1 - \xi_{1}^{2} )\left[ {\frac{1}{\beta a} + \frac{{H_{0}^{(1)} (\bar{\beta }a)}}{{H_{1}^{(1)} (\bar{\beta }a)}}} \right] - 2(1 - \xi_{1}^{2} )(1 - \xi_{2}^{2} )^{1/2}\\& \quad \times \left[ {\frac{1}{{\bar{\alpha }a}} + \frac{{H_{0}^{(1)} (\bar{\alpha }a)}}{{H_{1}^{(1)} (\bar{\alpha }a)}}} \right] + b\frac{{\xi_{1}^{2} (2 - \xi_{1}^{2} )}}{{(1 - \xi_{2}^{2} )^{1/2} }}\frac{{H_{0}^{(1)} (\bar{\alpha }a)}}{{H_{1}^{(1)} (\bar{\alpha }a)}} = 0, \end{aligned}$$
(19)

In Eq. (19) we introduce the notation \(\varPsi (\alpha )\), which describes the relation of cylindrical functions with indices 0 and 1. In the case of a cylindrical cavity, the waves must decrease at infinity (Summerfield conditions), so that the Henkel functions of the first kind come \(\varPsi_{K} (y) = K_{0}^{{}} (y)/K_{1}^{{}} (y)\). Solutions (19) describing damped waves correspond to real \(\gamma_{p}\). Where in \(\gamma_{p} \succ k_{2} \succ k_{1}\), a \(C_{ph} \prec c_{s} \prec c_{p}\). Then \(\alpha ,\beta\)-Imaginary and it is possible to transition to the functions of Macdonald:

$$\varPsi_{H01} (\alpha ) = H_{0}^{(1)} (\alpha )/H_{1}^{(1)} (\alpha ) = - iK_{0}^{{}} (\left| \alpha \right|)/K_{1}^{{}} (\left| \alpha \right|) = - i\varPsi_{K} (\left| \alpha \right|),$$
(20)

where \(\varPsi_{K} (y) = K_{0}^{{}} (y)/K_{1}^{{}} (y).\)

Then, (19) takes the following form

$$\begin{aligned} &4(1 - \xi_{1}^{2} )\left[ {\frac{1}{\beta a} + \varPsi_{H01} (\bar{\beta }a)} \right] - 2(1 - \xi_{1}^{2} )(1 - \xi_{2}^{2} )^{1/2}\\& \quad \times \left[ {\frac{1}{{\bar{\alpha }a}} + \varPsi_{H01} (\bar{\alpha }a)} \right] + b\frac{{\xi_{1}^{2} (2 - \xi_{1}^{2} )}}{{(1 - \xi_{2}^{2} )^{1/2} }}\varPsi_{H01} (\bar{\alpha }a) = 0. \end{aligned}$$
(21)

To describe the viscoelastic properties of the material, the Boltzmann–Voltaire hereditary theory with the Rzhanitsyn–Koltunov relaxation core was used in the form \(R(t) = Ae^{ - \beta t} t^{\alpha - 1}\). In this case, the sine of G(s) and cosine Г(c) Fourier samples core relaxation R(t) is expressed through Г(α)—Gamma function:

$$\begin{aligned} \varGamma^{S}& = \frac{A\varGamma (\alpha)}{{(\omega^{2} + \beta^{2})^{{{\raise0.7ex\hbox{$\alpha $} \!\mathord{\left/{\vphantom {\alpha 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}}}}}\text{Sin} \left({\alpha \,{\text{arc}}\,tg\frac{\omega}{\beta}} \right),\quad \\ \varGamma^{C}&= \frac{\varGamma (\alpha)}{{(\omega^{2} + \beta^{2})^{{{\raise0.7ex\hbox{$\alpha $} \!\mathord{\left/{\vphantom {\alpha 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}}}}}{\text{s}}\left({\alpha \,{\text{arc}}\,tg\frac{\omega}{\beta}} \right). \end{aligned}$$

Figure 1 shows the solution of Eq. (21) in the form of a family of curves whose parameter is the Poisson’s ratio—the only elastic parameters of the relaxation core

$$A = 0.01;\quad \beta = 0.05;\quad \alpha = 0.1,$$

on which depends the type of dispersion curve. Here \(U\varPsi_{k} = C_{ph} /c\)—normalized phase velocity, \(\xi = R\omega /c\)—normalized quantity related to the radius of curvature and frequency.

Fig. 1
figure 1

The change in phase velocity from the radius of curvature at \(1.\,\nu = 0.1;\,\,2.\,\nu = 0.18;\,\,3.\,\nu = 0.25;\,4.\,\nu = 0.4\)

Full size image

It can be seen that the critical value ξ = ξZ, below which begins extreme mode, when no mode exists, but the phase velocity of the surface wave, which takes the maximum value at this point, is always C. For any \(\xi \ge \xi_{Z}\) dependences are obtained rapidly decaying with distance from the boundary, which confirms the surface character of the waves, moreover, if the rate of decay ur with increasing ν decreases. Decay rate uz from ν does not depend. Components ur and uz are always in quadrature, and, thus, the movement of particles in a substance during the propagation of axisymmetric waves along the surface of a cylindrical cavity is completely analogous to the movement of particles during the propagation of an ordinary Rayleigh wave [19, 20]. The points of the medium move along ellipses lying in the so-called sagittal planes, which in this case are all the planes passing through the axis of the cylinder. If \(\gamma_{p} a \to \infty\) and use the asymptotic expression of the Hankel function, then from (21) and taking into account (22) we obtain the following Rayleigh equation

$$4(1 - \xi_{1}^{2} )^{1/2} - \frac{{(2 - \xi_{1}^{2} )^{2} }}{{(1 - \xi_{2}^{2} )^{1/2} }} = 0.$$
(22)

Numerical results: the dependence of the surface wave velocity on the wavelength at \(\,\nu = 0;\,\,\,\nu = 0.25;\,\,\nu = 0.4\) shown in Fig. 2. Despite the apparent surface character, axisymmetric waves on the surface of a cylindrical cavity differ from Rayleigh waves on a plane by the presence of dispersion. At the same time, as for a surface wave on a plane, but unlike cylindrical systems with a limited linear volume, in-phase (synchronous harmonic oscillations are comparable at any time in phases) oscillations of the cavity are impossible. In the case of a rod, it is the finite size of the cross section that leads to a lot of modality, since it forms a resonator having characteristic eigen frequencies at which common-mode oscillation is possible. From the study of the surface wave at specific values of the radius of curvature on the surface of an extended cylindrical cavity in the volume of a known material (steel) simulating a pipe with sufficiently thick walls, the following is obtained: for the central casing of an oil or gas well, which is supposed to be examined, in the entire range of interest frequency (from 0 to 30 kHz) the existence of waves of this type is impossible, even if these frequencies lie slightly above the cutoff. It can be shown that even at 30 kHz the distance over which the amplitude dropped at least 9 times exceeds 12 cm with an internal radius of about 10 cm. From the calculations it follows that up to 48 kHz wave attenuation at a depth equal to the thickness of the investigated casing string, does not exceed 0.76 times, i.e., from the point of view of surface effects, these are very thin walls. This fact is favorable for the development of low-frequency probing complexes, since allows you to choose the form and method of excitation of oscillations, without fear that the energy that should bring information about the deep layers of the system will go “wasted” along the axis.

Fig. 2
figure 2

The dependence of the velocity of the surface wave on the wavelength \(1.\,\nu = 0;\,\,2.\,\,\nu = 0.25;\,3.\,\nu = 0.4\)

Full size image

Conclusions

  1. 1.

    The dispersion dependences of the phase velocity on frequency for axisymmetric and nonaxisymmetric elastic waves on the surface of a cylindrical circular cavity with different values of the Poisson coefficient of the medium are constructed; a region of non-propagation of waves of this type at low frequencies or at small radii of curvature is noted.

  2. 2.

    The dependence of the critical value of the normalized radius of curvature and frequency, on the value of Poisson’s ratio material surrounding the cavity.