Abstract
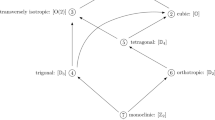
Harmonic and Cartan decompositions are used to prove that there are eight symmetry classes of elasticity tensors. Recent results in apparent contradiction with this conclusion are discussed in a short history of the problem.
Similar content being viewed by others
References
G. Backus, A geometrical picture of anisotropic elastic tensors. Reviews of Geophysics and Space Physics 8 (1970) 633–671.
R. Baerheim, Harmonic decomposition of the anisotropic elasticity tensor. Q. J. Mech. Appl. Math. 46 (1993) 391–419.
S. Bhagavantam and T. Venkatarayudu, Theory of Groups and its Application to Physical Problems. Academic Press, New York-London (1969).
J.P. Boehler, A.A. Kirillov, and E.T. Onat, On the polynomial invariants of the elasticity tensor. J. Elasticity 34 (1994) 97–110.
S.C. Cowin, Properties of the anisotropic elasticity tensor. Q. J. Mech. Appl. Math. 42 (1989) 249–266.
S.C. Cowin, Optimization of the strain energy density in linear anisotropic elasticity. J. Elasticity 34 (1994) 45–68.
S.C. Cowin, On the number of distinct elastic constants associated with certain anisotropic elastic symmetries. Z. angew. Math. Phys. (ZAMP) 46 (Special Issue): (1995) S210-S224.
S.C. Cowin and M.M. Mehrabadi, On the identification of material symmetry for anisotropic elastic materials. Q. J. Mech. Appl. Math. 40 (1987) 451–476.
S.C. Cowin and M.M. Mehrabadi, The structure of the linear anisotropic elastic symmetries. J. Mech. Phys. Solids 40 (1992) 1459–1471.
A. Danescu, An application of the trace formula to anisotropic nonlinear elasticity. To be published in: J. Elasticity.
F.I. Fedorov, Theory of Elastic Waves in Crystals, Plenum Press, New York (1968).
R. Fieschi, Matter tensors in the crystallographic groups of cartesian symmetry. Physica 24 (1957) 972–976.
R. Fieschi and F.G. Fumi, Matter tensors in symmetrical systems. Nuovo Cimento 9 (1952) 739–756.
R. Fieschi and F.G. Fumi, High-order tensors in symmetrical systems. Nuovo Cimento 10 (1953) 865–882.
F.G. Fumi, Photoelasticity in crystals. Nuovo Cimento 9 (1952) 184–185.
V.L. German, Some theorems on anisotropic media. Doklady Akad. Nauk SSSR 48 (1945) 95–98. In Russian.
M. Golubitsky, I. Stewart and D.G. Schaeffer, Singularities and Groups in Bifurcation Theory Vol. 2. Springer-Verlag, New York-Berlin (1985).
M.E. Gurtin, The linear theory of elasticity. In C. Truesdell, editor, Mechanics of Solids II volume VIa/2 of Handbüch der Physik, pages 1–295. Springer, Berlin (1972).
R.F.S. Hearmon, An Introduction to Applied Anisotropic Elasticity. Oxford University Press, London (1961).
C. Hermann, Tensoren und Kristallsymmetrie. Zeitschr. f. Kristallographie, 89 (1934) 32–48.
Y.-Z. Huo and G.Del Piero, On the completeness of the crystallographic symmetries in the description of the symmetries of the elastic tensor. J. Elasticity 25 (1991) 203–246.
H. Jagodinski, Kristallographie. In S. Flügge, editor, Kristallphysik I, volume VII/1 of Handbüch der Physik pages 1–103. Springer, Berlin (1955).
J.P. Jaric, On the conditions for the existence of a plane of symmetry for anisotropic elastic material. Mech. Res. Comm. 21 (1994) 153–174.
A.G. Khaktevich, The elastic constants of crystals. Kristallografiya 6 (1961) 700–703.
V.A. Koptsik and Yu. I. Sirotin, The symmetry of piezoeletric and elastic tensors and the symmetry of the physical properties of crystals. Kristallografiya 6 (1961) 766–768.
L.D. Landau and E.M. Lifshiz, Theory of Elasticity, volume 7 of Course of Theoretical Physics Pergamon Press, London (1959).
S.G. Lekhnitskii, Theory of Elasticity of an Anisotropic Elastic Body Holden-Day Series in Mathematical Physics. Holden-Day, San Francisco (1963).
D.E. Littlewood, The Theory of Group Characters and Matrix Representations of Groups Oxford University Press, Oxford (1940).
D.E. Littlewood, Invariant theory under orthogonal groups. Proc. Lond. Math. Soc. 50 (1949) 349–379.
A.E.H. Love, A Treatise on the Mathematical Theory of Elasticity University Press, Cambridge (1927).
M.M. Mehrabadi and S.C. Cowin, Eigentensors of linear anisotropic elastic materials. Q. J. Mech. Appl. Math. 43 (1990) 15–41.
W. Miller, Symmetry Groups and their Applications Academic Press, New York-London (1972).
E. Mochizuki, Spherical harmonic decomposition of an elastic tensor. Geophysical Journal 93 (1988) 521–526.
E.T. Onat, Effective properties of elastic materials that contain penny shaped voids. Internat. J. Engrg. Sci. 22 (1984) 1013–1021.
J.F. Pierce, Representations for transversely hemitropic and transversely isotropic stress-strain relations. J. Elasticity 37 (1995) 243–280.
P. Podio-Guidugli and M. Vianello, Internal constraints and linear constitutive relations for transversely isotropic materials. Rend. Mat. Acc. Lincei 2 (1991) 241–248.
J. Rychlewski, Unconventional approach to linear elasticity. Arch. Mech. 47 (1995) 149–171.
J.A. Schouten, Der Ricci-Kalkül Springer, Berlin (1924).
J.A. Schouten, Tensor Analysis for Physicists Oxford University Press, London (1951).
J.A. Schouten, Ricci-Calculus Springer, Berlin (1954).
I.R. Shafarevich, Basic notions of algebra. In A.I. Kostrikin and I.R. Shafarevich, editors, Algebra I, volume 11 of Encyclopaedia of Mathematical Sciences pages 1–258. Springer, Berlin (1990).
Yu.I. Sirotin, Group tensor spaces. Kristallografiya 5 (1960) 157–320.
C. Somigliana, Sulla legge di razionalità rispetto alle proprietà elastiche dei cristalli. Atti Reale Accad. Naz. Lincei (Rend.) 3 (1894) 238–246.
A.J.M. Spencer, A note on the decomposition of tensors into traceless symmetric tensors. Internat. J. Engrg. Sci. 8 (1970) 475–481.
E.S. Suhubi, Thermoelastic solids. In A. Cemal Eringen, editor, Continuum Mechanics of Songle-Substance Bodies volume II of Continuum Physics pages 173–275 Academic Press, New York (1975).
S. Sutcliffe, Spectral decomposition of the elasticity tensor. Trans. ASME J. Appl. Mech. 59 (1992) 762–773.
R.N. Thurston, Waves in solids. In C. Truesdell, editor, Mechanics of Solids IV volume VIa/4 of Handbüch der Physik pages 109–308. Springer, Berlin (1974).
L.J. Walpole, Fourth-rank tensors of the thirty-two crystal classes: Multiplication tables. Proc. R. Soc. Lond. A 391 (1984) 149–179.
H. Weyl, The Classical Groups, Their Invariants and Representations, Princeton University Press, Princeton (1946).
Q.-S. Zheng, Theory of representations for tensor functions — a unified invariant approach to constitutive equations. AMR Applied Mechanics Reviews 47 (1994) 545–587.
Q.-S. Zheng and J.P. Boehler, The description, classification and reality of material and physical symmetries. Acta Mech. 102 (1994) 73–89.
Q.-S. Zheng and A.J.M. Spencer, On the canonical representations for Kronecker powers of orthogonal tensors with application to material symmetry problems. Internat. J. Engrg. Sci. 31 (1993) 617–635.
Author information
Authors and Affiliations
Additional information
Research supported by GNFM of CNR (Italy)
Rights and permissions
About this article
Cite this article
Forte, S., Vianello, M. Symmetry classes for elasticity tensors. J Elasticity 43, 81–108 (1996). https://doi.org/10.1007/BF00042505
Received:
Issue Date:
DOI: https://doi.org/10.1007/BF00042505