Skip to main content
SpringerLink
Log in

Surfactants in Foam Stability: A Phase-Field Model

  • Published:
Archive for Rational Mechanics and Analysis Aims and scope Submit manuscript

Abstract

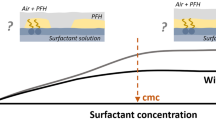
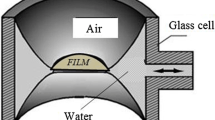
The role of surfactants in stabilizing the formation of bubbles in foams is studied using a phase-field model. The analysis is centered on a van der Walls–Cahn– Hilliard-type energy with an added term which accounts for the interplay between the presence of a surfactant density and the creation of interfaces. In particular, it is concluded that the surfactant segregates to the interfaces, and that the prescription of the distribution of surfactant will dictate the locus of interfaces, which is in agreement with the experimental results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Ambrosio L., Fusco N., Pallara D. (2000) Functions of Bounded Variation and Free Discontinuity Problems. Oxford University Press, New York

    MATH  Google Scholar 

  2. Ambrosio L., De Lellis C., Mantegazza C. (1999) Line energies for gradient vector fields in the plane. Calc. Var. Partial Differential Equations 9, 327–355

    Article  MathSciNet  Google Scholar 

  3. Aviles P., Giga Y. (1999) On lower semicontinuity of a defect energy obtained by a singular limit of the Ginzburg-Landau type energy for gradient fields. Proc. Roy. Soc. Edinburgh Sect. A 129, 1–17

    MathSciNet  Google Scholar 

  4. Baldo S. (1990) Minimal interface criterion for phase transitions in mixtures of Cahn-Hilliard fluids. Ann. Inst. H. Poincaré Anal. Non Linéaire 7, 67–90

    MathSciNet  Google Scholar 

  5. Barroso A.C., Fonseca I. (1994) Anisotropic singular perturbations–the vectorial case. Proc. Roy. Soc. Edinburgh Sect. A 124, 527–571

    MathSciNet  Google Scholar 

  6. Bouchittè G. (1990) Singular perturbations of variational problems arising from a two-phase transition model. Appl. Math. Optim. 21, 289–314

    Article  MathSciNet  Google Scholar 

  7. Bronsard L., Kohn R.V. (1990) On the slowness of phase boundary motion in one space dimension. Commun. Pure Appl. Math. 43, 983–997

    MathSciNet  Google Scholar 

  8. Carr J., Pego R. (1989) Metastable patterns in solutions of u t = ε2 u xx − f(u). Commun. Pure Appl. Math. 42, 523–576

    MathSciNet  Google Scholar 

  9. Conti S., Fonseca I., Leoni G. (2002) A Γ-convergence result for the two-gradient theory of phase transitions. Commun. Pure Appl. Math. 55, 857–936

    Article  MathSciNet  Google Scholar 

  10. Conti S., Schweizer B. (2006) A sharp-interface limit for the geometrically linear two-well problem in two dimensions. Arch. Ration. Mech. Anal. 179, 413–452

    Article  MathSciNet  Google Scholar 

  11. Dal Maso G. (1993) An Introduction to Γ-Convergence. Progress in Nonlinear Differential Equations and their Applications, 8. Birkhäuser Boston

    Google Scholar 

  12. Fonseca I., Mantegazza C. (2000) Second order singular perturbation models for phase transitions. SIAM J. Math. Anal. 31, 1121–1143

    Article  MathSciNet  Google Scholar 

  13. Fonseca I., Tartar L. (1989) The gradient theory of phase transitions for systems with two potential wells. Proc. Roy. Soc. Edinburgh Sect. A 111, 89–102

    MathSciNet  Google Scholar 

  14. Grant C.P. (1995) Slow motion in one-dimensional Cahn-Morral systems. SIAM J. Math. Anal. 26, 21–34

    Article  MathSciNet  Google Scholar 

  15. Gurtin M.E. (1987) Some results and conjectures in the gradient theory of phase transitions. Metastability and Incompletely Posed Problems. IMA Volumes in Mathematics and Its Applications, 3, pp. 135–146. Springer, New York

    Google Scholar 

  16. Gurtin M.E., Matano H. (1988) On the structure of equilibrium phase transitions within the gradient theory of fluids. Quart. Appl. Math. 46, 301–317

    MathSciNet  Google Scholar 

  17. Jin W., Kohn R.V. (2000) Singular perturbation and the energy of folds. J. Nonlinear Sci. 10, 355–390

    Article  ADS  MathSciNet  Google Scholar 

  18. Kraynik A.M. (2003) Foam structure: From soap froth to solid foams. MRS Bulletin 28, 275–278

    Google Scholar 

  19. Kohn R.V., Sternberg P. (1989) Local minimisers and singular perturbations. Proc. Roy. Soc. Edinburgh Sect. A 111, 69–84

    MathSciNet  Google Scholar 

  20. Modica L., Mortola S. Un esempio di Γ−-convergenza. Boll. Unione Mat. Ital. Sez. B Artic. Ric. Mat. (8) 14, 285–299 (1977)

  21. Modica L. (1987) The gradient theory of phase transitions and the minimal interface criterion. Arch. Ration. Mech. Anal. 98, 123–142

    Article  MathSciNet  Google Scholar 

  22. Owen N.C., Sternberg P. (1991) Nonconvex variational problems with anisotropic perturbations. Nonlinear Anal. 16, 705–719

    Article  MathSciNet  Google Scholar 

  23. Perkins, R., Sekerka, R., Warren, J., Langer, S. Private communication

  24. Rockafellar R.T. (1970) Convex Analysis. Princeton Mathematical Series, no. 28, Princeton University Press, Princeton

    MATH  Google Scholar 

  25. Sternberg P. (1988) The effect of a singular perturbation on nonconvex variational problems. Arch. Ration. Mech. Anal. 101, 209–260

    Article  MathSciNet  Google Scholar 

  26. Sternberg P. Vector-valued local minimizers of nonconvex variational problems. Current directions in nonlinear partial differential equations. Rocky Mountain J. Math. 21, 799–807

Download references

Author information

Authors and Affiliations

  1. Department of Mathematical Sciences, Carnegie Mellon University, Pittsburgh, PA, 15213, USA

    Irene Fonseca

  2. Classe di Matematica, SISSA, Via Beirut 2-4, 34014, Miramare Grignano (Trieste), Italy

    Massimiliano Morini

  3. Mathematics Institute, University of Warwick, Coventry, CV4 7AL, UK

    Valeriy Slastikov

Authors
  1. Irene Fonseca
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Massimiliano Morini
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Valeriy Slastikov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Irene Fonseca.

Additional information

Communicated by L. Ambrosio

Rights and permissions

Reprints and permissions

About this article

Cite this article

Fonseca, I., Morini, M. & Slastikov, V. Surfactants in Foam Stability: A Phase-Field Model. Arch Rational Mech Anal 183, 411–456 (2007). https://doi.org/10.1007/s00205-006-0012-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00205-006-0012-x

Keywords

Search

Navigation