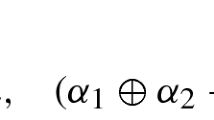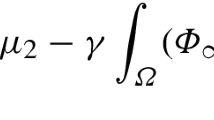Abstract
Given two densities f and g, we consider the problem of transporting a fraction \({m \in [0,\min\{\|f\|_{L^1},\|g\|_{L^1}\}]}\) of the mass of f onto g minimizing a transportation cost. If the cost per unit of mass is given by |x − y|2, we will see that uniqueness of solutions holds for \({m \in [\|f\wedge g\|_{L^1},\min\{\|f\|_{L^1},\|g\|_{L^1}\}]}\) . This extends the result of Caffarelli and McCann in Ann Math (in print), where the authors consider two densities with disjoint supports. The free boundaries of the active regions are shown to be (n − 1)-rectifiable (provided the supports of f and g have Lipschitz boundaries), and under some weak regularity assumptions on the geometry of the supports they are also locally semiconvex. Moreover, assuming f and g supported on two bounded strictly convex sets \({{\Omega,\Lambda \subset \mathbb {R}^n}}\) , and bounded away from zero and infinity on their respective supports, \({C^{0,\alpha}_{\rm loc}}\) regularity of the optimal transport map and local C 1 regularity of the free boundaries away from \({{\Omega\cap \Lambda}}\) are shown. Finally, the optimal transport map extends to a global homeomorphism between the active regions.
Similar content being viewed by others
References
Alvarez O., Cardaliaguet P., Monneau R.: Existence and uniqueness for dislocation dynamics with positive velocity. Interf. Free Bound. 7(4), 415–434 (2005)
Alberti G., Ambrosio L.: A geometrical approach to monotone functions in \({\mathbb{R}^n}\). Math. Z. 230(2), 259–316 (1999)
Ambrosio, L., Fusco, N., Pallara, D.: Functions of bounded variation and free discontinuity problems. Oxford Mathematical Monographs. The Clarendon Press, Oxford University Press, 2000
Ambrosio, L., Gigli, N., Savaré, G.: Gradient flows in metric spaces and in the space of probability measures. Lectures in Mathematics ETH Zurich. Birkhauser, Basel, 2005
Brenier Y.: Polar decomposition and increasing rearrangement of vector fields. C. R. Acad. Sci. Paris Sér. I Math. 305(19), 805–808 (1987)
Brenier Y.: Polar factorization and monotone rearrangement of vector-valued functions. Comm. Pure Appl. Math. 44, 375–417 (1991)
Caffarelli L.: The regularity of mappings with a convex potential. J. Am. Math. Soc. 5(1), 99–104 (1992)
Caffarelli, L., McCann, R.J.: Free boundaries in optimal transport and Monge–Ampère obstacle problems. Ann. of Math. (in print)
Cordero-Erasquin D., McCann R.J., Schmuckenschlager M.: Riemannian interpolation inequality à la Borell, Brascamp and Lieb. Invent. Math. 146(2), 219–257 (2001)
Dellacherie, C., Meyer, P.-A.: Probabilities and potential. North-Holland Mathematics Studies. 29, North-Holland, Amsterdam/New York, 1978
Evans L.C., Gariepy R.F.: Measure Theory and Fine Properties of Functions. Stud. Adv. Math. . CRC Press, Boca Raton (1992)
Fathi, A., Figalli, A.: Optimal transportation on non-compact manifolds. Israel J. Math. (to appear)
Figalli A.: Existence, Uniqueness, and Regularity of Optimal Transport Maps. SIAM J. Math. Anal. 39(1), 126–137 (2007)
McCann R.J.: Existence and uniqueness of monotone measure-preserving maps. Duke Math. J. 80(2), 309–323 (1995)
McCann R.J.: A convexity principle for interacting gases. Adv. Math. 128(1), 153–179 (1997)
McCann R.J.: Polar factorization of maps on Riemannian manifolds. Geom. Funct. Anal. 11(3), 589–608 (2001)
Villani, C.: Optimal transport, old and new. Notes from the Saint-Flour 2005 Summer School. Grundlehren der mathematischen Wissenschaften (To appear). Preliminary version available at http://www.umpa.ens-lyon.fr/~cvillani
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by L. Ambrosio
Rights and permissions
About this article
Cite this article
Figalli, A. The Optimal Partial Transport Problem. Arch Rational Mech Anal 195, 533–560 (2010). https://doi.org/10.1007/s00205-008-0212-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00205-008-0212-7