Skip to main content
SpringerLink
Log in

A mathematical model and a numerical model for hyperbolic mass transport in compressible flows

  • Original
  • Published:
Heat and Mass Transfer Aims and scope Submit manuscript

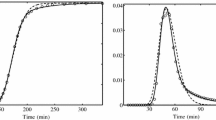
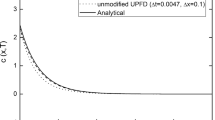
Abstract

A number of contributions have been made during the last decades to model pure-diffusive transport problems by using the so-called hyperbolic diffusion equations. These equations are used for both mass and heat transport. The hyperbolic diffusion equations are obtained by substituting the classic constitutive equation (Fick’s and Fourier’s law, respectively), by a more general differential equation, due to Cattaneo (C R Acad Sci Ser I Math 247:431–433, 1958). In some applications the use of a parabolic model for diffusive processes is assumed to be accurate enough in spite of predicting an infinite speed of propagation (Cattaneo, C R Acad Sci Ser I Math 247:431–433, 1958). However, the use of a wave-like equation that predicts a finite velocity of propagation is necessary in many other calculations. The studies of heat or mass transport with finite velocity of propagation have been traditionally limited to pure-diffusive situations. However, the authors have recently proposed a generalization of Cattaneo’s law that can also be used in convective-diffusive problems (Gómez, Technical Report (in Spanish), University of A Coruña, 2003; Gómez et al., in An alternative formulation for the advective-diffusive transport problem. 7th Congress on computational methods in engineering. Lisbon, Portugal, 2004a; Gómez et al., in On the intrinsic instability of the advection–diffusion equation. Proc. of the 4th European congress on computational methods in applied sciences and engineering (CDROM). Jyväskylä, Finland, 2004b) (see also Christov and Jordan, Phys Rev Lett 94:4301–4304, 2005). This constitutive equation has been applied to engineering problems in the context of mass transport within an incompressible fluid (Gómez et al., Comput Methods Appl Mech Eng, doi:10.1016/j.cma.2006.09.016, 2006). In this paper we extend the model to compressible flow problems. A discontinuous Galerkin method is also proposed to numerically solve the equations. Finally, we present some examples to test out the performance of the numerical and the mathematical model.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

References

  1. Compte A (1997) The generalized Cattaneo equation for the description of anomalous transport processes. J Phys A Math Gen 30:7277–7289

    Article  MATH  MathSciNet  Google Scholar 

  2. Özisik MN, Tzou DY (1994) On the wave theory in heat conduction. ASME J Heat Transf 116:526–535

    Article  Google Scholar 

  3. Fick A (1855) Uber diffusion. Poggendorff’s Ann Phys Chem 94:59–86

    Article  Google Scholar 

  4. Fourier JB (1822) Théorie analytique de la chaleur. Jacques Gabay

  5. Jiang F, Sousa ACM (2006) SPH numerical modeling for ballistic-diffusive heat conduction. Numer Heat Transf 50:499-515

    Article  Google Scholar 

  6. Vick B, Özisik MN (1983) Growth and decay of a thermal pulse predicted by the hyperbolic heat conduction equation. ASME J Heat Transf 105:902–907

    Article  Google Scholar 

  7. Cattaneo MC (1958) Sur une forme de l’equation de la chaleur éliminant le paradoxe d’une propagation instantaneé. C R Acad Sci Ser I Math 247:431–433

    MathSciNet  Google Scholar 

  8. Joseph DD, Preziosi L (1989) Heat waves. Rev Mod Phys 61:41–73

    Article  MATH  MathSciNet  Google Scholar 

  9. Joseph DD, Preziosi L (1990) Addendum to the paper “heat waves”. Rev Mod Phys 62:375–391

    Article  MathSciNet  Google Scholar 

  10. Duhamel P (2004) Application of a new finite integral transform method to the wave model of conduction. Int J Heat Mass Transf 47:573–588

    Article  MATH  Google Scholar 

  11. Ali YM, Zhang LC (2005) Relativistic heat conduction. Int J Heat Mass Transf 48:2397–2406

    Article  Google Scholar 

  12. Haji-Sheikh A, Minkowycz WJ, Sparrow EM (2002) Certain anomalies in the analysis of hyperbolic heat conduction. J Heat Transf 124:307–319

    Article  Google Scholar 

  13. Jiang F, Sousa ACM (2005) Analytical solution for hyperbolic heat conduction in a hollow sphere. AIAA J Thermophys Heat Transf 19:595–598

    Article  Google Scholar 

  14. Jiang F (2006) Solution and analysis of hyperbolic heat propagation in hollow spherical objects. Heat Mass Transf 42:1083–1091

    Article  Google Scholar 

  15. Arora M (1996) Explicit characteristic-based high resolution algorithms for hyperbolic conservation laws with stiff source terms. PhD dissertation, University of Michigan

  16. Carey GF, Tsai M (1982) Hyperbolic heat transfer with reflection. Numer Heat Transf 5:309–327

    Article  Google Scholar 

  17. Wu W, Li X (2006) Application of the time discontinuous Galerkin finite element method to heat wave simulation. Int J Heat Mass Transf 49:1679–1684

    Article  Google Scholar 

  18. Yang HQ (1992) Solution of two-dimensional hyperbolic heat conduction by high resolution numerical methods, AIAA-922937

  19. Manzari MT, Manzari MT (1999) On numerical solution of hyperbolic heat equation. Commun Numer Methods Eng 15:853–866

    Article  MATH  MathSciNet  Google Scholar 

  20. Shen W, Han S (2003) A numerical solution of two-dimensional hyperbolic heat conduction with non-linear boundary conditions. Heat Mass Transf 39:499–507

    Google Scholar 

  21. Gómez H (2003) A new formulation for the advective-diffusive transport problem. Technical Report (in Spanish), University of A Coruña

  22. Gómez H, Colominas I, Navarrina F, Casteleiro M (2004a) An alternative formulation for the advective-diffusive transport problem. In: Mota Soares CA, Batista AL, Bugeda G, Casteleiro M, Goicolea JM, Martins JAC, Pina CAB, Rodrigues HC (eds) 7th Congress on computational methods in engineering. Laboratório Nacional de Engenharia Civil, Lisboa (Portugal)

  23. Gómez H, Colominas I, Navarrina F, Casteleiro M (2004b) On the intrinsic instability of the advection–diffusion equation. In: Neittaanmäki P, Rossi T, Korotov S, Oñate E, Périaux J, Knörzer D (eds) Proc. of the 4th European congress on computational methods in applied sciences and engineering (CDROM). ECCOMAS-European Community on Computational Methods in Applied Sciences, Jyväskylä (Finland)

  24. Gómez H, Colominas I, Navarrina F, Casteleiro M (2006) A finite element formulation for a convection-diffusion equation based on Cattaneo’s law. Comput Methods Appl Mech Eng. doi:10.1016/j.cma.2006.09.016

  25. Christov CI, Jordan PM (2005) Heat conduction paradox involving second-sound propagation in moving media. Phys Rev Lett 94:4301–4304

    Article  Google Scholar 

  26. Courant R, Friedrichs KO (1999) Supersonic flow and shock waves. Springer, New York

    Google Scholar 

  27. Reed WH, Hill TR (1973) Triangular mesh methods for the neutron transport equation. Technical Report LA-UR-73-479, Los Alamos Scientific Laboratory

  28. Cockburn B, Karniadakis GE, Shu C-W (eds) (1999) Discontinuous Galerkin methods. Theory, computation and applications. Springer, New York

    Google Scholar 

  29. Cockburn B, Shu C-W (1989) TVB Runge-Kutta local projection discontinuous Galerkin finite element method for scalar conservation laws II: general framework. Math Comput 52:411–435

    Article  MATH  MathSciNet  Google Scholar 

  30. Cockburn B, Lin S-Y, Shu C-W (1989) TVB Runge-Kutta local projection discontinuous Galerkin finite element method for conservation laws III: one-dimensional systems. J Comput Phys 84:90–113

    Article  MATH  MathSciNet  Google Scholar 

  31. Cockburn B, Shu C-W (1998) The Runge-Kutta discontinuous Galerkin finite element method for conservation laws V: multidimensional systems. J Comput Phys 141:199–224

    Article  MATH  MathSciNet  Google Scholar 

  32. Cockburn B, Shu C-W (2001) Runge-Kutta discontinuous Galerkin methods for convection dominated flows. J Sci Comput 16:173–261

    Article  MATH  MathSciNet  Google Scholar 

  33. Bassi F, Rebay S (1997) A high-order accurate discontinuous finite element method for the numerical solution of the compressible Navier-Stokes equations. J Comput Phys 131:267–279

    Article  MATH  MathSciNet  Google Scholar 

  34. Cockburn B, Shu C-W (1998) The local discontinuous Galerkin method for time-dependent convection-diffusion systems. SIAM J Numer Anal 35:2440–2463

    Article  MATH  MathSciNet  Google Scholar 

  35. Baumann CE, Oden JT (1999) A discontinuous hp finite element method for convection-diffusion problems. Comput Methods Appl Mech Eng 175:311–341

    Article  MATH  MathSciNet  Google Scholar 

  36. Baumann CE, Oden JT (1999) A discontinuous hp finite element method for the Euler and the Navier-Stokes equations. Int J Numer Methods Eng 31:79–95

    Article  MATH  MathSciNet  Google Scholar 

  37. Castillo P (2002) Performance of discontinuous Galerkin methods for elliptic PDEs. SIAM J Sci Comput 24:524–547

    Article  MATH  MathSciNet  Google Scholar 

  38. Castillo P, Cockburn B, Perugia I, Schötzau D (2000) An a priori error analysis of the local discontinuous Galerkin method for elliptic problems. SIAM J Numer Anal 38:1676–1706

    Article  MATH  MathSciNet  Google Scholar 

  39. Van Leer B (1982) Flux vector splitting for the Euler equations. Lect Notes Phys 170:507–512

    Article  Google Scholar 

  40. Hänel D, Schwane R, Seider G (1987) On the accuracy of upwind schemes for the resolution of the Navier-Stokes equations, AIAA Paper, 87-1005

  41. Bibb KL, Peraire J, Riley CJ (1997) Hypersonic flow computations on unstructured meshes, AIAA Paper, 97-0625

  42. Gómez H, Colominas I, Navarrina F, Casteleiro M, A discontinuous Galerkin method for a hyperbolic model for convection-diffusion problems in CFD. Int J Numer Methods Eng (accepted)

  43. Gómez H (2006) A hyperbolic formulation for convective-diffusive problems in CFD. PhD dissertation (in Spanish), University of A Coruña

  44. Shu C-W, Osher S (1988) Efficient implementation of essentially non-oscillatory shock-capturing schemes. J Comput Phys 77:439–471

    Article  MATH  MathSciNet  Google Scholar 

  45. Sarrate J, Huerta A (2000) Efficient unstructured quadrilateral mesh generation. Int J Numer Methods Eng 49:1327–1350

    Article  MATH  Google Scholar 

Download references

Acknowledgments

H. Gómez gratefully acknowledges the support provided by Ministerio de Educación y Ciencia through the postdoctoral fellowships program. The authors were partially supported by Xunta de Galicia (grants # PGIDIT05PXIC118002PN and # PGDIT06TAM11801PR), Ministerio de Educación y Ciencia (grants # DPI2004-05156, # DPI2006-15275 and # DPI2007-61214) cofinanced with FEDER funds, Universidad de A Coruña and Fundación de la Ingeniería Civil de Galicia. This funding is gratefully acknowledged.

Author information

Authors and Affiliations

  1. Institute for Computational Engineering and Sciences, The University of Texas at Austin, 1 University Station, C0200, 201 E. 24th Street, Austin, TX, 78712, USA

    Héctor Gómez

  2. Civil Engineering School, Department of Mathematical Methods, University of A Coruña, Campus de Elviña, 15192, A Coruña, Spain

    Héctor Gómez, Ignasi Colominas, Fermín Navarrina & Manuel Casteleiro

Authors
  1. Héctor Gómez
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ignasi Colominas
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Fermín Navarrina
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Manuel Casteleiro
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Ignasi Colominas.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Gómez, H., Colominas, I., Navarrina, F. et al. A mathematical model and a numerical model for hyperbolic mass transport in compressible flows. Heat Mass Transfer 45, 219–226 (2008). https://doi.org/10.1007/s00231-008-0418-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00231-008-0418-0

Keywords

Search

Navigation