Skip to main content
SpringerLink
Log in

A Nonlinear Fluid-Structure Interaction Problem in Compliant Arteries Treated with Vascular Stents

  • Published:
Applied Mathematics & Optimization Submit manuscript

Abstract

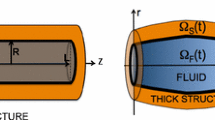
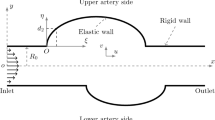
We study a nonlinear fluid-structure interaction problem between an incompressible, viscous fluid in 3D and an elastic structure whose Lamé elastic parameters, thickness and density are all functions of space allowing jump discontinuities. This problem is motivated by studying the interaction between blood flow and arterial walls treated with vascular prostheses called stents. A stent is a metallic mesh-like tube used to prop the clogged arteries open. The Navier–Stokes equations for an incompressible, viscous fluid are used to model blood flow, and the cylindrical Koiter shell equations with discontinuous coefficients are used to model the elastic properties of arterial walls treated with stents. The fluid and structure are coupled via two coupling conditions evaluated at the moving fluid-structure interface. No assumption on axial symmetry is used in the model. We prove the existence of a weak solution to the underlying nonlinear 3D moving-boundary problem, and design a loosely-coupled partitioned scheme (\(\beta \)-scheme) for its solution. The numerical scheme was motivated by the main steps in the constructive existence proof. The existence proof shows that the proposed numerical \(\beta \)-scheme converges to a weak solution of the nonlinear problem. This is the first convergence result for the proposed partitioned \(\beta \)-scheme. Several numerical examples are presented where different stent configurations are considered. The numerical fluid-structure interaction solutions clearly show that the presence of a stent induces wave reflections in arterial walls, and significant flow disturbances, especially near the proximal site of the stent.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11

Similar content being viewed by others

References

  1. Adams, A.R.: Sobolev Spaces. Academic Press [A subsidiary of Harcourt Brace Jovanovich, Publishers], New York, 1975. Pure and Applied Mathematics, Vol. 65

  2. Astorino, M., Chouly, F., Fernández, M.A.: Robin based semi-implicit coupling in fluid-structure interaction. SIAM J. Sci. Comput. 31, 4041–4065 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  3. Badia, S., Nobile, F., Vergara, C.: Fluid-structure partitioned procedures based on Robin transmission conditions. J. Comput. Phys. 227, 7027–7051 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  4. Badia, S., Quaini, A., Quarteroni, A.: Splitting methods based on algebraic factorization for fluid-structure interaction. SIAM J. Sci. Comput. 30(4), 1778–1805 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  5. Banks, J., Henshaw, W., Schwendeman, D.: An analysis of a new stable partitioned algorithm for FSI problems. Part I. J. Comput. Phys. 269, 108–137 (2014)

    Article  MathSciNet  Google Scholar 

  6. Banks, J., Henshaw, W., Schwendeman, D.: An analysis of a new stable partitioned algorithm for FSI problems. Part II. J. Comput. Phys. 268, 399–416 (2014)

    Article  MathSciNet  Google Scholar 

  7. Barbu, V., Grujić, Z., Lasiecka, I., Tuffaha, A.: Existence of the energy-level weak solutions for a nonlinear fluid-structure interaction model. Fluids and Waves, vol. 440. American Mathematical Society, Providence, RI (2007)

    Chapter  Google Scholar 

  8. Barbu, V., Grujić, Z., Lasiecka, I., Tuffaha, A.: Smoothness of weak solutions to a nonlinear fluid-structure interaction model. Indiana Univ. Math. J. 57(3), 1173–1207 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  9. Beirão da Veiga, H.: On the existence of strong solutions to a coupled fluid-structure evolution problem. J. Math. Fluid Mech. 6(1), 21–52 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  10. Bociu, L., Toundykov, D., Zolésio, J.-P.: Well-posedness analysis for a linearization of a fluid-elasticity interaction. SIAM J. Math. Anal. 47(3), 1958–2000 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  11. Bukac, M., Canic, S.: Longitudinal displacement in viscoelastic arteries: a novel fluid-structure interaction computational model, and experimental validation. J. Math. Biosci. Eng. 10(2), 295–318 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  12. Bukac, M., Muha, B.: Stability and convergence analysis of the kinematically coupled scheme and its extensions for the fluid-structure interaction. (2016). arXiv:1601.00664

  13. Bukac, M., Canic, S., Glowinski, R., Tambaca, J., Quaini, A.: Fluid-structure interaction in blood flow capturing non-zero longitudinal structure displacement. J. Comput. Phys. 235, 515–541 (2013)

    Article  MathSciNet  Google Scholar 

  14. Bukac, M., Canic, S., Muha, B.: A partitioned scheme for fluid-composite structure interaction problems. J. Comput. Phys. 281, 493–517 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  15. Bukač, M., Yotov, I., Zunino, P.: An operator splitting approach for the interaction between a fluid and a multilayered poroelastic structure. Numer. Methods Partial Differ. Equ. 31(4), 1054–1100 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  16. Čanić, S., Muha, B., Bukač, M.: Fluid-structure interaction in hemodynamics: modeling, analysis, and numerical simulation. In Fluid-Structure Interaction and Biomedical Applications, pp. 79–195. Springer Basel, Basel (2014)

  17. Čanić, S., Muha, B., Bukač, M.: Stability of the kinematically coupled \(\beta \)-scheme for fluid-structure interaction problems in hemodynamics. Int. J. Numer. Anal. Model. 12(1), 54–80 (2015)

    MathSciNet  MATH  Google Scholar 

  18. Canic, S., Tambača, J.: Cardiovascular stents as PDE nets: 1D vs. 3D. IMA J. Appl. Math. 77(6), 748–770 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  19. Causin, P., Gerbeau, J.F., Nobile, F.: Added-mass effect in the design of partitioned algorithms for fluid-structure problems. Comput. Methods Appl. Mech. Eng. 194(42–44), 4506–4527 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  20. Chacon-Rebollo, T., Girault, V., Murat, F., Pironneau, O.: Analysis of a simplified coupled fluid-structure model for computational hemodynamics. SIAM J. Numer. Anal. To appear

  21. Chambolle, A., Desjardins, B., Esteban, M.J., Grandmont, C.: Existence of weak solutions for the unsteady interaction of a viscous fluid with an elastic plate. J. Math. Fluid Mech. 7(3), 368–404 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  22. Cheng, C.H.A., Coutand, D., Shkoller, S.: Navier–Stokes equations interacting with a nonlinear elastic biofluid shell. SIAM J. Math. Anal. 39(3), 742–800 (electronic) (2007)

  23. Cheng, C.H.A., Shkoller, S.: The interaction of the 3D Navier–Stokes equations with a moving nonlinear Koiter elastic shell. SIAM J. Math. Anal. 42(3), 1094–1155 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  24. Ciarlet, C.R., Roquefort, A.: Justification of a two-dimensional shell model of koiter type. C.R. Acad. Sci. Paris Ser I Math. 331(5), 411–416 (2000)

  25. Ciarlet, P.G.: A two-dimensional nonlinear shell model of koiter type. C.R. Acad. Sci. Paris Ser I Math. 331, 405–410 (2000)

  26. Ciarlet, C.H., Coutand, D.: An existence theorem for nonlinearly elastic “flexural” shells. J. Elast. 50(3), 261–277 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  27. Conca, C., Murat, F., Pironneau, O.: The Stokes and Navier–Stokes equations with boundary conditions involving the pressure. Jpn. J. Math. (N.S.) 20(2), 279–318 (1994)

  28. Coutand, D., Shkoller, S.: Motion of an elastic solid inside an incompressible viscous fluid. Arch. Rational Mech. Anal. 176(1), 25–102 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  29. Coutand, D., Shkoller, S.: The interaction between quasilinear elastodynamics and the Navier–Stokes equations. Arch. Rational Mech. Anal. 179(3), 303–352 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  30. Deparis, S., Fernandez, M., Formaggia, L.: Acceleration of a fixed point algorithm for a fluid-structure interaction using transpiration condition. Math. Model. Numer. Anal. 37(4), 601–616 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  31. Deparis, S., Discacciati, M., Fourestey, G., Quarteroni, A.: Fluid-structure algorithms based on Steklov–Poincaré operators. Comput. Methods Appl. Mech. Eng. 195(41–43), 5797–5812 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  32. Donea, J.: Arbitrary lagrangian-eulerian finite element methods. Comput. Methods Transient Anal. pp. 473–516 (1983)

  33. Du, Q., Gunzburger, M.D., Hou, L.S., Lee, J.: Analysis of a linear fluid-structure interaction problem. Discret. Contin. Dyn. Syst. 9(3), 633–650 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  34. Farhat, C., Geuzaine, P., Grandmont, C.: The discrete geometric conservation law and the nonlinear stability of ale schemes for the solution of flow problems on moving grids. J. Comput. Phys. 174(2), 669–694 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  35. Fernández, M.A.: Incremental displacement-correction schemes for incompressible fluid-structure interaction. Numer. Math. 123(1), 21–65 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  36. Fernández, M.A., Gerbeau, J.F., Grandmont, C.: A projection algorithm for fluid-structure interaction problems with strong added-mass effect. C. R. Math. 342(4), 279–284 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  37. Figueroa, C., Vignon-Clementel, I., Jansen, K., Hughes, T., Taylor, C.: A coupled momentum method for modeling blood flow in three-dimensional deformable arteries. Comput. Methods Appl. Mech. Eng. 195(41–43), 5685–5706 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  38. Formaggia, L., Gerbeau, J.F., Nobile, F., Quarteroni, A.: On the coupling of 3d and 1d navier-stokes equations for flow problems in compliant vessels. Comput. Methods Appl. Mech. Eng. 191(6–7), 561–582 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  39. Freefem++. Université Pierre et Marie Curie, Laboratoire Jacques-Louis Lions. http://www.freefem.org/ff++/

  40. Glowinski, R.: Finite element methods for incompressible viscous flow. In: Ciarlet, P.G., Lions, J.-L. (eds.) Handbook of Numerical Analysis, vol. 9. Elsevier, New York (2003)

    Google Scholar 

  41. Grandmont, C., Hillairet, M.: Existence of global strong solutions to a beam-fluid interaction system. (2015). arXiv:1504.00830

  42. Grandmont, C.: Existence of weak solutions for the unsteady interaction of a viscous fluid with an elastic plate. SIAM J. Math. Anal. 40(2), 716–737 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  43. Guidoboni, G., Glowinski, R., Cavallini, N., Canic, S.: Stable loosely-coupled-type algorithm for fluid-structure interaction in blood flow. J. Comput. Phys. 228(18), 6916–6937 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  44. Guidoboni, G., Guidorzi, M., Padula, M.: Continuous dependence on initial data in fluid-structure motions. J. Math. Fluid Mech. 14(1), 1–32 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  45. Hundertmark-Zaušková, A., Lukáčová-Medvidová, M., Rusnáková, G.: Fluid-structure interaction for shear-dependent non-Newtonian fluids. In Topics in Mathematical Modeling and Analysis. Jind\(\breve{{\rm r}}\)ich Ne\(\breve{{\rm a}}\)as Center for Mathematical Modeling Lecture Notes, vol. 7, pp. 109–158. Matfyzpress, Prague (2012)

  46. Hundertmark-Zaušková, A., Lukáčová-Medvid’ová, M., Rusnáková, G.: Kinematic splitting algorithm for fluidn++structure interaction in hemodynamics. Comput. Methods Appl. Mech. Eng. (2013). To appear

  47. Ignatova, M., Kukavica, I., Lasiecka, I., Tuffaha. A.: On well-posedness for a free boundary fluid-structure model. J. Math. Phys. 53(11), 115624 (2012)

  48. Ignatova, M., Kukavica, I., Lasiecka, I., Tuffaha, A.: On well-posedness and small data global existence for an interface damped free boundary fluid-structure model. Nonlinearity 27(3), 467 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  49. Koiter, W.T.: On the foundations of the linear theory of thin elastic shells. i, ii. Proc. K. Ned. Akad. Wet. Ser. B, 73(3), 169 (1970)

  50. Kukavica, I., Tuffaha, A., Ziane, M.: Strong solutions for a fluid structure interaction system. Adv. Differ. Equ. 15(3–4), 231–254 (2010)

    MathSciNet  MATH  Google Scholar 

  51. Kukavica, I., Tuffaha, A.: Solutions to a fluid-structure interaction free boundary problem. DCDS-A 32(4), 1355–1389 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  52. Kukavica, I., Tuffaha, A.: Solutions to a free boundary problem of fluid-structure interaction. Indiana Univ. Math. J. 61, 1817–1859 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  53. Kukavica, I., Tuffaha, A.: Well-posedness for the compressible Navier–Stokes–Lamé system with a free interface. Nonlinearity 25(11), 3111–3137 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  54. Lengeler, D.: Weak solutions for an incompressible, generalized Newtonian fluid interacting with a linearly elastic Koiter type shell. SIAM J. Math. Anal. 46(4), 2614–2649 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  55. Lengeler, D., Ružička, M.: Weak solutions for an incompressible Newtonian fluid interacting with a Koiter type shell. Arch. Rational Mech. Anal. 211(1), 205–255 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  56. Lequeurre, J.: Existence of strong solutions to a fluid-structure system. SIAM J. Math. Anal. 43(1), 389–410 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  57. Lequeurre, J.: Existence of strong solutions for a system coupling the Navier–Stokes equations and a damped wave equation. J. Math. Fluid Mech. 15(2), 249–271 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  58. Muha, B.: A note on the trace theorem for domains which are locally subgraph of a Hölder continuous function. Netw. Heterog. Media 9(1), 191–196 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  59. Muha, B., Čanić, S.: A nonlinear, 3d fluid-structure interaction problem driven by the time-dependent dynamic pressure data: a constructive existence proof. Commun. Inf. Syst. 13(3), 357–397 (2013)

    MathSciNet  MATH  Google Scholar 

  60. Muha, B., Čanić, S.: Existence of a weak solution to a nonlinear fluid-structure interaction problem modeling the flow of an incompressible, viscous fluid in a cylinder with deformable walls. Arch. Rational Mech. Anal. 207(3), 919–968 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  61. Muha, B., Čanić, S.: Existence of a solution to a fluid-multi-layered-structure interaction problem. J. Differ. Equ. 256(2), 658–706 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  62. Muha, B., Čanić, S.: Fluid-structure interaction between an incompressible, viscous 3D fluid and an elastic shell with nonlinear Koiter membrane energy. Interfaces Free Bound. 17(4), 465–495 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  63. Muha, B., Čanić, S.: Existence of a weak solution to a fluid-elastic structure interaction problem with the Navier slip boundary condition. J. Differ. Equations (2016). doi:10.1016/j.jde.2016.02.029

  64. Murea, C.M., Sy, S.: A fast method for solving fluid-structure interaction problems numerically. Int. J. Numer. Methods Fluids 60(10), 1149–1172 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  65. Nobile, F., Vergara, C.: An effective fluid-structure interaction formulation for vascular dynamics by generalized Robin conditions. SIAM J. Sci. Comput. 30, 731–763 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  66. Quarteroni, A., Tuveri, M., Veneziani, A.: Computational vascular fluid dynamics: problems, models and methods. survey article. Comput. Visual Sci. 2, 163–197 (2000)

    Article  MATH  Google Scholar 

  67. Raymond, J.-P., Vanninathan, M.: A fluid-structure model coupling the navier-stokes equations and the lamé system. J. Math. Pure Appl. 102(3), 546–596 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  68. Simon, J.: Compact sets in the space \(L^p(0, T;B)\). Ann. Mat. Pura Appl. 4(146), 65–96 (1987)

    MathSciNet  MATH  Google Scholar 

  69. Tambača, J., Kosor, M., Čanić, S., Paniagua, D.: Mathematical modeling of vascular stents. SIAM J. Appl. Math. 70(6), 1922–1952 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  70. Temam, R.: Sur la résolution exacte et approchée d’un problème hyperbolique non linéaire de T. Carleman Arch. Rational Mech. Anal. 35, 351–362 (1969)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgments

The authors would like to thank Frédéric Hecht and Olivier Pironneau for their help with FreeFem++. Special thanks are extended to the funding agencies: US National Science Foundation (Grants DMS-1318763 (Canic and Bukac), DMS-1311709 (Canic and Muha), and DMS-1262385 (Canic)), the Croatian Science Foundation (Hrvatska Zaklada za Znanost; Grant Number 9477), and the UH Cullen Chair Funds (Canic).

Author information

Authors and Affiliations

  1. University of Notre Dame, Notre Dame, IN, USA

    Martina Bukač

  2. University of Houston, Houston, TX, USA

    Sunčica Čanić

  3. University of Zagreb, Zagreb, Croatia

    Boris Muha

Authors
  1. Martina Bukač
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Sunčica Čanić
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Boris Muha
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Martina Bukač.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Bukač, M., Čanić, S. & Muha, B. A Nonlinear Fluid-Structure Interaction Problem in Compliant Arteries Treated with Vascular Stents. Appl Math Optim 73, 433–473 (2016). https://doi.org/10.1007/s00245-016-9343-7

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00245-016-9343-7

Keywords

Mathematics Subject Classification

Search

Navigation