A BME solution of the stochastic three-dimensional Laplace equation representing a geothermal field subject to site-specific information

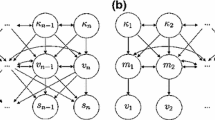
Abstract

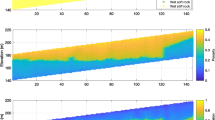
This work develops a model of the geothermal field in the Nea Kessani region (Greece) by means of the Bayesian maximum entropy (BME) method, which describes the temperature variations across space in the underground geological formations. The geothermal field is formed by a thermal reservoir consisting of arcosic sandstones. The temperature distribution vs depth was first investigated by the Greek Institute of Geology and Mineral Exploration (IGME) using measurements in a set of vertical drill holes. These measurements showed that hot fluids rising from the deep enter the reservoir in a restricted area of the field and flow towards local thermal springs. The field modelling, which was based on the powerful BME concept, involves the solution of a stochastic partial differential equation that assimilates important site-specific information. The stochastic three-dimensional steady-state Laplace equation was considered as general knowledge and the drilling exploration data were used to construct the specificatory knowledge base in the BME terminology. The produced map is more informative and, in general, it gives higher temperature estimates compared to previous studies of the same region. This is also in agreement with the quartz geothermometry analysis carried out by IGME.

Appendices

Appendix 1

By applying the mean expectation operator on Eq. 6 we get:

$$ \begin{aligned} \overline{T} ({\mathbf{s}}_{i} ) = & \overline{{A_{1} f_{1} ({\mathbf{s}}_{i} ) + A_{2} f_{2} ({\mathbf{s}}_{i} ) + A_{3} f_{3} ({\mathbf{s}}_{i} ) + A_{4} f_{4} ({\mathbf{s}}_{i} ) + A_{4} f_{4} ({\mathbf{s}}_{i} ) + A_{6} f_{6} ({\mathbf{s}}_{i} )}} \\ = & m_{1} f_{1} ({\mathbf{s}}_{i} ) + m_{2} f_{2} ({\mathbf{s}}_{i} ) + m_{3} f_{3} ({\mathbf{s}}_{i} ) + m_{4} f_{4} ({\mathbf{s}}_{i} ) + m_{5} f_{5} ({\mathbf{s}}_{i} ) + m_{6} f_{6} ({\mathbf{s}}_{i} ) \\ \end{aligned} $$

$$ \begin{aligned} \sigma ^{2}_{i} = & E[T({\mathbf{s}}_{i} )^{2} ] - \{ E[T({\mathbf{s}}_{i} )]\} ^{2} \\ = & \overline{{[A_{1} f_{1} ({\mathbf{s}}_{i} ) + A_{2} f_{2} ({\mathbf{s}}_{i} ) + A_{3} f_{3} ({\mathbf{s}}_{i} ) + A_{4} f_{4} ({\mathbf{s}}_{i} ) + A_{5} f_{5} ({\mathbf{s}}_{i} ) + A_{6} f_{6} ({\mathbf{s}}_{i} )]^{2} }} \\ - & [\overline{{A_{1} f_{1} ({\mathbf{s}}_{i} ) + A_{2} f_{2} ({\mathbf{s}}_{i} ) + A_{3} f_{3} ({\mathbf{s}}_{i} ) + A_{4} f_{4} ({\mathbf{s}}_{i} ) + A_{5} f_{5} ({\mathbf{s}}_{i} ) + A_{6} f_{6} ({\mathbf{s}}_{i} )}} ]^{2} \\ \end{aligned} $$

Expanding the quadratics in the above expression we finally get

$$ \begin{aligned} & (\overline{{A_{1} ^{2}}} - m^{2}_{1} )f_{1} ^{2} ({\mathbf{s}}_{i} ) + (\overline{{A_{2} ^{2}}} - m^{2}_{2} )f_{2} ^{2} ({\mathbf{s}}_{i} ) + (\overline{{A_{3} ^{2}}} - m^{2}_{3} )f_{3} ^{2} ({\mathbf{s}}_{i} ) + (\overline{{A_{4} ^{2}}} - m^{2}_{4} )f_{4} ^{2} ({\mathbf{s}}_{i} ) + (\overline{{A_{5} ^{2}}} - m^{2}_{5} )f_{5} ^{2} ({\mathbf{s}}_{i} ) \\ & + (\overline{{A_{6} ^{2}}} - m^{2}_{6} )f_{6} ^{2} ({\mathbf{s}}_{i} ) \\ & = \sigma ^{2}_{1} f_{1} ^{2} ({\mathbf{s}}_{i} ) + \sigma ^{2}_{2} f_{2} ^{2} ({\mathbf{s}}_{i} ) + \sigma ^{2}_{3} f_{3} ^{2} ({\mathbf{s}}_{i} ) + \sigma ^{2}_{4} f_{4} ^{2} ({\mathbf{s}}_{i} ) + \sigma ^{2}_{5} f_{5} ^{2} ({\mathbf{s}}_{i} ) + \sigma ^{2}_{6} f_{6} ^{2} ({\mathbf{s}}_{i} ) \\ \end{aligned} $$

$$ \begin{aligned} c_{{i,j}} = & E\{ [T({\mathbf{s}}_{i} ) - \overline{T} ({\mathbf{s}}_{i} )][T({\mathbf{s}}_{j} ) - \overline{T} ({\mathbf{s}}_{j} )]\} = E\{ T({\mathbf{s}}_{i} )T({\mathbf{s}}_{j} )\} - E\{ T({\mathbf{s}}_{i} )\} E\{ T({\mathbf{s}}_{j} )\} \\ = & \sigma ^{2}_{1} f_{1} ({\mathbf{s}}_{i} )f_{1} ({\mathbf{s}}_{j} ) + \sigma ^{2}_{2} f_{2} ({\mathbf{s}}_{i} )f_{2} ({\mathbf{s}}_{j} ) + \sigma ^{2}_{3} f_{3} ({\mathbf{s}}_{i} )f_{3} ({\mathbf{s}}_{j} ) \\ & \quad + \sigma ^{2}_{4} f_{4} ({\mathbf{s}}_{i} )f_{4} ({\mathbf{s}}_{j} ) + \sigma ^{2}_{5} f_{5} ({\mathbf{s}}_{i} )f_{5} ({\mathbf{s}}_{j} ) + \sigma ^{2}_{6} f_{6} ({\mathbf{s}}_{i} )f_{6} ({\mathbf{s}}_{j} ) \\ \end{aligned} $$

According to Papoulis and Pillai (2002), the joint density for n normal variables that have zero mean is given by

$$ f({\mathbf{X}}) = \frac{1} {{{\sqrt {(2\pi )^{n} \Delta}}}}\exp {\left\{{- \frac{1} {2}{\mathbf{X}}^{{\text{T}}} {\mathbf{C}}^{{- 1}} {\mathbf{X}}} \right\}} $$

(16)

where $X = \left({\begin{array}{*{20}c} {x_1} \\ \vdots \\ {x_n} \\ \end{array}} \right)$is the random vector, X ^T is the transpose vector, $C = \left({\begin{array}{*{20}c} {c_{11}}& \ldots& {c_{1n}} \\ \vdots& \ddots& \vdots \\ {c_{n1}}& \cdots& {c_{nn}} \\ \end{array}} \right)$ is the covariance matrix and Δ is the determinant of C. In case of two independent normal variables, x and y, with zero mean the exponent of Eq. 16 is

$$\left[{\frac{{x^2 \sigma _y^2 + y^2 \sigma _x^2 - 2xyr_{xy}}}{{2(r_{xy}^2 - 1)\sigma _x^2 \sigma _y^2}}} \right]$$

(17)

where r _xy is the correlation coefficient. For three normal variables x,y and z, the exponent of Eq. 16 becomes

$$\left[{\frac{{(r_{xy}^2 - 1)\left({z - \frac{{x\sigma _z (r_{xy} r_{yz} - r_{xz})}}{{(r_{xy}^2 - 1)\sigma _x}} - \frac{{y\sigma _z (r_{xy} r_{xz} - r_{yz})}}{{(r_{xy}^2 - 1)\sigma _y}}} \right)^2}}{{2(- 1 + r_{xy}^2 + r_{xz}^2 - 2r_{xy} r_{xz} r_{yz} + r_{yz}^2)\sigma _z^2}} + \frac{{x^2 \sigma _y^2 + y^2 \sigma _x^2 - 2xyr_{xy}}}{{2(r_{xy}^2 - 1)\sigma _x^2 \sigma _y^2}}} \right]$$

(18)

Appendix 2

The conditional density of three normal variables with zero mean is

$$ f(z|x,y) = \frac{1} {{{\sqrt {2\pi P} }}}{\text{e}}^{{ - (z - hx - wy)^{2} /2P}} $$

(19)

We also know that

$$f(z|x,y) = \frac{{f(x,y,z)}}{{f(x,y)}}$$

(20)

Working only with the exponential parts and working in terms of Eqs. 17, 18 and 20 we find

$$ \begin{array}{*{20}l} {{{\left[ {\frac{{(r^{2}_{{xy}} - 1){\left( {z - \frac{{x\sigma _{z} (r_{{xy}} r_{{yz}} - r_{{xz}} )}} {{(r^{2}_{{xy}} - 1)\sigma _{x} }} - \frac{{y\sigma _{z} (r_{{xy}} r_{{xz}} - r_{{yz}} )}} {{(r^{2}_{{xy}} - 1)\sigma _{y} }}} \right)}^{2} }} {{2( - 1 + r^{2}_{{xy}} + r^{2}_{{xz}} - 2r_{{xy}} r_{{xz}} r_{{yz}} + r^{2}_{{yz}} )\sigma ^{2}_{z} }} + \frac{{x^{2} \sigma ^{2}_{y} + y^{2} \sigma ^{2}_{x} - 2xyr_{{xy}} }} {{2(r^{2}_{{xy}} - 1)\sigma ^{2}_{x} \sigma ^{2}_{y} }}} \right]}/\frac{{x^{2} \sigma ^{2}_{y} + y^{2} \sigma ^{2}_{x} - 2xyr_{{xy}} }} {{2(r^{2}_{{xy}} - 1)\sigma ^{2}_{x} \sigma ^{2}_{y} }}} \hfill} \\ {{ = \frac{{(r^{2}_{{xy}} - 1){\left( {z - \frac{{x\sigma _{z} (r_{{xy}} r_{{yz}} - r_{{xz}} )}} {{(r^{2}_{{xy}} - 1)\sigma _{x} }} - \frac{{y\sigma _{z} (r_{{xy}} r_{{xz}} - r_{{yz}} )}} {{(r^{2}_{{xy}} - 1)\sigma _{y} }}} \right)}^{2} }} {{2( - 1 + r^{2}_{{xy}} + r^{2}_{{xz}} - 2r_{{xy}} r_{{xz}} r_{{yz}} + r^{2}_{{yz}} )\sigma ^{2}_{z} }} + \frac{{x^{2} \sigma ^{2}_{y} + y^{2} \sigma ^{2}_{x} - 2xyr_{{xy}} }} {{2(r^{2}_{{xy}} - 1)\sigma ^{2}_{x} \sigma ^{2}_{y} }} - \frac{{x^{2} \sigma ^{2}_{y} + y^{2} \sigma ^{2}_{x} - 2xyr_{{xy}} }} {{2(r^{2}_{{xy}} - 1)\sigma ^{2}_{x} \sigma ^{2}_{y} }}} \hfill} \\ {{ = \frac{{(r^{2}_{{xy}} - 1){\left( {z - \frac{{x\sigma _{z} (r_{{xy}} r_{{yz}} - r_{{xz}} )}} {{(r^{2}_{{xy}} - 1)\sigma _{x} }} - \frac{{y\sigma _{z} (r_{{xy}} r_{{xz}} - r_{{yz}} )}} {{(r^{2}_{{xy}} - 1)\sigma _{y} }}} \right)}^{2} }} {{2( - 1 + r^{2}_{{xy}} + r^{2}_{{xz}} - 2r_{{xy}} r_{{xz}} r_{{yz}} + r^{2}_{{yz}} )\sigma ^{2}_{z} }}} \hfill} \\ {{ = \frac{{{\left( {z - \frac{{x\sigma _{z} (r_{{xy}} r_{{yz}} - r_{{xz}} )}} {{(r^{2}_{{xy}} - 1)\sigma _{x} }} - \frac{{y\sigma _{z} (r_{{xy}} r_{{xz}} - r_{{yz}} )}} {{(r^{2}_{{xy}} - 1)\sigma _{y} }}} \right)}^{2} }} {{2( - 1 + r^{2}_{{xy}} + r^{2}_{{xz}} - 2r_{{xy}} r_{{xz}} r_{{yz}} + r^{2}_{{yz}} )\sigma ^{2}_{z} /(r^{2}_{{xy}} - 1)}}} \hfill} \\ \end{array} $$

which is the exponent of f(z|x,y). By comparison with Eq. 19 we get

$$P = ( - 1 + r_{xy}^2 + r_{xz}^2 - 2r_{xy} r_{xz} r_{yz} + r_{yz}^2 )\sigma _z^2 /(r_{xy}^2 - 1),\quad h = \frac{{\sigma _z (r_{xy} r_{yz} - r_{xz} )}}{{(r_{xy}^2 - 1)\sigma _x}},\quad w = \frac{{\sigma _z (r_{xy} r_{xz} - r_{yz} )}}{{(r_{xy}^2 - 1)\sigma _y}}$$

which define Eq. 14. In order to derive Eq. 15 we have to substitute the random variables in the exponent of f(z|x,y) with ones having non-zero mean values. More specifically, if x= T(s _i ), y= T(s _j ) and z = T(s _k ) are random variables with normal joint density and $\overline{x} = E[(T({\varvec {s}}_{i} )] = \overline{T} ({\varvec {s}}_{i} ),\quad \overline{y} = E[(T({\varvec {s}}_{j} )] = \overline{T} ({\varvec {s}}_{j} ),\quad \overline{z} = E[(T({\varvec {s}}_{k} )] = \overline{T} ({\varvec {s}}_{k} ),$ then f(z|x,y) is a normal density with exponent $\frac{{{\left( {(z - \overline{z} ) - \frac{{(x - \overline{x} )\sigma _{z} (r_{{xy}} r_{{yz}} - r_{{xz}} )}} {{(r^{2}_{{xy}} - 1)\sigma _{x}}} - \frac{{(y - \overline{y} )\sigma _{z} (r_{{xy}} r_{{xz}} - r_{{yz}} )}} {{(r^{2}_{{xy}} - 1)\sigma _{y}}}} \right)}^{2}}} {{2( - 1 + r^{2}_{{xy}} + r^{2}_{{xz}} - 2r_{{xy}} r_{{xz}} r_{{yz}} + r^{2}_{{yz}} )\sigma ^{2}_{z} /(r^{2}_{{xy}} - 1)}},$ and the mean value is given by $E\{{\mathbf{z}}|x,y\} = z + \frac{{(x - \overline{x} )\sigma _{z} (r_{{xy}} r_{{yz}} - r_{{xz}} )}} {{(r^{2}_{{xy}} - 1)\sigma _{x}}} + \frac{{(y - \overline{y} )\sigma _{z} (r_{{xy}} r_{{xz}} - r_{{yz}} )}} {{(r^{2}_{{xy}} - 1)\sigma _{y}}},$ or $\overline T ({\mathbf{s}}_k) = T({\mathbf{s}}_k) + \frac{{(T({\mathbf{s}}_i) - \overline T ({\mathbf{s}}_i))\sigma _k (r_{i,j} r_{j,k} - r_{i,k})}}{{(r_{i,j}^2 - 1)\sigma _i}} + \frac{{(T({\mathbf{s}}_j) - \overline T ({\mathbf{s}}_j))\sigma _k (r_{i,j} r_{i,k} - r_{j,k})}}{{(r_{i,j}^2 - 1)\sigma _j}}$ which is Eq. 15.