Skip to main content
SpringerLink
Log in

A phase-field model for fracture in biological tissues

  • Original Paper
  • Published:
Biomechanics and Modeling in Mechanobiology Aims and scope Submit manuscript

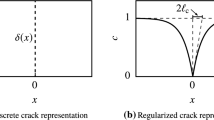
Abstract

This work presents a recently developed phase-field model of fracture equipped with anisotropic crack driving force to model failure phenomena in soft biological tissues at finite deformations. The phase-field models present a promising and innovative approach to thermodynamically consistent modeling of fracture, applicable to both rate-dependent or rate-independent brittle and ductile failure modes. One key advantage of diffusive crack modeling lies in predicting the complex crack topologies where methods with sharp crack discontinuities are known to suffer. The starting point is the derivation of a regularized crack surface functional that \({\varGamma }\)-converges to a sharp crack topology for vanishing length-scale parameter. A constitutive balance equation of this functional governs the crack phase-field evolution in a modular format in terms of a crack driving state function. This allows flexibility to introduce alternative stress-based failure criteria, e.g., isotropic or anisotropic, whose maximum value from the deformation history drives the irreversible crack phase field. The resulting multi-field problem is solved by a robust operator split scheme that successively updates a history field, the crack phase field and finally the displacement field in a typical time step. For the representative numerical simulations, a hyperelastic anisotropic free energy, typical to incompressible soft biological tissues, is used which degrades with evolving phase field as a result of coupled constitutive setup. A quantitative comparison with experimental data is provided for verification of the proposed methodology.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17

Similar content being viewed by others

Notes

  1. Generalized Ginzburg–Landau Equation. Equation (6) was defined in Miehe et al. (2010b) as a generalized Ginzburg–Landau equation for the phase-field evolution, when it is represented

    figure a

    in terms of the variational derivative of a total energy density function \(\widehat{\psi }_{\text {tot}}\) by the phase field d. In contrast to classical phase-field equations as reviewed in Gurtin (1996), the above equation accounts for the irreversibility due to the Macauley bracket. The total energy must contain contributions due to the degrading bulk response and the regularized fracture surface energy. A simple example for finite elasticity at fracture is

    figure b

    where the parameter \(g_c\) is a critical energy release rate. For this ansatz, (7) results in the evolution Eq. (6) with the crack driving state function

    figure c

    that contains the nominal energy \(\widetilde{\psi }({\varvec{ F }})\) of the undamaged material. In this variationally consistent setting, the crack driving state function is derived from a total energy, see Miehe et al. (2014) for more advanced definitions. In contrast, when starting from (2) and using (4), the definition of the crack driving is state function \(\widetilde{D}\) is not constrained to be related to a variational derivative of an energy. This allows the incorporation of the convenient stress-based function outlined in (19) below.

References

  • Alastrué V, Martínez VA, Doblaré M, Menzel A (2009) Anisotropic micro-sphere-based finite elasticity applied to blood vessel modelling. J Mech Phys Solids 57:178–203

    Article  MATH  Google Scholar 

  • Ambrosio L, Tortorelli VM (1990) Approximation of functionals depending on jumps by elliptic functionals via \(\gamma \)-convergence. Commun Pure Appl Math 43:999–1036

    Article  MathSciNet  MATH  Google Scholar 

  • Arruda EM, Boyce MC (1993) A three-dimensional constitutive model for the large stretch behavior of rubber elastic materials. J Mech Phys Solids 41:389–412

    Article  Google Scholar 

  • Balzani D, Neff P, Schröder J, Holzapfel GA (2006) A polyconvex framework for soft biological tissues. Adjustment to experimental data. Int J Solids Struct 43:6052–6070

    Article  MathSciNet  MATH  Google Scholar 

  • Balzani D, Brinkhues S, Holzapfel GA (2012) Constitutive framework for the modeling of damage in collagenous soft tissues with application to arterial walls. Comput Methods Appl Mech Eng 213–216:139–151

    Article  MathSciNet  MATH  Google Scholar 

  • Belytschko T, Black T (1999) Elastic crack growth in finite elements with minimal remeshing. Int J Numer Methods Eng 45:601–620

    Article  MathSciNet  MATH  Google Scholar 

  • Billiar KL, Sacks MS (2000) Biaxial mechanical properties of the native and glutaraldehyde-treated aortic valve cusp : part ii—a structural constitutive model. J Biomech Eng 122:327–335

    Article  Google Scholar 

  • Bischoff JE, Arruda EM, Grosh K (2002) A microstructurally based orthotropic hyperelastic constitutive law. J Appl Mech 69:570–579

    Article  MATH  Google Scholar 

  • Borden MJ, Hughes TJR, Landis CM, Verhoosel CV (2014) A higher-order phase-field model for brittle fracture: formulation and analysis within the isogeometric analysis framework. Comput Methods Appl Mech Eng 273:100–118

    Article  MathSciNet  MATH  Google Scholar 

  • Bourdin B, Francfort GA, Marigo JJ (2000) Numerical experiments in revisited brittle fracture. J Mech Phys Solids 48:797–826

    Article  MathSciNet  MATH  Google Scholar 

  • Bourdin B, Francfort GA, Marigo JJ (2008) Special invited exposition: the variational approach to fracture. J Elast 91:5–148

    Article  MathSciNet  MATH  Google Scholar 

  • Braides DP (1998) Approximation of free discontinuity problems. Springer, Berlin

    MATH  Google Scholar 

  • Braides DP (2002) \(\Gamma \)-convergence for beginners. Oxford University Press, New York

    Book  MATH  Google Scholar 

  • Buliga M (1999) Energy minimizing brittle crack propagation. J Elast 52:201–238

    Article  MathSciNet  MATH  Google Scholar 

  • Capriz G (1989) Continua with microstructure. Springer, Berlin

    Book  MATH  Google Scholar 

  • Castaneda-Zuniga WR, Formanek A, Tadavarthy M, Vlodaver Z, Edwards J, Zollikofer C, Amplatz K (1980) The mechanism of balloon angioplasty. Radiology 135:565–571

    Article  Google Scholar 

  • Chuong C, Fung Y (1984) Compressibility and constitutive equation of arterial wall in radial compression experiments. J Biomech 17:35–40

    Article  Google Scholar 

  • Dal Maso G (1993) An introduction to \(\Gamma \)-convergence. Birkhäuser, Boston

    Book  MATH  Google Scholar 

  • Dal Maso G, Toader R (2002) A model for the quasistatic growth of brittle fractures: existence and approximation results. Arch Ration Mech Anal 162:101–135

    Article  MathSciNet  MATH  Google Scholar 

  • Delfino A, Stergiopulos N, Moore J, Meister JJ (1997) Residual strain effects on the stress field in a thick wall finite element model of the human carotid bifurcation. J Biomech 30:777–786

    Article  Google Scholar 

  • Demiray H (1972) A note on the elasticity of soft biological tissues. J Biomech 5(3):309–311

    Article  Google Scholar 

  • Ferrara A, Pandolfi A (2008) Numerical modelling of fracture in human arteries. Comput Methods Biomech Biomed Eng 11:553–567

    Article  Google Scholar 

  • Ferrara A, Pandolfi A (2010) A numerical study of arterial media dissection processes. Int J Fract 166:21–33

    Article  MathSciNet  MATH  Google Scholar 

  • Flory PJ (1969) Statistical mechanics of chain molecules. Wiley, Chichester-New York

    Google Scholar 

  • Flory PJ, Rehner J (1943) Statistical mechanics of cross-linked polymer networks: I. rubberlike elasticity. J Chem Phys 11:512–520

    Article  Google Scholar 

  • Francfort GA, Marigo JJ (1998) Revisiting brittle fracture as an energy minimization problem. J Mech Phys Solids 46:1319–1342

    Article  MathSciNet  MATH  Google Scholar 

  • Frémond M (2002) Non-smooth thermomechanics. Springer, Berlin

    Book  MATH  Google Scholar 

  • Frémond M, Nedjar B (1996) Damage, gradient of damage and principle of virtual power. Int J Solids Struct 92:178–192

    MathSciNet  MATH  Google Scholar 

  • Fung YC, Fronek K, Patitucci P (1979) Pseudoelasticity of arteries and the choice of its mathematical expression. Am J Physiol 237:H620–H631

    Google Scholar 

  • Gasser TC, Holzapfel GA (2006) Modeling the propagation of arterial dissection. Eur J Mech A Solids 25:617–633

    Article  MathSciNet  MATH  Google Scholar 

  • Gasser TC, Holzapfel GA (2007) Finite element modeling of balloon angioplasty by considering overstretch of remnant non-diseased tissues in lesions. Comput Mech 40:47–60

    Article  MATH  Google Scholar 

  • Gasser TC, Ogden RW, Holzapfel GA (2006) Hyperelastic modelling of arterial layers with distributed collagen fibre orientations. J R Soc Interface 3:15–35

    Article  Google Scholar 

  • Gelsea K, Pöschl E, Aignera T (2003) Collagens-structure, function, and biosynthesis. Adv Drug Deliv Rev 55:1531–1546

    Article  Google Scholar 

  • Gertz SD, Roberts WC (1990) Hemodynamic shear force in rupture of coronary arterial atherosclerotic plaques. Am J Physiol 66:1368–1372

    Google Scholar 

  • Govindjee S, Simo JC (1991) A micro-mechanically based continuum damage model for carbon black-filled rubbers incorporating mullins effect. J Mech Phys Solids 39:87–112

    Article  MathSciNet  MATH  Google Scholar 

  • Gundiah N, Ratcliffe MB, Pruitt LA (2007) Determination of strain energy function for arterial elastin: experiments using histology and mechanical tests. J Biomech 70:586–594

    Article  Google Scholar 

  • Gürses E, Miehe C (2009) A computational framework of three dimensional configurational force driven brittle crack propagation. Comput Methods Appl Mech Eng 198:1413–1428

    Article  MATH  Google Scholar 

  • Gurtin ME (1996) Generalized Ginzburg-Landau and Cahn-Hilliard equations based on a microforce balance. Phys D Nonlinear Phenom 92:178–192

    Article  MathSciNet  MATH  Google Scholar 

  • Hakim V, Karma A (2009) Laws of crack motion and phase-field models of fracture. J Mech Phys Solids 57:342–368

    Article  MATH  Google Scholar 

  • Holzapfel G, Sommer G, Regitnig P (2004) Anisotropic mechanical properties of tissue components in human atherosclerotic plaques. J Biomech Eng 126:657–665

    Article  Google Scholar 

  • Holzapfel GA, Gasser TC, Ogden RW (2000) A new constitutive framework for arterial wall mechanics and a comparative study of material models. J Elast 61:1–48

    Article  MathSciNet  MATH  Google Scholar 

  • Humphrey JD (2002) Cardiovascular solid mechanics, cells, tissues, and organs. Springer, New York

    Book  Google Scholar 

  • James H, Guth E (1943) Theory of elastic properties of rubber. J Chem Phys 11:455–481

    Article  Google Scholar 

  • Kachanov LM (1986) Introduction to continuum damage mechanics, 1st edn. Springer, Netherlands

  • Kuhl E, Garikipati K, Arruda EM, Grosh K (2005) Remodeling of biological tissue: mechanically induced reorientation of a transversely isotropic chain network. J Mech Phys Solids 53:1552–1573

    Article  MathSciNet  MATH  Google Scholar 

  • Lanir Y (1979) A structural theory for the homogeneous biaxial stress–strain relationships in flat collagenous tissues. J Biomech 12:423–436

    Article  Google Scholar 

  • Lendon CL, Davies MJ, Born GVR, Richardson PD (1991) Atherosclerotic plaque caps are locally weakened when macrophages density is increased. Atherosclerosis 87:87–90

    Article  Google Scholar 

  • Linder C, Armero F (2007) Finite elements with embedded strong discontinuities for the modeling of failure in solids. Int J Numer Methods Eng 72:1391–1433

    Article  MathSciNet  MATH  Google Scholar 

  • Linder C, Raina A (2013) A strong discontinuity approach on multiple levels to model solids at failure. Comput Methods Appl Mech Eng 253:558–583

    Article  MATH  Google Scholar 

  • Loree HM, Kamm RD, Atkinson CM, Lee RT (1991) Turbulent pressure fluctuations on surface of model vascular stenoses. Am J Physiol 261:H644–H650

    Google Scholar 

  • Loree HM, Kamm RD, Stringfellow RG, Lee RT (1992) Effects of fibrous cap thickness on peak circumferencial stress in model atherosclerotic vessels. Circ Res 71:850–858

    Article  Google Scholar 

  • Mariano PM (2001) Multifield theories in mechanics of solids. Arch Appl Mech 38:1–93

    MathSciNet  Google Scholar 

  • Max N (1999) Weights for computing vertex normals from facet normals. J Gr Tools 4(2):1–6

    Article  Google Scholar 

  • Menzel A, Steinmann P (2001) A theoretical and computational framework for anisotropic continuum damage mechanics at large strains. Int J Solids Struct 38:9505–9523

    Article  MATH  Google Scholar 

  • Miehe C (2011) A multi-field incremental variational framework for gradient-extended standard dissipative solids. J Mech Phys Solids 59:898–923

    Article  MathSciNet  MATH  Google Scholar 

  • Miehe C, Schänzel L (2014) Phase field modeling of fracture in rubbery polymers. Part I: finite elasticity coupled with brittle failure. J Mech Phys Solids 65:93–113

    Article  MathSciNet  MATH  Google Scholar 

  • Miehe C, Göktepe S, Lulei F (2004) A micro–macro approach to rubber-like materials. Part I: the non-affine micro-sphere model of rubber elasticity. J Mech Phys Solids 52:2617–2660

    Article  MathSciNet  MATH  Google Scholar 

  • Miehe C, Hofacker M, Welschinger F (2010a) A phase field model for rate-independent crack propagation: Robust algorithmic implementation based on operator splits. Comput Methods Appl Mech Eng 199:2765–2778

    Article  MathSciNet  MATH  Google Scholar 

  • Miehe C, Welschinger F, Hofacker M (2010b) Thermodynamically consistent phase-field models of fracture: variational principles and multi-field fe implementations. Int J Numer Methods Eng 83:1273–1311

  • Miehe C, Schänzel L, Ulmer H (2014) Phase field modeling of fracture in multi-physics problems. Part I. Balance of crack surface and failure criteria for brittle crack propagation in thermo-elastic solids. Comput Methods Appl Mech Eng. doi:10.1016/j.cma.2014.11.016

  • Moës N, Dolbow J, Belytschko T (1999) A finite element method for crack growth without remeshing. Int J Numer Methods Eng 46:131–150

  • Mumford D, Shah J (1989) Optimal approximations by piecewise smooth functions and associated variational problems. Commun Pure Appl Math 42:577–685

    Article  MathSciNet  MATH  Google Scholar 

  • Oliver J (1996) Modelling strong discontinuities in solid mechanics via strain softening constitutive equations. Part1: fundamentals. Int J Numer Methods Eng 39:3575–3600

    Article  MATH  Google Scholar 

  • Ortiz M, Pandolfi A (1999) Finite-deformation irreversible cohesive elements for three-dimensional crack-propagation analysis. Int J Numer Methods Eng 44:1267–1282

    Article  MATH  Google Scholar 

  • Ortiz M, Quigley JJ (1991) Adaptive mesh refinement in strain localization problems. Comput Methods Appl Mech Eng 90:781–804

    Article  Google Scholar 

  • Raina A, Linder C (2014) A homogenization approach for nonwoven materials based on fiber undulations and reorientation. J Mech Phys Solids 65:12–34

    Article  MathSciNet  Google Scholar 

  • Rhodin JAG (1980) Architecture of the vessel wall. Compr Physiol Handb Physiol Cardiovasc Syst Vasc Smooth Muscle 2:1–31

  • Schulze-bauer CAJ, Regitnig P, Holzapfel GA (2002) Mechanics of the human femoral adventitia including the high-pressure response. Am J Physiol Heart Circ Physiol 282:2427–2440

    Article  Google Scholar 

  • Silver FH, Christiansen DL, Buntin CM (1989) Mechanical properties of the aorta: a review. Crit Rev Biomed Eng 17:323–358

    Google Scholar 

  • Simo JC, Oliver J, Armero F (1993) An analysis of strong discontinuities induced by strain-softening in rate-independent inelastic solids. Comput Mech 12:277–296

    Article  MathSciNet  MATH  Google Scholar 

  • Sommer G, Gasser TC, Regitnig P, Auer M, Holzapfel G (2008) Dissection properties of the human aortic media: an experimental study. J Biomech Eng 130:021007

  • Steinmann P, Miehe C, Stein E (1994) On the localization analysis of orthotropic Hill type elastoplastic solids. J Mech Phys Solids 42:1969–1994

    Article  MathSciNet  MATH  Google Scholar 

  • Vaishnav RN, Vossoughi J (1987) Residual stress and strain in aortic segments. J Biomech 20(3):235–239

    Article  Google Scholar 

  • Vaishnav RN, Young JT, Patel DJ (1973) Distribution of stresses and of strain-energy density through the wall thickness in a canine aortic segment. Circ Res 32:577–583

    Article  Google Scholar 

  • Wells GN, Sluys LJ (2001) A new method for modelling cohesive cracks using finite elements. Int J Numer Methods Eng 50:2667–2682

    Article  MATH  Google Scholar 

  • Xu XP, Needleman A (1994) Numerical simulations of fast crack growth in brittle solids. J Mech Phys Solids 42:1397–1434

    Article  MATH  Google Scholar 

Download references

Acknowledgments

Support for this research was provided by the German Research Foundation (DFG) for the Cluster of Excellence Exc 310 Simulation Technology at the University of Stuttgart.

Author information

Authors and Affiliations

  1. Institute of Applied Mechanics (CE), Chair I, University of Stuttgart, Pfaffenwaldring 7, 70569, Stuttgart, Germany

    Arun Raina & Christian Miehe

Authors
  1. Arun Raina
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Christian Miehe
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Christian Miehe.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Raina, A., Miehe, C. A phase-field model for fracture in biological tissues. Biomech Model Mechanobiol 15, 479–496 (2016). https://doi.org/10.1007/s10237-015-0702-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10237-015-0702-0

Keywords

Search

Navigation