Analysis and measurements of mixing in pressure-driven microchannel flow

Abstract

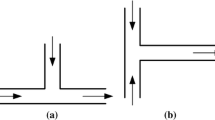
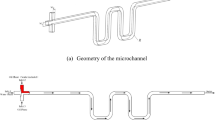
The mixing phenomena for two fluid streams in pressure-driven rectangular microchannels are analyzed and directly compared with the measurements of mixing intensity for a wide range of aspect ratio (width/depth = 1–20). In the analysis, the three-dimensional transport equation for species mixing was solved using the spectral method in a dimensionless fashion covering a large regime of the normalized downstream distance. The analysis reveals the details of non-uniform mixing process, which originates from the top and bottom walls of the channel and stretches out toward the center of the channel, and its transition to uniformity. Employing different length scales for the non-uniform and uniform mixing regimes, the growth of mixing intensity can be expressed in a simple relationship for various aspect ratios in the large range. The mixing experiments were carried out on silicon- and poly(methyl methacrylate) (PMMA)-based T-type micromixers utilizing fluids of pH indicator (in silicon channel) and fluorescent dye (in PMMA channel) to evaluate the mixing intensity based on flow visualization images. Using conventional microscopes, the experiments demonstrate the mixing intensity as a power law of the stream velocity for all the microfluidic channels tested. The variations of measured mixing intensity with the normalized downstream distance are found in favorable agreement with the numerical simulations. The comparison between the experiments and simulations tells the capabilities and limitations on the use of conventional microscopes to measure the mixing performance.

Appendices

Appendix 1: Discretization with the Chebyshev polynomials

The concentration C(ξ, η, Z*) is approximated by a truncated double series of Chebyshev polynomials as

$$C(\xi, \eta, Z^{ * })\,=\,{\sum\limits_{m\,=\,0}^M {{\sum\limits_{n\,=\,0}^N {C_{{mn}} (Z^{ * })T_{m} } }} }(\xi)T_{n} (\eta),$$

(19)

where T _m (ξ) and T _n (η) denote the Chebyshev polynomials and C _mn the expansion coefficient. By choosing the Chebyshev Gauss-Lobato collocation points

$$\xi _{i}\,=\,\cos \frac{{i\pi }}{M},\quad \eta _{j}\,=\,\cos \frac{{j\pi }}{N},\qquad i\,=\,0,1, \ldots, M,{\enspace}j\,=\,0,1, \ldots, N,$$

(20)

we can form the following discrete transform/inverse transform

$$C_{{mn}} (Z^{ * })\,=\,\frac{4}{{d_{i} d_{m} e_{j} e_{n} MN}}{\sum\limits_{i\,=\,0}^M {{\sum\limits_{j\,=\,0}^N {C(\xi _{i}, \eta _{j}, Z^{ * })T_{m}} }} } (\xi _{i})T_{n} (\eta _{j}),$$

(21a)

$$C(\xi _{i}, \eta _{j}, Z^{ * })\,=\,{\sum\limits_{m\,=\,0}^M {{\sum\limits_{n\,=\,0}^N {C_{{mn}} (Z^{ * })T_{m}} }} } (\xi _{i})T_{n} (\eta _{j}),$$

(21b)

where $d_{l}\,=\,\left\{ {\begin{array}{*{20}c} {{2},} & {{l\,=\,0,M},} \\ {{1},} & {{l\,=\,{\hbox{otherwise},}}} \\ \end{array} } \right.$ and $e_{l}\,=\,\left\{ {\begin{array}{*{20}c} {{2},} & {{l\,=\,0,N},} \\ {{1},} & {{l\,=\,{\hbox{otherwise}}.}} \\ \end{array} } \right.$ This means that the truncated double series in Eqs.  21a, 21b will interpolate C(ξ, η, Z*) at the collocation points ${\left({\xi _{i}, \eta _{j} } \right)},\;i\,=\,0,1, \ldots, M,j\,=\,0,1, \ldots, N.$ Substituting Eqs.  21a, 21b into Eq. 19, C(ξ, η, Z*) can be further expressed as

$$C(\xi, \eta, Z^{ * })\,=\,{\sum\limits_{i\,=\,0}^M {{\sum\limits_{j\,=\,0}^N {C(\xi _{i}, \eta _{j}, Z^{ * })\phi _{i} (\xi)\varphi _{j} (\eta)} }}, }$$

(22a)

where $\phi _{i} (\xi){\text{ and }}\varphi _{j} (\eta)$ are the Lagrange interpolating polynomials defined as

$$\phi _{i} (\xi)\,=\,{\mathop \Pi \limits_{{\begin{subarray}{ll} l\,=\,0,\\ l\,\ne\,i\end{subarray}}}^M }\frac{{\xi - \xi _{l} }}{{\xi _{i} - \xi _{l} }},$$

(22b)

$$\varphi _{j} (\eta)\,=\,{\mathop \Pi \limits_{\begin{subarray}{ll} l = 0, \\ l \,\ne\, j \end{subarray}} ^N }\frac{{\eta - \eta _{l} }}{{\eta _{j} - \eta _{l} }}.$$

(22c)

The derivatives

$$\frac{{\partial C}}{{\partial \xi }}{\left({\xi _{i}, \eta _{j}, Z^{ * } } \right)},\,\frac{{\partial ^{2} C}}{{\partial \xi ^{2} }}{\left( {\xi _{i}, \eta _{j}, Z^{ * } }\right)},\,\frac{{\partial C}}{{\partial \eta }}{\left({\xi _{i}, \eta _{j}, Z^{ * } } \right)},$$

and

$$\frac{{\partial ^{2} C}}{{\partial \eta ^{2} }}{\left({\xi _{i}, \eta_{j}, Z^{ * } } \right)}$$

can be obtained as

$$\frac{{\partial C}}{{\partial \xi }}{\left({\xi _{i}, \eta _{j}, Z^{ * } } \right)}\,=\,{\sum\limits_{m\,=\,0}^M {{\sum\limits_{n\,=\,0}^N {C(\xi _{m}, \eta _{n}, Z^{ * })\frac{{{\hbox{d}}\phi _{m} }}{{\hbox {d}}\xi }}(\xi _{i})\varphi _{n} (\eta _{j})} }}, $$

(23a)

$$\frac{{\partial ^{2} C}}{{\partial \xi ^{2} }}{\left({\xi _{i}, \eta _{j}, Z^{ * } } \right)}\,=\,{\sum\limits_{m\,=\,0}^M {{\sum\limits_{n = 0}^N {C(\xi _{m}, \eta _{n}, Z^{ * })\frac{{\hbox{d}^{2} \phi _{m} }}{{\hbox{d}\xi ^{2} }}(\xi _{i})\varphi _{n} (\eta _{j})} }}, }$$

(23b)

$$\frac{{\partial C}}{{\partial \eta }}{\left({\xi _{i}, \eta _{j}, Z^{ * } } \right)}\,=\,{\sum\limits_{m\,=\,0}^M {{\sum\limits_{n\,=\,0}^N {C(\xi _{m}, \eta _{n}, Z^{ * })\phi _{m} (\xi _{i})\frac{{\hbox{d}\varphi _{n} }}{{\hbox{d}\eta }}(\eta _{j})} }}, }$$

(23c)

$$\frac{{\partial ^{2} C}}{{\partial \eta ^{2} }}{\left({\xi _{i}, \eta _{j}, Z^{ * } } \right)}\,=\,{\sum\limits_{m\,=\,0}^M {{\sum\limits_{n = 0}^N {C(\xi _{m}, \eta _{n}, Z^{ * })\phi _{m} (\xi _{i})\frac{{\hbox{d}^{2} \varphi _{n} }}{{\hbox{d}\eta ^{2} }}(\eta _{j})} }}, }$$

(23d)

where $\left[{{d\phi _{m} }}{\left({\xi _{i} } \right)}/{{d\xi }}\right]\,{\hbox{ and }}\,\left[{{d\varphi _{n} }}{\left({\eta _{j} } \right)}/{{d\eta }}\right]$ are usually denoted as the Chebyshev collocation derivative matrices formulated as

$${\left({{\mathbf{D}}_{\xi } } \right)}_{{im}}\,=\,\frac{{\hbox{d}\phi _{m} }}{{\hbox{d}\xi }}{\left({\xi _{i} } \right)}\,=\,\left\{ {\begin{array}{*{20}l} {{\frac{{{\hbox {d}_{i}} (- 1)^{{i + m}} }}{{{\hbox {d}_{m}} (\xi _{i} - \xi _{m})}},} } & {{i \ne m},} \\ {{\frac{{ - \xi _{m} }}{{2(1 - \xi ^{2}_{m})}},}} & {{1 \leq i\,=\,m \leq M - 1},} \\ {{\frac{{2M^{2} + 1}}{6},}} & {{i\,=\,m\,=\,0},} \\ {{ - \frac{{2M^{2} + 1}}{6},}} & {{i\,=\,m\,=\,M},} \\ \end{array} } \right.$$

(24a)

and likewise

$${\left({{\mathbf{D}}_{{_{\eta } }} } \right)}_{{jn}} = \frac{{\hbox{d}\varphi _{n} }}{{\hbox{d}\eta }}{\left({\eta _{j} } \right)}\,=\,\left\{ {\begin{array}{*{20}l} {{\frac{{e_{j} (- 1)^{{j + n}} }}{{e_{n} (\eta _{j} - \eta _{n})}},}} & {{j \ne n}, } \\ {{\frac{{ - \eta _{n} }}{{2(1 - \eta ^{2}_{n})}},}} & {{1 \leq j\,=\,n \leq N - 1}, } \\ {{\frac{{2N^{2} + 1}}{6},} } & {{j\,=\,n\,=\,0},} \\ {{ - \frac{{2N^{2} + 1}}{6},}} & {{j\,=\,n\,=\,N}.} \\ \end{array} } \right.$$

(24b)

The second derivative collocation matrices can then be obtained as

$$\frac{{\hbox{d}^{2} \phi _{m} }}{{\hbox{d}\xi ^{2} }}{\left({\xi _{i} } \right)} = {\left({{\mathbf{D}}^{2}_{\xi } } \right)}_{{im}}, $$

(25a)

$$\frac{{\hbox{d}^{2} \varphi _{n} }}{{\hbox{d}\eta ^{2} }}{\left({\eta _{j} } \right)}\,=\,{\left({{\mathbf{D}}^{2}_{\eta } } \right)}_{{jn}}.$$

(25b)

The boundary and initial conditions associated with the discretized equation are written as

$$C(- 1,\eta _{j}, Z^{ * })\,=\,1,\quad \frac{{\partial C}}{{\partial \eta }}(\xi _{i}, \pm 1,Z^{ * })\,=\,\frac{{\partial C}}{{\partial \xi }}(1,\eta _{j}, Z^{ * })\,=\,0,\quad i\,=\,0,1, \ldots, M,{\enspace}j\,=\,0,1, \ldots, N,$$

(26a)

$$C(\xi _{i}, \eta _{j}, 0)\,=\,0,\quad i\,=\,0,1, \ldots, M,{\enspace}j\,=\,0,1, \ldots, N,$$

(26b)

The actual computations of these derivatives can be done alternatively by the transform and inverse transform in Eqs.  21a, 21b, which can speed up via FFT. The details are referred to Canuto et al. (1988) and Trefethen (2000).

Appendix 2: Rescaling the coordinates for parabolic velocity profile

For the channel with large γ, where the velocity is portrayed as a parabolic profile, Eq. 7a is rewritten as

$$\frac{3}{2}(1 - 4Y^{2})\frac{{\partial C}}{{\partial Z^{ + } }} \,=\,\frac{{\partial ^{2} C}}{{\partial \chi ^{2} }} + \frac{{\partial ^{2} C}}{{\partial Y^{2} }},$$

(27)

where the downstream distance is rescaled as Z ⁺ = γ² Z*=Dz/(h ² u ₀), in reference to the channel depth instead of the channel width, and the dimensionless width is in the small scale χ = γ X. Notably, Eq. 27 now does not contain γ, i.e. independent of aspect ratio. Equation 27 is then solved with the same boundary conditions of Eq. 7b for the concentration C(χ, Y, Z ⁺) and the mixing intensity can be obtained by integrating C(χ, Y, Z ⁺) over χ from the midline (χ = 0) to the outer limit (χ = ±δ/2h) of the concentration boundary layer,

$$I_{{{\text{mix}}}} (Y,Z^{ + })\,=\,\frac{1}{\gamma }{\left[ {{\int_{\frac{{ - \delta }}{{2h}}}^0 {C^{\prime} (\chi, Y,Z^{ + })\hbox{d}\chi } } + {\int_0^{\frac{\delta }{{2h}}} {C(\chi, Y,Z^{ + })\hbox{d}\chi } }} \right]},$$

(28a)

or

$$\gamma I_{{{\text{mix}}}} (Y,Z^{ + })\,=\,{\int_{\frac{{ - \delta }}{{2h}}}^0 {C^{\prime} (\chi, Y,Z^{ + })d\chi } } + {\int_0^{\frac{\delta }{{2h}}} {C(\chi, Y,Z^{ + })\hbox{d}\chi } },$$

(28b)

where δ is taken as the dimensional width of interdiffusion region with a very small cutoff concentration (e.g. 1% or less). Then the product of I _mix and γ given by Eq. 28b is independent of aspect ratio until δ becomes large enough to touch the sidewalls.