Skip to main content
SpringerLink
Log in

Using Pseudo-Parabolic and Fractional Equations for Option Pricing in Jump Diffusion Models

  • Published:
Computational Economics Aims and scope Submit manuscript

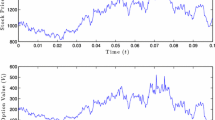
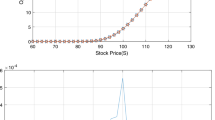
Abstract

In mathematical finance a popular approach for pricing options under some Lévy model would be to consider underlying that follows a Poisson jump diffusion process. As it is well known this results in a partial integro-differential equation (PIDE) that usually does not allow an analytical solution, while a numerical solution also faces some problems. In this paper we develop a new approach on how to transform the PIDE into a class of so-called pseudo-parabolic equations which are well known in mathematical physics but are relatively new for mathematical finance. As an example we will discuss several jump-diffusion models which Lévy measure allows such a transformation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  • Abu-Saman A. M., Assaf A. M. (2007) Stability and convergence of Crank-Nicholson method for fractional advection dispersion equation. Advances in Applied Mathematical Analysis 2: 117–125

    Google Scholar 

  • Amin K. (1993) Jump diffusion option valuation in discrete time. Journal of Finance 48: 1833–1863

    Google Scholar 

  • Andersen L., Andreasen J. (2000) Jump diffusion processes: Volatility smile fitting and numerical methods for option pricing. Review of Derivatives Research 4: 231–262

    Article  Google Scholar 

  • Bakas I., Khesin B., Kiritsis E. (1993) The logarithm of the derivative operator and higher spin algebras of w ∞ type. Communications in Mathematical Physics 151(2): 233–243

    Article  Google Scholar 

  • Boyarchenko S., Levendorskii S. (2002) Non-Gaussian Merton–Black–Scholes theory. World Scientific, Singapore

    Book  Google Scholar 

  • Bu, Y. (2007). Option pricing using Levy processes. PhD thesis, Department of Mathematical Statistics, Chalmers University of Technology and Goteborg University, GA.

  • Cannon J., Lin Y. (1988) Classical and weak solutions for one-dimensional pseudo-parabolic equations with typical boundary data. Annali di Matematica Pura ed Applicata 152: 375–385

    Article  Google Scholar 

  • Carr P., Geman H., Madan D., Yor M. (2002) The fine structure of asset returns: An empirical investigation. Journal of Business 75: 305–332

    Article  Google Scholar 

  • Carr P., Geman G., Madan D., Yor M. (2005) Pricing options on realized variance. Finance and Stochastics 4: 453–475

    Article  Google Scholar 

  • Carr P., Mayo A. (2007) On the numerical evaluation of option prices in jump diffusion processes. The European Journal of Finance 13: 353–372

    Article  Google Scholar 

  • Carr P., Wux L. (2004) Time-changed Lévy processes and option pricing. Journal of Financial Economics 71: 113–141

    Article  Google Scholar 

  • Cartea A., del Castillo-Negrete D. (2007) Fractional diffusion models of option prices in markets with jumps. Physica A 374: 749–763

    Article  Google Scholar 

  • Cont, R. (eds) (2009) Frontiers in quantitative finance: Volatility and credit risk modeling. Wiley Finance Press, New York

    Google Scholar 

  • Cont R., Tankov P. (2004) Financial modelling with jump processes. Financial matematics series. Chapman & Hall/CRC, Boca Raton

    Google Scholar 

  • Cont, R., & Voltchkova, E. (2003). A finite difference scheme for option pricing in jump diffusion and exponential Lévy models. Technical Report 513, Rapport Interne CMAP.

  • d’Halluin Y., Forsyth P. A., Labahn G. (2005) A semi-lagrangian approach for American Asian options under jump diffusion. SIAM Journal on Scientific Computing 27: 315–345

    Article  Google Scholar 

  • d’Halluin Y., Forsyth P. A., Vetzal K. R. (2004) A penalty method for American options with jump diffusion processes. Numerische Mathematik 97: 321–352

    Article  Google Scholar 

  • d’Halluin Y., Forsyth P. A., Vetzal K. R. (2005) Robust numerical methods for contingent claims under jump diffusion processes. IMA Journal of Numerical Analysis 25: 87–112

    Article  Google Scholar 

  • Eberly, D. (2008). Derivative approximation by finite differences. http://www.geometrictools.com/Documentation/FiniteDifferences.pdf. Accessed 2 March 2008.

  • Evans, M., Hastings, N., & Peacock, B. (2000). Erlang distribution. In Statistical distributions (3rd ed., Chap. 12, pp. 71–73). New York: Wiley.

  • Hilber N., Reich N., Schwab C., Winter C. (2009) Numerical methods for Lévy processes. Finance and Stochastics 13: 471–500

    Article  Google Scholar 

  • In’t Hout K. J., Welfert B. D. (2009) Unconditional stability of second-order ADI schemes applied to multi-dimensional diffusion equations with mixed derivative terms. Applied Numerical Mathematics 59: 677–692

    Article  Google Scholar 

  • Itkin, A., & Carr, P. (2006). Finite-difference approach to pricing barrier options under stochastic skew model. In Global derivatives & risk conference, May 8–12, Paris, France. http://www.chem.ucla.edu/~itkin/publications/Paris2006.pdf.

  • Itkin, A., Carr, P. (2011). Jumps without tears: A new splitting technology for barrier options. IJNAM, 8(4),667–704.

    Google Scholar 

  • Koponen I. (1995) Analytic approach to the problem of convergence of truncated Levy flights towards the gaussian stochastic process. Physical Review E 52: 1197–1199

    Article  Google Scholar 

  • Lanser D., Verwer J. (1999) Analysis of operator splitting for advection–discussion–reaction problems from air pollution modelling. Journal of Computational and Applied Mathematics 111: 201–216

    Article  Google Scholar 

  • Lipton A., Sepp A. (2009) Credit value adjustment for credit default swaps via the structural default model. The Journal of Credit Risk 5: 123–146

    Google Scholar 

  • Madan D., Seneta E. (1990) The variance gamma (V.G.) model for share market returns. Journal of Business 63: 511–524

    Article  Google Scholar 

  • Marom O., Momoniat E. (2009) A comparison of numerical solutions of fractional diffusion models in finance. Nonlinear Analysis: Real World Applications 10: 3435–3442

    Article  Google Scholar 

  • Matache, A., von Petersdorff, T., & Schwabx, C. (2002). Fast deterministic pricing of options on levy driven assets. Technical report, Risk Lab, ETH, Zurich.

  • Meerschaert M. M., Tadjeran C. (2004) Finite difference approximations for fractional advection–dispersion flow equations. Journal of Computational and Applied Mathematics 172: 65–77

    Article  Google Scholar 

  • Meerschaert M. M., Tadjeran C. (2006) Finite difference approximations for two-sided space-fractional partial differential equations. Applied Numerical Mathematics 56: 80–90

    Article  Google Scholar 

  • Oldham, K. B., & Spanier, J. (1974). The fractional calculus: Theory and applications of differentiation and integration to arbitrary order. Mathematics in science and engineering, V. San Diego, CA: Academic Press.

  • Sousa, E. (2008). Finite difference approximations for a fractional advection diffusion problem. Technical Report 08-26, Departamento de Matematica, Universidade de Coimbra.

  • Strauss, A. K. (2006). Numerical analysis of jump-diffusion models for option pricing. PhD thesis, Virginia Polytechnic Institute and State University.

  • Tadjeran C., Meerschaert M., Scheffler H.-P. (2006) A second-order accurate numerical approximation for the fractional diffusion equation. Journal of Computational Physics 213: 205–213

    Article  Google Scholar 

  • Tavella D., Randall C. (2000) Pricing financial instruments. The finite-difference method. Wiley series in financial engineering. Wiley, New York

    Google Scholar 

  • Wilmott P. (1998) Derivatives. Wiley, New York

    Google Scholar 

  • Yoshida H. (1990) Construction of higher order symplectic integrators. Physics Letters 150: 262–268

    Article  Google Scholar 

  • Zhang X. (1993) Numerical-analysis of American option pricing in a jump-diffusion model. Mathematics of Operations Research 22: 668–690

    Article  Google Scholar 

  • Zhang K., Wang S. (2009) A computational scheme for options under jump diffusion processes. International Journal of Numerical Analysis and Modeling 6: 110–123

    Google Scholar 

  • Zhou, J. (2006). Option pricing under generalized tempered stable processes. PhD thesis, University of Delware.

Download references

Author information

Authors and Affiliations

  1. New York University Polytechnic Institute, 6 Metro Tech Center, RH 517E, Brooklyn, NY, 11201, USA

    Andrey Itkin

  2. Morgan Stanley, 585 Broadway, New York, NY, 10036, USA

    Peter Carr

  3. New York University, New York, NY, USA

    Peter Carr

Authors
  1. Andrey Itkin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Peter Carr
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Andrey Itkin.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Itkin, A., Carr, P. Using Pseudo-Parabolic and Fractional Equations for Option Pricing in Jump Diffusion Models. Comput Econ 40, 63–104 (2012). https://doi.org/10.1007/s10614-011-9269-8

Download citation

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10614-011-9269-8

Keywords

Mathematics Subject Classification (2000)

Search

Navigation