Skip to main content

Advertisement

SpringerLink
Log in

An improved neuronal energy model that better captures of dynamic property of neuronal activity

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

Among the theories of neural information coding, the neural energy coding is more accessible to global coding features than traditional neural encoding. According to the shortcomings existing in the neuronal energy model, that is, the non-smooth nature of the energy curve, we proposed an improved neuronal energy model in this paper. The modified energy model is a good choice for establishment of the global model of brain function. And it is also the basis of energy calculation for functional cognitive neural networks in the future.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

References

  1. McLaughlin, D.W.: Ruling out and ruling in neural codes. Proc. Natl. Acad. Sci. (PNAS) 106(14), 5936–5941 (2009)

    Article  Google Scholar 

  2. Velde, F.V.D., Kamps, M.D.: The necessity of connection structures in neural models of variable binding. Cogn. Neurodyn. 9(4), 1–12 (2015)

  3. Kozma, R.: Reflections on a giant of brain science. Cogn. Neurodyn. 10(6), 457–469 (2016)

    Article  Google Scholar 

  4. Tozzi, A., Peters, J.F.: Towards a fourth spatial dimension of brain activity. Cogn. Neurodyn. 10(3), 189–199 (2016)

    Article  Google Scholar 

  5. Wang, R., Zhu, Y.: Can the activities of the large scale cortical network be expressed by neural energy–a brief review. Cogn. Neurodyn. 10(1), 1–5 (2016)

    Article  MathSciNet  Google Scholar 

  6. Laughlinl, S.B., Sejnowski, T.J.: Communication in neural networks. Science 301(5641), 1870–1873 (2003)

    Article  Google Scholar 

  7. Abbott, L.F.: Theoretical neuroscience rising. Neuron 60(3), 489–495 (2008)

    Article  Google Scholar 

  8. Wang, R., Tsuda, I., Zhang, Z.: A new work mechanism on neuronal activity. Int. J. Neural Syst. 25(03), 1450037 (2015)

    Article  Google Scholar 

  9. Hyder, F., Rothman, D.L., Shulman, R.G.: Total neuroenergetics support localized brain activity: implications for the interpretation of fMRI. Proc. Natl. Acad. Sci. (PNAS) 99(16), 10771–10776 (2002)

    Article  Google Scholar 

  10. Smith, A.J., Blumenfeld, H., Behar, K.L., Rothman, D.L., Shulman, R.G.: Cerebral energetics and spiking frequency: the neurophysiological basis of fMRI. Proc. Natl. Acad. Sci. (PNAS) 99(16), 10765–10770 (2002)

    Article  Google Scholar 

  11. Raichle, M.E., Gusnard, D.A.: Appraising the brain’s energy budget. Proc. Natl. Acad. Sci. (PNAS) 99(16), 10237–10239 (2002)

    Article  Google Scholar 

  12. Laughlin, S.B.: Energy as a constraint on the coding and processing of sensory information. Curr. Opin. Neurobiol. 11, 475–480 (2001)

    Article  Google Scholar 

  13. Levy, W.B., Baxter, R.A.: Energy efficient neural codes. Neural Comp. 8(3), 531–543 (2003)

    Article  Google Scholar 

  14. Fox, M.D., Raichle, M.E.: Spontaneous fluctuations in brain activity observed with functional magnetic resonance imaging. Nature 8, 700–711 (2007)

    Google Scholar 

  15. Bleton, H., Sejdić, E.: A cerebral blood flow evaluation during cognitive tasks following a cervical spinal cord injury: a case study using transcranial Doppler recordings. Cogn. Neurodyn. 9(6), 615–626 (2015)

  16. Wang, R., Zhang, Z.: Mechanism on brain information processing: energy coding. Appl. Phys. Lett. (APL) 89(12), 123903 (2006)

    Article  Google Scholar 

  17. Wang, R., Zhang, Z., Chen, G.: Energy coding and energy functions for local activities of brain. Neurocomputing 73(1–3), 139–150 (2009)

    Article  Google Scholar 

  18. Wang, R., Zhang, Z., Chen, G.: Energy function and energy evolution on neural population. IEEE Trans. Neural Netw. 19(3), 535–538 (2008)

    Article  Google Scholar 

  19. Wang, R., Wang, Z., Li, Y.: Neurodynamics analysis on neural energy encoding. Nonlinear Dyn. (Submitted) (2017)

  20. Wang, Z., Wang, R.: Energy distribution property and energy coding of a structural neural network. Front. Comput. Neurosci. 8, 14 (2014)

    Article  Google Scholar 

  21. Wang, R., Wang Z., Zhu, Z.: The dynamics property of neuronal activity from the consistency of two different neuron models. Nonlinear Dyn. (Submitted) (2017)

  22. Wang, Z., Wang, R., Fang, R.: Energy coding in neural network with inhibitory neurons. Cogn. Neurodyn. 9(2), 129–144 (2015)

    Article  MathSciNet  Google Scholar 

  23. Lin, A., Fox, P.T., Hardies, J., Duong, T.Q., Gao, J.-H.: Nonlinear coupling between cerebral blood flow, oxygen consumption, and ATP production in human visual cortex. Proc. Natl. Acad. Sci. PNAS 107(18), 8447 (2010)

    Google Scholar 

  24. Qin, S., Yin, H., Yang, C., Dou, Y., Liu, Z., Zhang, P., Yu, H., Huang, Y., Feng, J., Hao, J., Hao, J., Deng, L., Yan, X., Dong, X., Zhao, Z., Jiang, T., Wang, H.-W., Luo, S.-J., Xie, C.: A magnetic protein biocompass. Nature Mater. 15, 217–226 (2016)

    Article  Google Scholar 

  25. Zhang, Y., Pan, X., Wang, R.: Functional connectivity between prefrontal cortex and striatum estimated by phase locking value. Cogn. Neurodyn. 10, 245–254 (2016)

    Article  Google Scholar 

  26. Zheng, H., Wang, R., Qu, J.: Effect of different glucose supply conditions on neuronal energy metabolism. Cogn. Neurodyn. 10(6), 563–571 (2016)

    Article  Google Scholar 

  27. Wang, Y., Wang, R., Zhu, Y.: Optimal path-finding through mental exploration based on neural energy field gradients. Cogn. Neurodyn. 10(6), 1–13 (2016)

    Article  MathSciNet  Google Scholar 

  28. Zhang, H., Wang, Q., Perc, M., Chen, G.: Synaptic plasticity induced transition of spike propagation in neuronal networks. Commun. Nonlinear Sci. Numer. Simul. 18(3), 601–615 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  29. Guo, D., Chen, M., Perc, M., et al.: Firing regulation of fast-spiking interneurons by autaptic inhibition. EPL 114(3), 30001 (2016)

    Article  Google Scholar 

  30. Wang, Q., Chen, G., Perc, M.: Synchronous bursts on scale-free neuronal networks with attractive and repulsive coupling. PLoS One 6(1), e15851 (2010)

    Article  Google Scholar 

  31. Guo, D., Wang, Q., Perc, M.: Complex synchronous behavior in interneuronal networks with delayed inhibitory and fast electrical synapses. Phys. Rev. E Stat. Nonlinear Soft Matter Phys. 85(1), 878–896 (2012)

    Google Scholar 

Download references

Acknowledgements

This work is supported by the National Natural Science Foundation of China under (Grant Nos. 11232005, 11472104, 61633010 and 61473110).

Author information

Authors and Affiliations

  1. School of Computer Science, Hangzhou Dianzi University, Hangzhou, China

    Rubin Wang

  2. Institute for Cognitive Neurodynamics, East China University of Science and Technology, Shanghai, 200237, China

    Yanyan Wang & Rubin Wang

Authors
  1. Yanyan Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Rubin Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Rubin Wang.

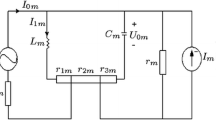
Appendix

Appendix

$$\begin{aligned} d_{1m}= & {} C_m ^{2}\left( {1+\frac{r_{3m} }{r_m }} \right) \\&\quad \times \left( {r_{0m} +r_{2m} +r_{3m} +\frac{r_{3m} \left( {r_{0m} +r_{2m} } \right) }{r_m }} \right) \\ d_{2m}= & {} C_m \left( {2r_{0m} +r_{2m} +r_{3m} +\frac{2r_{0m} r_{3m} +r_{2m} r_{3m} }{r_m }} \right) \\&\times \left( {Ke^{-at}-\frac{r_{2m} }{L_m }e^{-at}\int _{t_0 }^t {I_m e^{at}\mathrm{d}t} } \right) \\&{-\,2C_m \left( {1+\frac{r_{3m} }{r_m }} \right) \left( {r_{0m} +r_{2m} } \right) I_m } \\ d_{3m}= & {} C_m \left[ 1+2r_{0m} \left( {1+\frac{r_{3m} }{r_m }} \right) \frac{r_m +r_{1m}+r_{2m} }{r_m r_{1m} } \right. \\&\left. +\frac{r_m r_{2m} +r_m r_{3m} +r_{2m} r_{3m} }{r_m ^{2}}\left( {2+\frac{r_m +r_{2m} }{r_{1m} }} \right) \right] \\ d_{4m}= & {} \frac{r_m +r_{1m} +r_{2m} }{r_m ^{2}r_{1m} }\\&\quad \times \left( {\frac{r_{0m} }{r_{1m} }\left( {r_m +r_{1m} +r_{2m} } \right) +r_m +r_{2m} } \right) \\ d_{5m}= & {} \frac{2r_{0m} \left( {r_m +r_{1m} +r_{2m} } \right) +r_{1m} \left( {r_m +r_{2m} } \right) }{r_m r_{1m} }\\&\quad \times \left( {Ke^{-at}-\frac{r_{2m} }{L_m }e^{-at}\int _{t_0 }^t {I_m e^{at}\mathrm{d}t} } \right) \end{aligned}$$
$$\begin{aligned}&{-\frac{2r_{0m} \left( {r_m +r_{1m} +r_{2m} } \right) +r_{2m} \left( {r_m +2r_{1m} +r_{2m} } \right) }{r_m r_{1m} }\times I_m } \\ d_{6m}= & {} \left[ r_{0m} \left( {Ke^{-at}-\frac{r_{2m} }{L_m }e^{-at}\int _{t_0 }^t {I_m e^{a\tau }\mathrm{d}\tau } } \right) \right. \\&\left. -\,(r_{0m} +r_{2m} )I_m \right] \left( {Ke^{-at}-\frac{r_{2m} }{L_m }e^{-at}\int _{t_0 }^t {I_m e^{a\tau }\mathrm{d}\tau } -I_m } \right) \\&\quad \times \int _{t_0 }^t {I_m e^{a\tau }\mathrm{d}\tau } =\sum _{j=0}^n {\Gamma _j } +\sum _{j=0}^n {J_{mj} \frac{e^{at}-e^{at_j }}{a}} \\&-\,i_{0m\left( n\right) } \frac{e^{at}-e^{at_n }}{a}\sin \varphi _n \\&+\frac{i_{0m\left( n \right) } }{a^{2}+\omega _{m\left( n \right) } ^{2}}\left( ae^{at}\sin \left( {\omega _{m\left( n \right) } \left( {t-t_n } \right) +\varphi _n } \right) \right. \\&\left. -\,ae^{at_n }\sin \varphi _n \right) \\&+\frac{i_{0m\left( n \right) } }{a^{2}+\omega _{m\left( n \right) } ^{2}}\left( -\omega _{m\left( n \right) } e^{at}\cos \left( {\omega _{m\left( n \right) } \left( {t-t_n } \right) +\varphi _n } \right) \right. \\&\left. +\,\omega _{m\left( n \right) } e^{at_n }\cos \varphi _n \right) \end{aligned}$$

where

$$\begin{aligned} y_2= & {} \frac{i_{0m\left( n \right) } \omega _{m\left( n \right) } ^{2}r_{2m} \left( {al_1 +l_3 } \right) }{2L_m d_{1m} \left( {a^{2}+\omega _{m\left( n \right) } ^{2}} \right) \left( {\lambda _m ^{2}+\omega _{m\left( n \right) } ^{2}} \right) } \\&+\frac{i_{0m\left( n \right) } \lambda _m \left[ {ar_{2m} l_3 +a^{2}l_4 L_m +\omega _{m\left( n \right) } ^{2}\left( {l_4 L_m -l_1 r_{2m} } \right) -\left( {a^{2}+\omega _{m\left( n \right) } ^{2}} \right) \omega _{m\left( n \right) } l_2 L_m } \right] }{2L_m d_{1m} \left( {a^{2}+\omega _{m\left( n \right) } ^{2}} \right) \left( {\lambda _m ^{2}+\omega _{m\left( n \right) } ^{2}} \right) } \\ y_3= & {} \frac{-\lambda _m i_{0m\left( n \right) } \omega _{m\left( n \right) } r_{2m} \left( {al_1 +l_3 } \right) }{2L_m d_{1m} \left( {a^{2}+\omega _{m\left( n \right) } ^{2}} \right) \left( {\lambda _m ^{2}+\omega _{m\left( n \right) } ^{2}} \right) } \\&+\frac{i_{0m\left( n \right) } \omega _{m\left( n \right) } \left[ {ar_{2m} l_3 +a^{2}l_4 L_m +\omega _{m\left( n \right) } ^{2}\left( {l_4 L_m -l_1 r_{2m} } \right) -\left( {a^{2}+\omega _{m\left( n \right) } ^{2}} \right) \omega _{m\left( n \right) } l_2 L_m } \right] }{2L_m d_{1m} \left( {a^{2}+\omega _{m\left( n \right) } ^{2}} \right) \left( {\lambda _m ^{2}+\omega _{m\left( n \right) } ^{2}} \right) } \end{aligned}$$
$$\begin{aligned} C_n= & {} \frac{r_{2m} \left( {al_1 +l_3 } \right) \left( {\omega _{m\left( n \right) } \cos \varphi _n -a\sin \varphi _n } \right) }{\left( {a^{2}+\omega _{m\left( n \right) } ^{2}} \right) \left( {a+\lambda _m } \right) }\\&-\frac{l_3 r_{2m} -l_1 r_{2m} \lambda _m +l_4 L_m \left( {a+\lambda _m } \right) }{\lambda _m \left( {a+\lambda _m } \right) }\sin \varphi _n \\&+\frac{\omega _{m\left( n \right) } r_{2m} \left( {al_1 +l_3 } \right) \left( {\omega _{m\left( n \right) } \sin \varphi _n -\lambda _m \cos \varphi _n } \right) }{\left( {a^{2}+\omega _{m\left( n \right) } ^{2}} \right) \left( {\lambda _m ^{2}+\omega _{m\left( n \right) } ^{2}} \right) } \\&-\frac{\omega _{m\left( n \right) } ^{2}l_1 r_{2m} -ar_{2m} l_3 +L_m \left( {a^{2}+\omega _{m\left( n \right) } ^{2}} \right) \left( {\omega _{m\left( n \right) } l_2 -l_4 } \right) }{\left( {a^{2}+\omega _{m\left( n \right) } ^{2}} \right) \left( {\lambda _m ^{2}+\omega _{m\left( n \right) } ^{2}} \right) }\\&\quad \left( {\lambda _m\sin \varphi _n +\omega _{m\left( n \right) } \cos \varphi _n } \right) \\ D_n= & {} \frac{\left( {al_1 +l_3 } \right) KL_m }{\left( {a+\lambda _m } \right) }-\frac{r_{2m} \left( {al_1 +l_3 } \right) e^{-\lambda _m t_n }}{a\lambda _m }\sum _{k=0}^n {J_{mk} } \end{aligned}$$
$$\begin{aligned}&+\frac{r_{2m} \left( {al_1 +l_3 } \right) e^{-(a+\lambda _m )t_n }}{\left( {a+\lambda _m } \right) }\sum _{k=0}^n {\left( {J_{mk} \frac{e^{at_k }}{a}-\Gamma _k } \right) } \\&-\frac{l_1 r_{2m} -l_4 L_m }{\lambda _m }\sum _{j=0}^n {J_{mj} e^{-\lambda _m t_j }} \\&+\frac{r_{2m} \left( {al_1 +l_3 } \right) }{\left( {a+\lambda _m } \right) }\sum _{j=1}^n \sum _{k=0}^{j-1} {\left( {\Gamma _k -J_{mk} \frac{e^{at_k }}{a}} \right) } \\&\quad \times \left( {e^{-(a+\lambda _m )t_j }-e^{-(a+\lambda _m )t_{j-1} }} \right) \\&+\frac{r_{2m} \left( {al_1 +l_3 } \right) }{a\lambda _m }\sum _{j=1}^n {\sum _{k=0}^{j-1} {J_{mk} } \left( {e^{-\lambda _m t_j }-e^{-\lambda _m t_{j-1} }} \right) } \\&+\,r_{2m} \left( {al_1 +l_3 } \right) \sum _{j=1}^n \frac{i_{0m\left( {j-1} \right) } }{\left( {a^{2}+\omega _{m\left( {j-1} \right) } ^{2}} \right) \left( {\lambda _m ^{2}+\omega _{m\left( {j-1} \right) } ^{2}} \right) }\\&\times \left( {a\mathrm{A}_j +\omega _{m\left( {j-1} \right) } \mathrm{B}_j } \right) \\&+\left( {l_4 L_m -l_1 r_{2m} } \right) \sum _{j=1}^n {\frac{i_{0m\left( {j-1} \right) } }{\lambda _m ^{2}+\omega _{m\left( {j-1} \right) } ^{2}}A_j } -l_2 L_m \end{aligned}$$
$$\begin{aligned}&\quad \sum _{j=1}^n {\frac{i_{0m\left( {j-1} \right) } \omega _{m\left( {j-1} \right) } }{\lambda _m ^{2}+\omega _{m\left( {j-1} \right) } ^{2}}} A_j \\&+\frac{r_{2m} \left( {al_1 +l_3 } \right) }{\left( {a+\lambda _m } \right) }\sum _{j=1}^n \frac{i_{0m\left( {j-1} \right) } }{a^{2}+\omega _{m\left( {j-1} \right) } ^{2}}\\&\quad \times \left( {e^{-(a+\lambda _m )t_j +at_{j-1} }-e^{-\lambda _m t_{j-1} }} \right) \\&\quad \times \left( {\omega _{m\left( {j-1} \right) } \cos \varphi _{j-1} -a\sin \varphi _{j-1} } \right) \\&+\frac{l_1 r_{2m} -l_4 L_m }{\lambda _m }\sum _{j=1}^n i_{0m\left( {j-1} \right) } \\&\quad \times \left( {e^{-\lambda _m t_j }-e^{-\lambda _m t_{j-1} }} \right) \sin \varphi _{j-1} \\&-\frac{r_{2m} \left( {al_1 +l_3 } \right) }{a\lambda _m }\sum _{j=1}^n i_{0m\left( {j-1} \right) } \sin \varphi _{j-1} \\&\quad \times \left( {e^{-\lambda _m t_j }-e^{-\lambda _m t_{j-1} }} \right) \\&+\frac{r_{2m} \left( {al_1 +l_3 } \right) }{a\left( {a+\lambda _m } \right) }\sum _{j=1}^n i_{0m\left( {j-1} \right) } \sin \varphi _{j-1} \end{aligned}$$
$$\begin{aligned}&\quad \times \left( {e^{-(a+\lambda _m )t_j +at_{j-1} }-e^{-\lambda _m t_{j-1} }} \right) \\ \mathrm{A}_j= & {} \lambda _m e^{-\lambda _m t_j }\sin \left( {\omega _{m\left( {j-1} \right) } \left( {t_j -t_{j-1} } \right) +\varphi _{j-1} } \right) +\omega _{m\left( {j-1} \right) }\\&\quad \times e^{-\lambda _m t_j }\cos \left( {\omega _{m\left( {j-1} \right) } \left( {t_j -t_{j-1} } \right) +\varphi _{j-1} } \right) \\&-\,\lambda _m e^{-\lambda _m t_{j-1} }\sin \varphi _{j-1} -\omega _{m\left( {j-1} \right) } e^{-\lambda _m t_{j-1} }\cos \varphi _{j-1}\\ \mathrm{B}_j= & {} \omega _{m\left( {j-1} \right) } e^{-\lambda _m t_j }\sin \left( {\omega _{m\left( {j-1} \right) } \left( {t_j -t_{j-1} } \right) +\,\varphi _{j-1} } \right) \\&-\,\lambda _m e^{-\lambda _m t_j }\cos \left( {\omega _{m\left( {j-1} \right) } \left( {t_j -t_{j-1} } \right) +\varphi _{j-1} } \right) \\&-\,\omega _{m\left( {j-1} \right) } e^{-\lambda _m t_{j-1} }\sin \varphi _{j-1} +\lambda _m e^{-\lambda _m t_{j-1} }\cos \varphi _{j-1} \\ g_0= & {} \frac{r_{2m} l_3 +al_4 L_m }{2L_m d_{1m} \lambda _m ^{2}a}\left( {\sum _{k=0}^n {J_{mk} } -i_{0m\left( n \right) } \sin \varphi _n } \right) \\ \end{aligned}$$
$$\begin{aligned} g_2= & {} -\frac{K\left( {al_1 +l_3 } \right) }{2d_{1m} \left( {\lambda _m ^{2}-a^{2}} \right) } +\frac{r_{2m} \left( {al_1 +l_3 } \right) }{2L_m d_{1m} \left( {\lambda _m ^{2}-a^{2}} \right) }\\&\quad \times \left[ {\sum _{k=0}^n {\Gamma _k } -\frac{1}{a}\sum _{k=0}^n {J_{mk} e^{at_k }} +\left( {\frac{\omega _{m\left( n \right) } \cos \varphi _n -a\sin \varphi _n }{a^{2}+\omega _{m\left( n \right) } ^{2}}+\frac{\sin \varphi _n }{a}} \right) i_{0m\left( n \right) } e^{at_n }} \right] \\ g_3= & {} \frac{i_{0m\left( n \right) } \left[ {ar_{2m} l_3 +a^{2}l_4 L_m +\omega _{m\left( n \right) } ^{2}\left( {l_4 L_m -l_1 r_{2m} } \right) -\left( {a^{2}+\omega _{m\left( n \right) } ^{2}} \right) \omega _{m\left( n \right) } l_2 L_m } \right] }{2L_m d_{1m} \left( {a^{2}+\omega _{m\left( n \right) } ^{2}} \right) \left( {\lambda _m ^{2}+\omega _{m\left( n \right) } ^{2}} \right) } \\ g_4= & {} \frac{-r_{2m} \left( {al_1 +l_3 } \right) i_{0m\left( n \right) } \omega _{m\left( n \right) } }{2L_m d_{1m} \left( {a^{2}+\omega _{m\left( n \right) } ^{2}} \right) \left( {\lambda _m ^{2}+\omega _{m\left( n \right) } ^{2}} \right) } \end{aligned}$$

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, Y., Wang, R. An improved neuronal energy model that better captures of dynamic property of neuronal activity. Nonlinear Dyn 91, 319–327 (2018). https://doi.org/10.1007/s11071-017-3871-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-017-3871-9

Keywords

Search

Navigation