Geometrically nonlinear vibration of laminated composite cylindrical thin shells with non-continuous elastic boundary conditions

Abstract

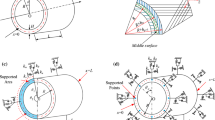
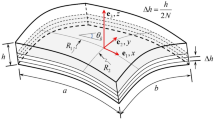
The geometrically nonlinear forced vibration response of non-continuous elastic-supported laminated composite thin cylindrical shells is investigated in this paper. Two kinds of non-continuous elastic supports are simulated by using artificial springs, which are point and arc constraints, respectively. By using a set of Chebyshev polynomials as the admissible displacement function, the nonlinear differential equation of motion of the shell subjected to periodic radial point loading is obtained through the Lagrange equations, in which the geometric nonlinearity is considered by using Donnell’s nonlinear shell theory. Then, these equations are solved by using the numerical method to obtain nonlinear amplitude–frequency response curves. The numerical results illustrate the effects of spring stiffness and constraint range on the nonlinear forced vibration of points-supported and arcs-supported laminated composite cylindrical shells. The results reveal that the geometric nonlinearity of the shell can be changed by adjusting the values of support stiffness and distribution areas of support, and the values of circumferential and radial stiffness have a more significant influence on amplitude–frequency response than the axial and torsional stiffness.

Appendices

Appendix A: The strains of mid-surface and curvature

$$\begin{aligned} \varepsilon _{x\left( 0 \right) }= & {} \frac{\partial u}{L\partial \xi }+\frac{1}{2}\left( {\frac{\partial w}{L\partial \xi }} \right) ^{2} \end{aligned}$$

(A.1)

$$\begin{aligned} \varepsilon _{\theta \left( 0 \right) }= & {} \frac{1}{R}\frac{\partial v}{\partial \theta }+\frac{w}{R}+\frac{1}{2}\left( {\frac{\partial w}{R\partial \theta }} \right) ^{2} \end{aligned}$$

(A.2)

$$\begin{aligned} \gamma _{x\theta \left( 0 \right) }= & {} \frac{1}{R}\frac{\partial u}{\partial \theta }+\frac{\partial v}{L\partial \xi }+\frac{\partial w}{L\partial \xi }\frac{1}{R}\frac{\partial w}{\partial \theta } \end{aligned}$$

(A.3)

$$\begin{aligned} \kappa _x= & {} -\frac{\partial ^{2}w}{L^{2}\partial \xi ^{2}} \end{aligned}$$

(A.4)

$$\begin{aligned} \kappa _\theta= & {} \frac{1}{R^{2}}\frac{\partial v}{\partial \theta }-\frac{1}{R^{2}}\frac{\partial ^{2}w}{\partial \theta ^{2}} \end{aligned}$$

(A.5)

$$\begin{aligned} \kappa _{x\theta }= & {} \frac{1}{RL}\frac{\partial v}{\partial \xi }-\frac{2}{RL}\frac{\partial ^{2}w}{\partial \xi \partial \theta } \end{aligned}$$

(A.6)

Appendix B: The stretching, coupling and bending stiffness coefficients

$$\begin{aligned} A_{ij}= & {} \sum _{p=1}^3 {{\overline{Q}} _{ij}^p } (h_{p+1} -h_p ) \end{aligned}$$

(B.1)

$$\begin{aligned} B_{ij}= & {} \frac{1}{2}\sum _{p=1}^3 {{\overline{Q}} _{ij}^p } (h_{p+1}^2 -h_p^2 ) \end{aligned}$$

(B.2)

$$\begin{aligned} D_{ij}= & {} \frac{1}{3}\sum _{p=1}^3 {{\overline{Q}} _{ij}^p } (h_{p+1}^3 -h_p^3 ) \end{aligned}$$

(B.3)

where p is the pth layer of the shell. In addition, all the $B_{ij}$ terms become zero for cylindrical shells laminated symmetrically with respect to their middle surfaces.

Appendix C: The mode vector U, V, and W

$$\begin{aligned} {{\bar{{\varvec{U}}}}^\mathrm{T}}= & {} \left[ {T^*\left( \xi \right) \cos \theta } \quad {T^*\left( \xi \right) \cos 2\theta } \cdots {T^*\left( \xi \right) \cos n\theta } \cdots {T^*\left( \xi \right) \cos N\theta } \quad 0 \right] \nonumber \\ \bar{{{\varvec{V}}}}^\mathrm{T}= & {} \left[ {T^*\left( \xi \right) \sin \theta } \quad {T^*\left( \xi \right) \sin 2\theta } \cdots {T^*\left( \xi \right) \sin n\theta } {\cdots } {T^*\left( \xi \right) \sin N\theta } \quad {0} \right] \nonumber \\ \bar{{{\varvec{W}}}}^\mathrm{T}= & {} \left[ {T^*\left( \xi \right) \cos \theta } \quad {T^*\left( \xi \right) \cos 2\theta } \cdots {T^*\left( \xi \right) \cos n\theta } \cdots {T^*\left( \xi \right) \cos N\theta } \quad {T^*\left( \xi \right) } \right] \end{aligned}$$

(C.1)

$T_m^*\left( \xi \right)$ is a Chebyshev polynomial of displacement components, $T_m^*\left( \xi \right) =T_m \left( {2\xi -1} \right) ,T_m \left( \xi \right)$ is the Chebyshev polynomials of the first kind, of which the recurrence expressions are given by

$$\begin{aligned} T_0 \left( \xi \right)= & {} 1,T_1 \left( \xi \right) =\xi ,T_{m+1} \left( \xi \right) \nonumber \\= & {} 2\xi T_m \left( \xi \right) -T_{m-1} \left( \xi \right) ,\left( {m\ge 2} \right) \end{aligned}$$

(C.2)

In the process of constructing Chebyshev polynomials, the polynomials are defined in the interval $[-\,1, 1]$, while $\xi \in \left[ {0, 1} \right]$, and the transformation of coordinates from $\xi$ to $2\xi -1$ is necessary.

Appendix D: Expressions for the mass matrix

The mass matrix Min Eq. (17) is expressed as

$$\begin{aligned} {\varvec{M}}=\left[ {{\begin{array}{lll} {{\varvec{M}}^{uu}}&{} &{} \\ &{} {{\varvec{M}}^{vv}}&{} \\ &{} &{} {{\varvec{M}}^{ww}} \\ \end{array} }} \right] \end{aligned}$$

(D.1)

where

$$\begin{aligned} {\varvec{M}}^{uu}= & {} \rho \hbox {HLR}\int _0^1 {\int _0^{2\pi } {\left[ \bar{{{\varvec{U}}}}\bar{{{\varvec{U}}}}^{\mathrm{T}}\right] } } \mathrm{d}\theta \mathrm{d}\xi \end{aligned}$$

(D.2)

$$\begin{aligned} {\varvec{M}}^{vv}= & {} \rho \hbox {HLR}\int _0^1 {\int _0^{2\pi } {\left[ \bar{{{\varvec{V}}}}\bar{{{\varvec{V}}}}^{\mathrm{T}}\right] } } \mathrm{d}\theta \mathrm{d}\xi \end{aligned}$$

(D.3)

$$\begin{aligned} {\varvec{M}}^{ww}= & {} \rho \hbox {HLR}\int _0^1 {\int _0^{2\pi } {\left[ \bar{{{\varvec{W}}}}\bar{{{\varvec{W}}}}^{\mathrm{T}}\right] } } \mathrm{d}\theta \mathrm{d}\xi \end{aligned}$$

(D.4)

Appendix E: Expressions of the stiffness matrix

The stiffness matrix Kin Eq. (17) is expressed as

$$\begin{aligned} {\varvec{K}}=\left[ {{\begin{array}{ccc} {{\varvec{K}}^{uu}}&{} {\frac{1}{2}{\varvec{K}}^{uv}}&{} {\frac{1}{2}{\varvec{K}}^{uw}} \\ {\frac{1}{2}{\varvec{K}}^{uv}}&{} {{\varvec{K}}^{vv}}&{} {\frac{1}{2}{\varvec{K}}^{vw}} \\ {\frac{1}{2}{\varvec{K}}^{uw}}&{} {\frac{1}{2}{\varvec{K}}^{vw}}&{} {{\varvec{K}}^{ww}} \\ \end{array} }} \right] \end{aligned}$$

(E.1)

where

$$\begin{aligned} {\varvec{K}}^{uu}= & {} \frac{L}{R}\int _0^1 \int _0^{2\pi } \nonumber \\&{\left( {A_{66} \frac{\partial \bar{{{\varvec{U}}}}}{\partial \theta }\frac{\partial \bar{{{\varvec{U}}}}^{\mathrm{T}}}{\partial \theta }+\frac{R^{2}A_{11} }{L^{2}}\frac{\partial \bar{{{\varvec{U}}}}}{\partial \xi }\frac{\partial \bar{{{\varvec{U}}}}^{\mathrm{T}}}{\partial \xi }} \right) } \hbox {d}\theta \hbox {d}\xi \end{aligned}$$

(E.2)

$$\begin{aligned} {\varvec{K}}^{uv}= & {} \frac{L}{R}\int _0^1 \int _0^{2\pi }\nonumber \\&\quad {\left( {\frac{2RA_{12} }{L}\frac{\partial \bar{{{\varvec{U}}}}}{\partial \xi }\frac{\partial \bar{{{\varvec{V}}}}^{\mathrm{T}}}{\partial \theta }+\frac{2RA_{66} }{L}\frac{\partial \bar{{{\varvec{U}}}}}{\partial \theta }\frac{\partial \bar{{{\varvec{V}}}}^{\mathrm{T}}}{\partial \xi }} \right) } \hbox {d}\theta \hbox {d}\xi \end{aligned}$$

(E.3)

$$\begin{aligned} {\varvec{K}}^{uw}= & {} \frac{L}{R}\int _0^1 {\int _0^{2\pi } {\left( {\left. {\frac{2RA_{12} }{L}\frac{\partial \bar{{{\varvec{U}}}}}{\partial \xi }\bar{{{\varvec{W}}}}^{T}} \right) } \right. } \hbox {d}\theta \hbox {d}\xi } \end{aligned}$$

(E.4)

$$\begin{aligned} {\varvec{K}}^{vv}= & {} \frac{L}{R}\int _0^1 \int _0^{2\pi } \left\{ \left( {A_{22} +\frac{D_{22} }{R^{2}}} \right) \frac{\partial \bar{{{\varvec{V}}}}}{\partial \theta }\frac{\partial \bar{{{\varvec{V}}}}^{\mathrm{T}}}{\partial \theta }\right. \nonumber \\&\left. +\left( \frac{R^{2}A_{66} }{L^{2}}+\frac{D_{66} }{L^{2}} \right) \frac{\partial \bar{{{\varvec{V}}}}}{\partial \xi }\frac{\partial \bar{{{\varvec{V}}}}^{T}}{\partial \xi } \right\} \hbox {d}\theta \hbox {d}\xi \end{aligned}$$

(E.5)

$$\begin{aligned} {\varvec{K}}^{vw}= & {} \frac{L}{R}\int _0^1 {\int _0^{2\pi }} \nonumber \\&\quad {\left( {2A_{22} \frac{\partial \bar{{{\varvec{V}}}}}{\partial \theta }\bar{{{\varvec{W}}}}^{T}-\frac{2D_{22} }{R^{2}}\frac{\partial \bar{{{\varvec{V}}}}}{\partial \theta }\frac{\partial ^{2}\bar{{{\varvec{W}}}}^{T}}{\partial \theta ^{2}}-\frac{4D_{66} }{L^{2}}\frac{\partial \bar{{{\varvec{V}}}}}{\partial \xi }\frac{\partial ^{2}\bar{{{\varvec{W}}}}^{\mathrm{T}}}{\partial \xi \partial \theta }} \right. } \nonumber \\&\quad -\left. {\frac{2D_{12} }{L^{2}}\frac{\partial \bar{{{\varvec{V}}}}}{\partial \theta }\frac{\partial ^{2}\bar{{{\varvec{W}}}}^{\mathrm{T}}}{\partial \xi ^{2}}} \right) \hbox {d}\theta \hbox {d}\xi \end{aligned}$$

(E.6)

$$\begin{aligned} {\varvec{K}}^{ww}= & {} \frac{L}{R}\int _0^1 \int _0^{2\pi }\nonumber \\&\left( {A_{22} \bar{{{\varvec{W}}}}\bar{{{\varvec{W}}}}^{\mathrm{T}}+\frac{R^{2}D_{11} }{L^{4}}\frac{\partial ^{2}\bar{{{\varvec{W}}}}}{\partial \xi ^{2}}\frac{\partial ^{2}\bar{{{\varvec{W}}}}^{\mathrm{T}}}{\partial \xi ^{2}}+\frac{D_{22} }{R^{2}}\frac{\partial ^{2}\bar{{{\varvec{W}}}}}{\partial \theta ^{2}}\frac{\partial ^{2}\bar{{{\varvec{W}}}}^{\mathrm{T}}}{\partial \theta ^{2}}} \right. \nonumber \\&+\frac{2D_{12} }{L^{2}}\frac{\partial ^{2}\bar{{{\varvec{W}}}}}{\partial \xi ^{2}}\frac{\partial ^{2}\bar{{{\varvec{W}}}}^{\mathrm{T}}}{\partial \theta ^{2}} \left. {+\frac{4D_{66} }{L^{2}}\frac{\partial ^{2}\bar{{{\varvec{W}}}}}{\partial \xi \partial \theta }\frac{\partial ^{2}\bar{{{\varvec{W}}}}^{\mathrm{T}}}{\partial \xi \partial \theta }} \right) \hbox {d}\theta \hbox {d}\xi \end{aligned}$$

(E.7)

Appendix F: Expressions of the nonlinear section

• (1) Nonlinear section $\hbox {U}_{{\varvec{Q}}}$ of laminated composite thin cylindrical shells

  $$\begin{aligned} {U_Q}= \frac{L}{R}\int _0^1 {\int _0^{2\pi } {\left\{ {\left. \begin{array}{l} \frac{{{A_{11}}{R^2}}}{{2{L^3}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial \bar{{\varvec{W}}}}}{{\partial \xi }}\frac{{\partial {{\bar{{\varvec{W}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{\bar{{\varvec{U}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{u}}}\\ + \frac{{{A_{12}}}}{{2L}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial \bar{{\varvec{W}}}}}{{\partial \theta }}\frac{{\partial {{\bar{{\varvec{W}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {U^T}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{u}}}\\ +\frac{{2{A_{66}}}}{L}{{\varvec{q}}_{\varvec{u}}}^\mathrm{T}\frac{{\partial \bar{{\varvec{U}}}}}{{\partial \theta }}\frac{{\partial {{\bar{{\varvec{W}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{\bar{{\varvec{W}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{{A_{12}}}}{{2L}}{{\varvec{q}}_{\varvec{u}}}^\mathrm{T}\frac{{\partial \bar{{\varvec{U}}}}}{{\partial \xi }}\frac{{\partial {{\bar{\mathrm{W}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{\bar{{\varvec{W}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{{A_{11}}{R^2}}}{{2{L^3}}}{{\varvec{q}}_{\varvec{u}}}^\mathrm{T}\frac{{\partial \bar{{\varvec{U}}}}}{{\partial \xi }}\frac{{\partial {{\bar{{\varvec{W}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{\bar{{\varvec{W}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{2{A_{12}}R}}{{{L^2}}}{{\varvec{q}}_{\varvec{v}}}^\mathrm{T}\frac{{\partial \bar{{\varvec{V}}}}}{{\partial \theta }}\frac{{\partial {{\bar{{\varvec{W}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{\bar{{\varvec{W}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}} \\ + \frac{{{A_{12}}R}}{{{L^2}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial \bar{{\varvec{W}}}}}{{\partial \xi }}\frac{{\partial {{\bar{{\varvec{W}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{\bar{{\varvec{V}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{v}}}\\ + \frac{{{A_{22}}}}{{2R}}{{\varvec{q}}_{\varvec{v}}}^\mathrm{T}\frac{{\partial \bar{{\varvec{V}}}}}{{\partial \theta }}\frac{{\partial {{\bar{{\varvec{W}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{\bar{{\varvec{W}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}} \\ + \frac{{2{A_{66}}R}}{{{L^2}}}{{\varvec{q}}_{\varvec{v}}}^\mathrm{T}\frac{{\partial \bar{{\varvec{V}}}}}{{\partial \xi }}\frac{{\partial {{\bar{{\varvec{W}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{\bar{\varvec{W}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{{A_{22}}}}{R}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial \bar{\varvec{W}}}}{{\partial \theta }}\frac{{\partial {{\bar{\varvec{W}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{\bar{\varvec{V}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{v}}}\\ + \frac{{{A_{12}}R}}{{2{L^2}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\bar{\varvec{W}}\frac{{\partial {{\bar{\varvec{W}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{\bar{\varvec{W}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{{A_{22}}}}{{2R}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\bar{\varvec{W}}\frac{{\partial {{\bar{\varvec{W}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{\bar{\varvec{W}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{{A_{12}}R}}{{2{L^2}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial {\varvec{W}}}}{{\partial \xi }}\frac{{\partial {{\bar{W}}^T}}}{{\partial \xi }}{q_w}{{\bar{W}}^T}{q_w}\\ + \frac{{{A_{22}}}}{{2R}}{q_w}^T\frac{{\partial \bar{W}}}{{\partial \theta }}\frac{{\partial {{\bar{W}}^T}}}{{\partial \theta }}{q_w}{{\bar{W}}^T}{q_w}\\ \frac{{{A_{11}}{R^2}}}{{4{L^4}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial \bar{\varvec{W}}}}{{\partial \xi }}\frac{{\partial {{\bar{\varvec{W}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial \bar{\varvec{W}}}}{{\partial \xi }}\frac{{\partial {{\bar{\varvec{W}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{{A_{22}}}}{{4{R^2}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial \bar{\varvec{W}}}}{{\partial \theta }}\frac{{\partial {{\bar{\varvec{W}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial \bar{\varvec{W}}}}{{\partial \theta }}\frac{{\partial {{\bar{\varvec{W}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{{A_{12}}}}{{4{L^2}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial \bar{\varvec{W}}}}{{\partial \theta }}\frac{{\partial {{\bar{\varvec{W}}}^T}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial \bar{\varvec{W}}}}{{\partial \xi }}\frac{{\partial {{\bar{\varvec{W}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{{A_{12}}}}{{4{L^2}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial \bar{\varvec{W}}}}{{\partial \xi }}\frac{{\partial {{\bar{\varvec{W}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial \bar{\varvec{W}}}}{{\partial \theta }}\frac{{\partial {{\bar{\varvec{W}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{{A_{66}}}}{{{L^2}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial \bar{\varvec{W}}}}{{\partial \xi }}\frac{{\partial {{\bar{\varvec{W}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial \bar{\varvec{W}}}}{{\partial \xi }}\frac{{\partial {{\bar{\varvec{W}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}} \end{array} \right\} } \right. \mathrm{{d}}\theta \mathrm{{d}}\xi } } \nonumber \\ \end{aligned}$$

  (F.1)

• (2) Element of nonlinear vector ${\varvec{Q}}'$

The nonlinear vector ${\varvec{Q}}'$ in Eqs. (17) is expressed as

$$\begin{aligned} {\varvec{Q}}' = \left[ {\begin{array}{l} {{{\varvec{Q}}^u}}\\ {{{\varvec{Q}}^v}}\\ {{{\varvec{Q}}^w}}\\ \end{array}} \right] \end{aligned}$$

(F.2)

where

$$\begin{aligned} {{\varvec{Q}}^u}= & {} \frac{L}{R}\int _0^1 {\int _0^{2\pi } {\left\{ {\left. \begin{array}{l} \frac{{{A_{11}}{R^2}}}{{2{L^3}}}\frac{{\partial {\bar{{\varvec{U}}}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \xi }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}} \\ + \frac{{{A_{11}}{R^2}}}{{2{L^3}}}\frac{{\partial {\bar{{\varvec{U}}}}}}{{\partial \xi }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{{A_{12}}}}{{2L}}\frac{{\partial {\bar{{\varvec{U}}}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \theta }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}} \\ + \frac{{2{A_{66}}}}{L}\frac{{\partial {\bar{{\varvec{U}}}}}}{{\partial \theta }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{{A_{12}}}}{{2L}}\frac{{\partial {\bar{{\varvec{U}}}}}}{{\partial \xi }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}} \end{array} \right\} } \right. {\mathrm{d}}\theta {\mathrm{d}}\xi } } \end{aligned}$$

(F.3)

$$\begin{aligned} {{\varvec{Q}}^v}= & {} \frac{L}{R}\int _0^1 {\int _0^{2\pi } {\left\{ {\left. \begin{array}{l} \frac{{2{A_{12}}R}}{{{L^2}}}\frac{{\partial {\bar{{\varvec{V}}}}}}{{\partial \theta }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}} \\ + \frac{{{A_{12}}R}}{{{L^2}}}\frac{{\partial {\bar{{\varvec{V}}}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \xi }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{{A_{22}}}}{R}\frac{{\partial {\bar{{\varvec{V}}}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \theta }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}} \\ + \frac{{{A_{22}}}}{{2R}}\frac{{\partial {\bar{{\varvec{V}}}}}}{{\partial \theta }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{2{A_{66}}R}}{{{L^2}}}\frac{{\partial {\bar{{\varvec{V}}}}}}{{\partial \xi }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}} \end{array} \right\} } \right. \mathrm{{d}}\theta \mathrm{{d}}\xi } } \end{aligned}$$

(F.4)

$$\begin{aligned} {{\varvec{Q}}^w}= & {} \frac{L}{R}\int _0^1 \int _0^{2\pi }\nonumber \\&\times \left\{ \begin{array}{l} \frac{{{A_{12}}R}}{{2{L^2}}}\left( {{\bar{{\varvec{W}}}}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}+ 2{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}{\bar{{\varvec{W}}}}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \xi }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}} \right) \\ + \frac{{{A_{22}}}}{{2R}}\left( {{\bar{{\varvec{W}}}}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}} + 2{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}{\bar{{\varvec{W}}}}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \theta }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}} \right) \\ + \frac{{{A_{12}}R}}{{2{L^2}}}\left( {2\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \xi }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}{{{\bar{{\varvec{W}}}}}^\mathrm{T}}{{\varvec{q}}_{\varvec{w}}} + {{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial {\varvec{W}}}}{{\partial \xi }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}{\bar{{\varvec{W}}}}} \right) \\ + \frac{{{A_{22}}}}{{2R}}\left( 2\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \theta }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}{{{\bar{{\varvec{W}}}}}^\mathrm{T}}{{\varvec{q}}_{\varvec{w}}} + {{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial {{\varvec{W}}}}}{{\partial \theta }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}{\bar{{\varvec{W}}}} \right) \\ + \frac{{{A_{11}}{R^2}}}{{2{L^4}}}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \xi }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \xi }}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{{A_{22}}}}{{2{R^2}}}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \theta }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \theta }}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{{A_{12}}}}{{4{L^2}}}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \theta }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \xi }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{{A_{12}}}}{{4{L^2}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \theta }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \xi }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{{A_{12}}}}{{4{L^2}}}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \xi }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \theta }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{{A_{12}}}}{{4{L^2}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \xi }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}{{\varvec{q}}_{\varvec{w}}}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \theta }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}}\\ + \frac{{{A_{66}}}}{{{L^2}}}\left( {\frac{{\partial \bar{\varvec{W}}}}{{\partial \xi }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }} + \frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \theta }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \xi }}} \right) {{\varvec{q}}_{\varvec{w}}}{{\varvec{q}}_{\varvec{w}}}^\mathrm{T}\frac{{\partial {\bar{{\varvec{W}}}}}}{{\partial \xi }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}}}{{\partial \theta }}{{\varvec{q}}_{\varvec{w}}} \end{array} \right\} \mathrm{d}\theta \mathrm{d}\xi \nonumber \\ \end{aligned}$$

(F.5)

Appendix G: Expressions of the spring stiffness matrix

The nonlinear vector ${{\varvec{Q}}}'$ in Eqs. (17) is expressed as

$$\begin{aligned} {{\varvec{K}}_{\mathrm{spr}}} = \left[ {\begin{array}{lll} {{\varvec{K}}_{\mathrm{spr}}^{uu}}&{}&{}\\ &{}{{\varvec{K}}_{\mathrm{spr}}^{vv}}&{}\\ &{}&{}{{\varvec{K}}_{\mathrm{spr}}^{ww}} \end{array}} \right] \end{aligned}$$

(G.1)

• (a) The spring stiffness matrix ${\varvec{K}}_\mathrm{spr}$ of the arcs-supported boundary condition

  $$\begin{aligned} K_{\mathrm{spr}}^{uu}= & {} \sum \limits _{\mathrm{{s}} = 1}^{\mathrm{NS}} {\int _{{\theta _s}}^{\theta {'_s}} {\left( {k_{u,s}^0{\bar{{\varvec{U}}}}\left( 0 \right) {{{\bar{{\varvec{U}}}}}^\mathrm{T}}\left( 0 \right) + k_{u,s}^1{\bar{{\varvec{U}}}}\left( 1 \right) {{{\bar{{\varvec{U}}}}}^\mathrm{T}}\left( 1 \right) } \right) R} \mathrm{{d}}\theta } \nonumber \\ \end{aligned}$$

  (G.2)
  $$\begin{aligned} K_{\mathrm{spr}}^{vv}= & {} \sum \limits _{s = 1}^{\mathrm{NS}} {\int _{{\theta _s}}^{\theta {'_s}} {\left( {k_{v,s}^0{\bar{{\varvec{V}}}}\left( 0 \right) {{{\bar{{\varvec{V}}}}}^\mathrm{T}}\left( 0 \right) + k_{v,s}^1{\bar{{\varvec{V}}}}\left( 1 \right) {{{\bar{{\varvec{V}}}}}^\mathrm{T}}\left( 1 \right) } \right) R} \mathrm{{d}}\theta } \nonumber \\ \end{aligned}$$

  (G.3)
  $$\begin{aligned} K_{\mathrm{spr}}^{ww}= & {} \sum \limits _{\mathrm{s} = 1}^{\mathrm{NS}} {\int _{{\theta _s}}^{\theta {'_s}} {\left( {k_{w,s}^0{\bar{{\varvec{W}}}}\left( 0 \right) {{{\bar{{\varvec{W}}}}}^\mathrm{T}}\left( 0 \right) + \frac{{k_{\theta ,s}^0}}{{{L^2}}}\frac{{\partial {\bar{{\varvec{W}}}}\left( 0 \right) }}{{\partial \xi }}\frac{{\partial {{{\bar{{\varvec{W}}}}}^\mathrm{T}}\left( 0 \right) }}{{\partial \xi }}} \right. } } \nonumber \\&+\,k_{w,s}^1{\bar{{\varvec{W}}}}\left( 1 \right) {{{\bar{{\varvec{W}}}}}^\mathrm{T}}\left( 1 \right) \nonumber \\&\left. { +\,\frac{{k_{\theta ,s}^1}}{{{L^2}}}\frac{{\partial {\bar{{\varvec{W}}}}\left( 1 \right) }}{{\partial \xi }}\frac{{\partial {\bar{{\varvec{W}}}}{{\left( 1 \right) }^\mathrm{T}}}}{{\partial \xi }}} \right) R\mathrm{{d}}\theta \end{aligned}$$

  (G.4)

• (b) The spring stiffness matrix ${\varvec{K}}_\mathrm{spr}$ of the points-supported boundary condition

  $$\begin{aligned} {K_{\mathrm{spr}}^{uu}}= & {} \sum \limits _{p = 1}^{\mathrm{NA}} \left( {k'^{o}_{\mathrm{u},p}}{\bar{{\varvec{U}}}}\left( {0,{\theta _{p}}} \right) {{{\bar{{\varvec{U}}}}}^\mathrm{T}}\left( {0,{\theta _{p}}} \right) \right. \nonumber \\&\left. +\,{k'^{1}_{u,p}}{\bar{{\varvec{U}}}}\left( {0,{\theta _{p}}} \right) {{{\bar{{\varvec{U}}}}}^\mathrm{T}}\left( {0,{\theta _{p}}} \right) \right) \end{aligned}$$

  (G.5)
  $$\begin{aligned} {K^{vv}_\mathrm{spr}}= & {} \sum \limits _{p = 1}^{\mathrm{NA}} \left( {k'^{0}_{v,p}}{\bar{{\varvec{V}}}}\left( {0,{\theta _p}} \right) {{{\bar{V}}}^\mathrm{T}}\left( {0,{\theta _p}} \right) \right. \nonumber \\&\left. +\, {k'^{1}_{v,p}}{\bar{{\varvec{V}}}}\left( {1,{\theta _p}} \right) {{{\bar{{\varvec{V}}}}}^\mathrm{T}}\left( {1,{\theta _p}} \right) \right) \end{aligned}$$

  (G.6)
  $$\begin{aligned} {K^{ww}_\mathrm{spr}}= & {} \sum \limits _{p = 1}^{\mathrm{NA}} \left( {k'^{0}_{w,p}}{\bar{{\varvec{W}}}}\left( {0,{\theta _p}} \right) {{{\bar{{\varvec{W}}}}}^\mathrm{T}}\left( {0,{\theta _p}} \right) \right. \nonumber \\&\left. +\, \frac{{{k'^{0}_{\theta ,s}}}}{{{L^2}}}\frac{{\partial {\bar{{\varvec{W}}}}\left( {0,{\theta _p}} \right) }}{{\partial \xi }}\frac{{\partial {\bar{{\varvec{W}}}}{{\left( {0,{\theta _p}} \right) }^\mathrm{T}}}}{{\partial \xi }} \right. \nonumber \\&+\,{k}_{w,p}^{\prime 1}{\bar{{\varvec{W}}}}\left( {1,{\theta _p}} \right) {{{\bar{{\varvec{W}}}}}^\mathrm{T}}\left( {1,{\theta _p}} \right) \nonumber \\&\left. +\,\frac{{{k^\prime {1}_{\theta ,p}}}}{{{L^2}}}\frac{{\partial {\bar{{\varvec{W}}}}\left( {1,{\theta _p}} \right) }}{{\partial \xi }}\frac{{\partial {\bar{{\varvec{W}}}}{{\left( {1,{\theta _p}} \right) }^\mathrm{T}}}}{{\partial \xi }} \right) \nonumber \\ \end{aligned}$$

  (G.7)

Appendix H: Expressions of the damping matrix

Rayleigh damping C is considered during analysis of the vibration response of laminated cylindrical shells, and it is given as follows

$$\begin{aligned} {\varvec{C}} = \alpha {{\varvec{M}}} + \beta \left( {{\varvec{K}}} + {{{\varvec{K}}}_{spr}} \right) \end{aligned}$$

(8)

where$\alpha , \beta$ are the damping parameters

$$\begin{aligned} \alpha= & {} 2 {\left( {\frac{{{\xi _2}}}{{\omega _2^2}} - \frac{{{\xi _1}}}{{\omega _1^2}}} \right) }\bigg / {\left( {\frac{1}{{\omega _2^2}} - \frac{1}{{\omega _1^2}}} \right) } \beta \nonumber \\= & {} 2{{\left( {{\xi _2}\omega _2^{} - {\xi _1}\omega _1^{}} \right) } {\bigg / } {\left( {\omega _2^2 - \omega _1^2} \right) }} \end{aligned}$$

(9)

where $\omega _1, \omega _2$ are the first-order and second-order natural frequencies of laminated cylindrical shell, and $\xi _1,\xi _2$ are the damp coefficient.