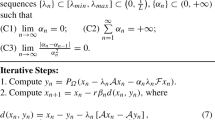Skip to main content
SpringerLink
Log in

Strong convergence of a double projection-type method for monotone variational inequalities in Hilbert spaces

  • Published:
Journal of Fixed Point Theory and Applications Aims and scope Submit manuscript

Abstract

We introduce a projection-type algorithm for solving monotone variational inequality problems in real Hilbert spaces without assuming Lipschitz continuity of the corresponding operator. We prove that the whole sequence of iterates converges strongly to a solution of the variational inequality. The method uses only two projections onto the feasible set in each iteration in contrast to other strongly convergent algorithms which either require plenty of projections within a step size rule or have to compute projections on possibly more complicated sets. Some numerical results illustrate the behavior of our method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Apostol, R.Y., Grynenko, A.A., Semenov, V.V.: Iterative algorithms for monotone bilevel variational inequalities. J. Comput. Appl. Math. 107, 3–14 (2012)

    Google Scholar 

  2. Aubin, J.-P., Ekeland, I.: Applied Nonlinear Analysis. Wiley, New York (1984)

    MATH  Google Scholar 

  3. Baiocchi, C., Capelo, A.: Variational and Quasivariational Inequalities. Applications to Free Boundary Problems. Wiley, New York (1984)

    MATH  Google Scholar 

  4. Bauschke, H.H., Combettes, P.L.: Convex Analysis and Monotone Operator Theory in Hilbert Spaces, CMS Books in Mathematics. Springer, New York (2011)

    Book  MATH  Google Scholar 

  5. Bauschke, H.H., Combettes, P.L.: A weak-to-strong convergence principle for Fej\(\acute{e}\)r-monotone methods in Hilbert spaces. Math. Oper. Res. 26, 248–264 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  6. Bello Cruz, J.Y., Iusem, A.N.: A strongly convergent direct method for monotone variational inequalities in Hilbert spaces. Numer. Funct. Anal. Optim. 30, 23–36 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  7. Bello Cruz, J.Y., Iusem, A.N.: An explicit algorithm for monotone variational inequalities. Optimization 61, 855–871 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  8. Bergounioux, M., Haddou, M., Hintermüller, M., Kunisch, K.: A comparison of a Moreau-Yosida based active set strategy and interior point methods for constrained optimal control problems. SIAM J. Optim. 11, 495–521 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  9. Browder, F.: Nonlinear monotone operators and convex sets in Banach spaces. Bull. Am. Math. Soc. 71, 780–785 (1965)

    Article  MathSciNet  MATH  Google Scholar 

  10. Cegielski, A.: Iterative methods for fixed point problems in Hilbert spaces. In: Lecture Notes in Mathematics, vol. 2057. Springer, Berlin (2013). https://doi.org/10.1007/978-3-642-30901-4

  11. Ceng, L.C., Hadjisavvas, N., Wong, N.-C.: Strong convergence theorem by a hybrid extragradient-like approximation method for variational inequalities and fixed point problems. J. Glob. Optim. 46, 635–646 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  12. Ceng, L.C., Schaible, S., Yao, J.C.: Strong convergence of iterative algorithms for variational inequalities in Banach spaces. J. Optim. Theory Appl. 141(2), 265–283 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  13. Ceng, L.C., Teboulle, M., Yao, J.C.: Weak convergence of an iterative method for pseudomonotone variational inequalities and fixed-point problems. J. Optim. Theory Appl. 146, 19–31 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  14. Censor, Y., Gibali, A., Reich, S.: Strong convergence of subgradient extragradient methods for the variational inequality problem in Hilbert space. Optim. Methods Softw. 26, 827–845 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  15. Censor, Y., Gibali, A., Reich, S.: The subgradient extragradient method for solving variational inequalities in Hilbert space. J. Optim. Theory Appl. 148, 318–335 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  16. Censor, Y., Gibali, A., Reich, S.: Extensions of Korpelevich’s extragradient method for the variational inequality problem in Euclidean space. Optimization 61, 1119–1132 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  17. Denisov, S., Semenov, V., Chabak, L.: Convergence of the modified extragradient method for variational inequalities with non-Lipschitz operators. Cybern. Syst. Anal. 51, 757–765 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  18. Facchinei, F., Pang, J.-S.: Finite-dimensional variational inequalities and complementarity problems, vol. II. In: Springer Series in Operations Research. Springer, New York (2003). https://doi.org/10.1007/b97543

  19. Glowinski, R., Lions, J.-L., Trémolières, R.: Numerical Analysis of Variational Inequalities. North-Holland, Amsterdam (1981)

    MATH  Google Scholar 

  20. Goebel, K., Reich, S.: Uniform Convexity, Hyperbolic Geometry, and Nonexpansive Mappings. Marcel Dekker, New York (1984)

    MATH  Google Scholar 

  21. Harker, P.T., Pang, J.-S.: A damped-Newton method for the linear complementarity problem. In: Allgower G, Georg K, (eds) Computational Solution of Nonlinear Systems of Equations, Lectures in Appl. Math., vol. 26, pp. 265–284. AMS, Providence (1990)

  22. Hartman, Ph, Stampacchia, G.: On some non linear elliptic differential functional equations. Acta Math. 115, 271–310 (1966)

    Article  MathSciNet  MATH  Google Scholar 

  23. He, B.-S., Yang, Z.-H., Yuan, X.-M.: An approximate proximal-extragradient type method for monotone variational inequalities. J. Math. Anal. Appl. 300, 362–374 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  24. Hieu, D.V., Anh, P.K., Muu, L.D.: Modified hybrid projection methods for finding common solutions to variational inequality problems. Comput. Optim. Appl. 66, 75–96 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  25. Iusem, A.N., Gárciga, R.: Otero; Inexact versions of proximal point and augmented Lagrangian algorithms in Banach spaces. Numer. Funct. Anal. Optim. 22, 609–640 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  26. Iusem, A.N., Nasri, M.: Korpelevich’s method for variational inequality problems in Banach spaces. J. Global Optim. 50, 59–76 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  27. Iusem, A.N., Svaiter, B.F.: A variant of Korpelevich’s method for variational inequalities with a new search strategy. Optimization 42, 309–321 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  28. Khobotov, E.N.: Modification of the extragradient method for solving variational inequalities and certain optimization problems. USSR Comput. Math. Math. Phys. 27, 120–127 (1989)

    Article  MATH  Google Scholar 

  29. Kinderlehrer, D., Stampacchia, G.: An Introduction to Variational Inequalities and Their Applications. Academic Press, New York (1980)

    MATH  Google Scholar 

  30. Konnov, I.V.: Combined Relaxation Methods for Variational Inequalities. Springer, Berlin (2001)

    Book  MATH  Google Scholar 

  31. Korpelevich, G.M.: The extragradient method for finding saddle points and other problems. Ékon. Mat. Metody 12, 747–756 (1976)

    MathSciNet  MATH  Google Scholar 

  32. Kraikaew, R., Saejung, S.: Strong convergence of the Halpern subgradient extragradient method for solving variational inequalities in Hilbert spaces. J. Optim. Theory Appl. 163, 399–412 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  33. Lyashko, S.I., Semenov, V.V., Voitova, T.A.: Low-cost modification of Korpelevich’s method for monotone equilibrium problems. Cybern. Syst. Anal. 47, 631–639 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  34. Maingé, P.-E.: The viscosity approximation process for quasi-nonexpansive mappings in Hilbert spaces. Comput. Math. Appl. 59, 74–79 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  35. Maingé, P.-E.: Numerical approach to monotone variational inequalities by a one-step projected reflected gradient method with line-search procedure. Comput. Math. Appl. 72(3), 720–728 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  36. Maingé, P.-E., Gobinddass, M.L.: Convergence of one-step projected gradient methods for variational inequalities. J. Optim. Theory Appl. 171, 146–168 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  37. Malitsky, YuV: Projected reflected gradient methods for monotone variational inequalities. SIAM J. Optim. 25, 502–520 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  38. Malitsky, YuV, Semenov, V.V.: An extragradient algorithm for monotone variational inequalities. Cybern. Syst. Anal. 50, 271–277 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  39. Malitsky, YuV, Semenov, V.V.: A hybrid method without extrapolation step for solving variational inequality problems. J. Global Optim. 61, 193–202 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  40. Nadezhkina, N., Takahashi, W.: Strong convergence theorem by a hybrid method for nonexpansive mappings and Lipschitz-continuous monotone mappings. SIAM J. Optim. 16, 1230–1241 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  41. Nagurney, A.: Network Economics: A Variational Inequality Approach. Kluwer Academic Publishers, Dordrecht (1999)

    Book  MATH  Google Scholar 

  42. Noor, M.A.: Modified projection method for pseudomonotone variational inequalities. Appl. Math. Lett. 15, 315–320 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  43. Popov, L.D.: A modification of the Arrow–Hurwicz method for finding saddle points. Math. Notes 28, 845–848 (1980)

    Article  MATH  Google Scholar 

  44. Solodov, M.V., Svaiter, B.F.: A new projection method for variational inequality problems. SIAM J. Control Optim. 37, 765–776 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  45. Takahashi, W.: Nonlinear Functional Analysis. Yokohama Publishers, Yokohama (2000)

    MATH  Google Scholar 

  46. Tseng, P.: A modified forward-backward splitting method for maximal monotone mappings. SIAM J. Control Optim. 38, 431–446 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  47. Xu, H.-K.: Iterative algorithms for nonlinear operators. J. Lond. Math. Soc. 66, 240–256 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  48. Yao, Y., Postolache, M.: Iterative methods for pseudomonotone variational inequalities and fixed-point problems. J. Optim. Theory Appl. 155, 273–287 (2012)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Author notes

  1. Yekini Shehu

    Present address: Institute of Mathematics, University of Würzburg, Campus Hubland Nord, Emil-Fischer-Str.30, 97074, Würzburg, Germany

Authors and Affiliations

  1. Institute of Mathematics, University of Würzburg, Campus Hubland Nord, Emil-Fischer-Str.30, 97074, Würzburg, Germany

    Christian Kanzow

  2. Department of Mathematics, University of Nigeria, Nsukka, Nigeria

    Yekini Shehu

Authors
  1. Christian Kanzow
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yekini Shehu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yekini Shehu.

Additional information

The research of this author is supported by the Alexander von Humboldt Foundation (Grant no. 1163904).

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Kanzow, C., Shehu, Y. Strong convergence of a double projection-type method for monotone variational inequalities in Hilbert spaces. J. Fixed Point Theory Appl. 20, 51 (2018). https://doi.org/10.1007/s11784-018-0531-8

Download citation

  • Published:

  • DOI: https://doi.org/10.1007/s11784-018-0531-8

Mathematics Subject Classification

Keywords

Search

Navigation