Skip to main content
SpringerLink
Log in

Solving an inverse initial-boundary-value problem using basis function method

  • Published:
Computational and Applied Mathematics Aims and scope Submit manuscript

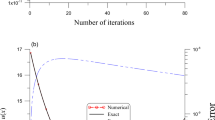
Abstract

In this paper, we will first study the existence and uniqueness of the solution of an inverse initial-boundary-value problem, via an auxiliary problem. Furthermore, we propose a stable numerical approach based on the use of the solution to the auxiliary problem as a basis function for solving this problem in the presence of noisy data. Also note that the inverse problem has a unique solution, but this solution is unstable and hence the problem is ill-posed. This instability is overcome using the Tikhonov regularization method. The effectiveness of the algorithm is illustrated by numerical example.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  • Abtahi M, Pourgholi R, Shidfar A (2011) Existence and uniqueness of solution for a two dimensional nonlinear inverse diffusion problem. Nonlinear Anal (TMA) 74:2462–2467

    Article  MathSciNet  MATH  Google Scholar 

  • Alves CJS, Chen CS, Saler B (2002) The method of fundamental solutions for solving Poisson problems. International series on advances in boundary elements. Boundary Elements XXIV 13:67–76

    MathSciNet  Google Scholar 

  • Anger G (1990) Inverse problems in differential equations. Plenum Press, New York

    Google Scholar 

  • Bard A, Faulkner LR (1980) Electrochemical methods. Wiley, New York

    Google Scholar 

  • Bazant MZ, Chu KT, Bayly BJ (2005) Current voltage relations for electrochemical thin film. SIAM J Appl Maths 65:1463–1484

    Article  MathSciNet  MATH  Google Scholar 

  • Beck JV, Blackwell B, St CR (1985) Clair, inverse heat conduction: ill-posed problems. Wiley-Interscience, New York

    Google Scholar 

  • Beck JV, Blackwell B, Haji-sheikh A (1996) Comparison of some inverse heat conduction methods using experimental data. Int J Heat Mass Transf 3:3649–3657

    Article  Google Scholar 

  • Bhat YS, Moskow S (2007) Linearization of a nonlinear periodic boundary condition related to corrosion modeling. J Comput Math 25:645–660

    MathSciNet  MATH  Google Scholar 

  • Biegert M, Warma M (2009) The heat equation with nonlinear generalized Robin boundary conditions. J Differ Equ 247:1949–1979

    Article  MathSciNet  MATH  Google Scholar 

  • Cabeza JMG, Garcia JAM, Rodriguez AC (2005) A sequential algorithm of inverse heat conduction problems using singular value decomposition. Int J Therm Sci 4:235–244

    Article  Google Scholar 

  • Cannon JR (1984) The one-dimensional heat equation. Addison Wesley, Reading

    Book  MATH  Google Scholar 

  • Hansen PC (1992) Analysis of discrete Ill-posed problems by means of the L-curve. SIAM Rev 34:561–580

    Article  MathSciNet  MATH  Google Scholar 

  • Hansen PC (1998) Rank-deficient and discrete ill-posed problems. SIAM, Philadelphia

    Book  Google Scholar 

  • Hömberg D, Krumbiegel K, Rehberg J (2013) Boundary coefficient control—a maximal parabolic regularity approach (Preprint)

  • Hon YC, Wei T (2004) A fundamental solution method for inverse heat conduction problem. Eng Anal Boundary Elem 28:489–495

    Article  MATH  Google Scholar 

  • Lawson CL, Hanson RJ (1974) Solving least squares problems, Philadelphia, PA: SIAM, 1995. First published by Prentice-Hall (1974).

  • Murio DA (1993) The mollification method and the numerical solution of ill-posed problems. Wiley-Interscience, NewYork

    Book  Google Scholar 

  • Murio DC, Paloschi JR (1988) Combined mollification-future temperature procedure for solution of inverse heat conduction problem. J comput Appl Math 23:235–244

    Article  MathSciNet  MATH  Google Scholar 

  • Molhem H, Pourgholi R (2008) A numerical algorithm for solving a one-dimensional inverse heat conduction problem. J Math Stat 4:60–63

    Article  MathSciNet  Google Scholar 

  • Newman J (1968) Engineering design of electrochemical systems. Ind Eng Chem Fundam 60:12–27

    Google Scholar 

  • Newman J (1973) Electroanalytical chemistry, vol 6. Marcel Dekker, New York

    Google Scholar 

  • Pazy A (1983) Semigroups of linear operators and applications to partial differential equations, Applied Mathematical Sciences, 44. Springer, New York

    Book  Google Scholar 

  • Pourgholi R, Azizi N, Gasimov YS, Aliev F, Khalafi HK (2009) Removal of numerical instability in the solution of an inverse heat conduction problem. Commun Nonlinear Sci Numer Simul 14:2664–2669

    Article  MathSciNet  MATH  Google Scholar 

  • Pourgholi R, Rostamian M (2010) A numerical technique for solving IHCPs using Tikhonov regularization method. Appl Math Model 34:2102–2110

    Article  MathSciNet  MATH  Google Scholar 

  • Pourgholi R, Rostamian M, Emamjome M (2010) A numerical method for solving a nonlinear inverse parabolic problem. Inverse Probl Sci Eng 18:1151–1164

    Article  MathSciNet  MATH  Google Scholar 

  • Rousar I, Micka K, Kimla A (1986) Electrochemical engineering. 1. Elsevier, Amsterdam

    Google Scholar 

  • Shidfar A, Azary H (1997) Nonlinear parabolic problems. Nonlinear Anal Theory Methods Appl 30:4823–4832

    Article  MathSciNet  MATH  Google Scholar 

  • Shidfar A, Pourgholi R (2006a) Numerical approximation of solution of an inverse heat conduction problem based on Legendre polynomials. Appl. Math. Comput 175:1366–1374

    Article  MathSciNet  MATH  Google Scholar 

  • Shidfar A, Pourgholi R, Ebrahimi M (2006b) A Numerical Method for Solving of a Nonlinear Inverse Diffusion Problem. Comput Math Appl 52:1021–1030

    Article  MathSciNet  MATH  Google Scholar 

  • Tikhonov AN, Arsenin VY (1977) On the solution of ill-posed problems. Wiley, New York

    Google Scholar 

  • Yan L, Yang FL, Fu CL (2009) A meshless method for solving an inverse spacewise-dependent heat source problem. J Comput Phys 228:123–136

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Mathematics and Computer Sciences, Damghan University, 36715-364, Damghan, Iran

    Reza Pourgholi, Amin Esfahani, Hamideh Rahimi & S. Hashem Tabasi

  2. Department of Mathematics, IME-USP, Rua do Matão 1010, Cidade Universitária, 05508-090 , São Paulo, SP, Brazil

    Amin Esfahani

Authors
  1. Reza Pourgholi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Amin Esfahani
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Hamideh Rahimi
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. S. Hashem Tabasi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Reza Pourgholi.

Additional information

Communicated by Ruben Spies.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Pourgholi, R., Esfahani, A., Rahimi, H. et al. Solving an inverse initial-boundary-value problem using basis function method. Comp. Appl. Math. 32, 27–40 (2013). https://doi.org/10.1007/s40314-013-0005-y

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40314-013-0005-y

Keywords

Mathematics Subject Classification (2000)

Search

Navigation