Skip to main content
SpringerLink
Log in

Local maximum entropy approximation-based streamline upwind Petrov–Galerkin meshfree method for convection–diffusion problem

  • Technical Paper
  • Published:
Journal of the Brazilian Society of Mechanical Sciences and Engineering Aims and scope Submit manuscript

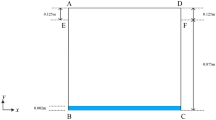
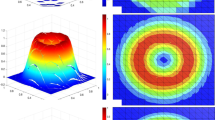
Abstract

Local maximum entropy (LME) meshfree basis functions are among the few approximants that possess the Kronecker delta property on the boundary, which enable to impose the essential boundary conditions directly like FEM. This study presents the potential of a meshfree method based on LME approximation for the Convection–Diffusion problem via well-celebrated SUPG. The present LME based Streamline Upwind Petrov–Galerkin meshfree method (SUPGM) benefits from the advantages emanated from both methods. LME approximants accounts for the disposal of the elements and direct imposition of boundary conditions and the SUPGM technique to deal with the issues associated with the non-self-adjoint convective term. Two standard benchmark problems are considered to validate the LME-SUPGM. The effect of different priors and radius of support that defines the LME basis are studied to test their importance in the present method. It is found that a balance between stability and accuracy is possible with ease by tuning the effective radius of support for LME for the given problem. The present method converges faster than FEM-SUPG and provides a smooth solution relatively.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15

Similar content being viewed by others

References

  1. Belytschko T, Lu YY, Gu L (1994) Element-free Galerkin methods. Int J Numer Methods Eng 37:229–256. https://doi.org/10.1002/nme.1620370205

    Article  MathSciNet  MATH  Google Scholar 

  2. Atluri SN, Zhu T (1998) A new Meshless local Petrov-Galerkin (MLPG) approach in computational mechanics. Comput Mech 22:117–127. https://doi.org/10.1007/s004660050346

    Article  MathSciNet  MATH  Google Scholar 

  3. Ghehsareh HR, Raei M, Zaghian A (2019) Application of meshless local Petrov–Galerkin technique to simulate two-dimensional time-fractional Tricomi-type problem. J Braz Soc Mech Sci Eng 41:252. https://doi.org/10.1007/s40430-019-1749-0

    Article  Google Scholar 

  4. Fries T-P, Matthies HG (2004) Classification and Overview of Meshfree Methods. Inform der Tech Univ Braunschweig 2003–03

  5. Zhang T, Li X (2018) A generalized element-free Galerkin method for Stokes problem. Comput Math Appl 75:3127–3138. https://doi.org/10.1016/j.camwa.2018.01.035

    Article  MathSciNet  MATH  Google Scholar 

  6. Sukumar N (2004) Construction of polygonal interpolants: a maximum entropy approach. Int J Numer Methods Eng 61:2159–2181. https://doi.org/10.1002/nme.1193

    Article  MathSciNet  MATH  Google Scholar 

  7. Sukumar N, Wright RW (2007) Overview and construction of meshfree basis functions : from moving least squares to entropy approximants, pp 181–205. https://doi.org/10.1002/nme

  8. Arroyo M, Ortiz M (2006) Local maximum-entropy approximation schemes : a seamless bridge between finite elements and meshfree methods. Int J Numer Methods Eng 63:2167–2202. https://doi.org/10.1002/nme.1534

    Article  MathSciNet  MATH  Google Scholar 

  9. Masud A, Khurram RA (2004) A multiscale/stabilized finite element method for the advection–diffusion equation. Comput Methods Appl Mech Eng 193:1997–2018. https://doi.org/10.1016/j.cma.2003.12.047

    Article  MATH  Google Scholar 

  10. Brezzi F, Franca LP, Hughes TJR, Russo A (1997) b = ∝ g. Comput Methods Appl Mech Eng 145:329–339. https://doi.org/10.1016/S0045-7825(96)01221-2

    Article  MATH  Google Scholar 

  11. John V, Knobloch P, Novo J (2018) Finite elements for scalar convection-dominated equations and incompressible flow problems: a never ending story? Comput Vis Sci 19:47–63. https://doi.org/10.1007/s00791-018-0290-5

    Article  MathSciNet  Google Scholar 

  12. Brooks AN, Hughes TJR (1982) Streamline upwind/Petrov–Galerkin formulations for convection dominated flows with particular emphasis on the incompressible Navier–Stokes equations. Comput Methods Appl Mech Eng 32:199–259. https://doi.org/10.1016/0045-7825(82)90071-8

    Article  MathSciNet  MATH  Google Scholar 

  13. Lima RC, Mesquita ALA, Blanco CJC et al (2014) On the application of SUPG/θ-method in 2D advection–diffusion-reaction simulation. J Braz Soc Mech Sci Eng 36:591–603. https://doi.org/10.1007/s40430-013-0099-6

    Article  Google Scholar 

  14. Franca LP, Frey SL, Hughes TJR (1992) Stabilized finite element methods: I. Application to the advective-diffusive model. Comput Methods Appl Mech Eng 95:253–276. https://doi.org/10.1016/0045-7825(92)90143-8

    Article  MathSciNet  MATH  Google Scholar 

  15. Hughes TJR, Scovazzi G, Franca LP (2017) Multiscale and stabilized methods. In: Encyclopedia of computational mechanics second edition. American Cancer Society, pp 1–64

  16. Brezzi F, Marini D, Russo A (1998) Applications of the pseudo residual-free bubbles to the stabilization of convection-diffusion problems. Comput Methods Appl Mech Eng 166:51–63. https://doi.org/10.1016/S0045-7825(98)00082-6

    Article  MathSciNet  MATH  Google Scholar 

  17. Hughes TJR (1995) Multiscale phenomena: Green’s functions, the Dirichlet-to-Neumann formulation, subgrid scale models, bubbles and the origins of stabilized methods. Comput Methods Appl Mech Eng 127:387–401. https://doi.org/10.1016/0045-7825(95)00844-9

    Article  MathSciNet  MATH  Google Scholar 

  18. Hughes TJR, Mazzei L, Oberai AA, Wray AA (2001) The multiscale formulation of large eddy simulation: decay of homogeneous isotropic turbulence. Phys Fluids 13:505–512. https://doi.org/10.1063/1.1332391

    Article  MATH  Google Scholar 

  19. Shishkin GI, Roos H-G, Stynes M, Tobiska L (1997) Numerical methods for singularly perturbed differential equations. Convection–diffusion and flow problems. Berlin etc., Springer-Verlag 1996. XVI, 348 pp., DM 148,00. ISBN 3-540-60718-8 (Springer Series in Computation. ZAMM J Appl Math Mech/Zeitschrift für Angew Math und Mech 77:403. https://doi.org/10.1002/zamm.19970770525

  20. Lin H, N Atluri S (2000) Meshless local Petrov–Galerkin (MLPG) Method for convection–diffusion problems. C Comput Model Eng Sci 1

  21. Ouyang J, Zhang L, Zhang X (2007) Nonstandard element free Galerkin method for solving unsteady convection dominated problems. Acta Aerodyn Sin 25:287–293

    Google Scholar 

  22. Khankham S, Luadsong A, Aschariyaphotha N (2015) MLPG method based on moving kriging interpolation for solving convection–diffusion equations with integral condition. J King Saud Univ Sci 27:292–301. https://doi.org/10.1016/j.jksus.2015.03.001

    Article  Google Scholar 

  23. Wu X-H, Dai Y-J, Tao W-Q (2012) MLPG/SUPG Method for convection-dominated problems. Numer Heat Transf Part B Fundam 61:36–51. https://doi.org/10.1080/10407790.2011.630962

    Article  Google Scholar 

  24. Hong CTWDLYHK (2014) Adaptive meshless local maximum-entropy finite element method for convection–diffusion problems, pp 189–200. https://doi.org/10.1007/s00466-013-0901-4

  25. Cyron CJ, Nissen K, Gravemeier V, Wall WA (2010) Information flux maximum-entropy approximation schemes for convection–diffusion problems. Int J Numer Methods Fluids 64:1180–1200. https://doi.org/10.1002/fld.2271

    Article  MathSciNet  MATH  Google Scholar 

  26. Nissen K, Wall WA (2016) Pressure-stabilized maximum-entropy methods for incompressible Stokes. Int J Numer Methods Fluids 82:35–56. https://doi.org/10.1002/fld.4205

    Article  MathSciNet  Google Scholar 

  27. Nissen K, Cyron C, Gravemeier V, Wall W (2012) Information-flux method: a meshfree maximum-entropy Petrov-Galerkin method including stabilised finite element methods. Comput Methods Appl Mech Eng s 241–244:225–237. https://doi.org/10.1016/j.cma.2012.05.015

    Article  MathSciNet  MATH  Google Scholar 

  28. Sukumar N (2005) Maximum entropy approximation

  29. Ortiz A, Puso MA, Sukumar N (2010) Maximum-entropy meshfree method for compressible and near-incompressible elasticity. Comput Methods Appl Mech Eng 199:1859–1871. https://doi.org/10.1016/j.cma.2010.02.013

    Article  MathSciNet  MATH  Google Scholar 

  30. Hughes TJR, Franca LP, Balestra M (1986) A new finite element formulation for computational fluid dynamics: V. Circumventing the babuška-brezzi condition: a stable Petrov–Galerkin formulation of the stokes problem accommodating equal-order interpolations. Comput Methods Appl Mech Eng 59:85–99. https://doi.org/10.1016/0045-7825(86)90025-3

    Article  MATH  Google Scholar 

  31. Franca LP, Hauke G, Masud A (2006) Revisiting stabilized finite element methods for the advective–diffusive equation. Comput Methods Appl Mech Eng 195:1560–1572. https://doi.org/10.1016/j.cma.2005.05.028

    Article  MathSciNet  MATH  Google Scholar 

  32. Hughes TJR, Franca LP, Hulbert GM (1989) A new finite element formulation for computational fluid dynamics: VIII. The galerkin/least-squares method for advective-diffusive equations. Comput Methods Appl Mech Eng 73:173–189. https://doi.org/10.1016/0045-7825(89)90111-4

    Article  MathSciNet  MATH  Google Scholar 

  33. Peddavarapu S, Raghuraman S (2020) Maximum entropy-based variational multiscale element-free Galerkin methods for scalar advection–diffusion problems. J Therm Anal Calorim. https://doi.org/10.1007/s10973-020-09845-y

    Article  Google Scholar 

  34. Sukumar N, Moran B, Belytschko T (1998) The natural element method in solid mechanics. Int J Numer Methods Eng 887:839–887

    Article  MathSciNet  Google Scholar 

  35. Leonard BP (1988) Simple high-accuracy resolution program for convective modelling of discontinuities. Int J Numer Methods Fluids 8:1291–1318. https://doi.org/10.1002/fld.1650081013

    Article  MATH  Google Scholar 

  36. Peco C, Millán D, Rosolen A, Arroyo M (2015) Efficient implementation of Galerkin meshfree methods for large-scale problems with an emphasis on maximum entropy approximants. Comput Struct 150:52–62. https://doi.org/10.1016/j.compstruc.2014.12.005

    Article  Google Scholar 

  37. Chen Z-J, Li Z-Y, Wu X-H, Tao W-Q (2018) A meshless local Petrov–Galerkin approach for solving the convection-dominated problems based on the streamline upwind idea and the variational multiscale concept. Numer Heat Transf Part B Fundam 73:19–32. https://doi.org/10.1080/10407790.2017.1420320

    Article  Google Scholar 

  38. Ortiz-bernardin A, Hale JS (2015) Cyron CJ Volume-averaged nodal projection method for nearly-incompressible elasticity using meshfree and bubble basis functions. Comput Method Appl Mech Eng 56:1–52

    MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Mechanical Engineering, SASTRA Deemed to be University, Thanjavur, 613401, India

    Sreehari Peddavarapu & Raghuraman Srinivasan

Authors
  1. Sreehari Peddavarapu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Raghuraman Srinivasan
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Sreehari Peddavarapu.

Ethics declarations

Conflict of interest

The authors declare that there is no conflict of interest.

Additional information

Technical Editor: Monica Carvalho.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Peddavarapu, S., Srinivasan, R. Local maximum entropy approximation-based streamline upwind Petrov–Galerkin meshfree method for convection–diffusion problem. J Braz. Soc. Mech. Sci. Eng. 43, 326 (2021). https://doi.org/10.1007/s40430-021-03038-w

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s40430-021-03038-w

Keywords

Search

Navigation