Anisotropic crystal–melt interfacial energy and stiffness of aluminum

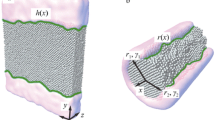
Abstract

The crystal–melt interfacial free energy is an important quantity governing many kinetic phenomena including solidification and crystal growth. Although general calculation methods are available, it is still difficult to obtain the interfacial energies that differ only slightly due to anisotropy. Here, we report such a calculation of Al crystal–melt interfacial energy based on the general framework of the capillary fluctuation method (CFM). The subtle dependence of both the melting temperature and interfacial free energy at melting temperature on the crystal interface orientation was examined. For Al, the average melting temperature is obtained at 934.79 ± 5 K and the orientationally averaged mean interfacial free energy is 98.35 mJ/m². In addition, the anisotropy of the interfacial free energy is found weak, nevertheless with the values ranked as γ₁₀₀ > γ₁₁₀ > γ₁₁₁.

APPENDIX: ERROR ANALYSIS

According to Eq. (1), the interfacial stiffness γ̃ is related to the size of the capillary fluctuation modes, the later terms can be obtained from simulation directly. Therefore, the error of γ̃ comes from the distributions of capillary fluctuation modes <∣A(k)∣² × k²>, which can be expressed as

$$A\left( k \right) = {a_k} + i{b_k}\quad .$$

(8)

Figure 6 illustrated the distributions of ∣A(k)∣ × k for (100)[001] orientation, picked out from 4000 interface profiles for each simulation. We found that except for the longest mode (K = 1), both a_k × k and b_k × k tend to be Gaussian, and the correlation coefficient r is close to 0, the statistical data are shown in Table IV.

FIG. 6

Distribution of interface fluctuation wave’s amplitude. The left figure represents the real part of the amplitude whereas the right one represents the imaginary part. The solid lines are Gaussian.

TABLE IV Distribution of interface fluctuation wave’s amplitude for (100)[001] orientations hypothesis testing.

We also conducted a Chi-square hypothesis testing for ∣A(k)∣ × k and found that most of the modes can be considered as 2D-Gaussian. In hypothesis testing, we used 6 × 6 inspection intervals, and the observations of samples χ² are shown in Table IV. If significance level α was selected as 5%, the quantile of χ_0.95(25) would be 37.652. It is found that for all modes except K = 1, ∣A(k)∣ × k follows 2D-Gaussian or similar distribution. This situation can be explained by different relaxation times, where the time correlation function42 can be expressed by

$$\left\langle {A\left( {k,t} \right){A^*}\left( {k,0} \right)} \right\rangle = \left\langle {{{\left| {A\left( k \right)} \right|}^2}} \right\rangle \exp \left[ { - {\rm{\mu \Gamma }}{k^2}t} \right]\quad ,$$

(9)

where μ is the kinetic coefficient and Γ = γ̃T_M/L_V, T_M denotes the melting temperature and L_V is the latent heat of fusion. Here, τ(t) is defined as

$$\tau \left( t \right) = {{\left\langle {A\left( {k,t} \right){A^*}\left( {k,0} \right)} \right\rangle } \over {\left\langle {{{\left| {A\left( k \right)} \right|}^2}} \right\rangle }}\quad .$$

(10)

In Table IV, the relaxation times are given when τ = 0.1, the value can be considered as there is no relation between two interface profiles. For the longest mode (K = 1), this value is 372 ps, which is very large in simulation time, lead to dependence of ∣A(k)∣ × k. For the short mode, this independence becomes very weak and the distribution of ∣A(k)∣ × k tends to be 2D-Gaussian.

From Table IV, the averages of a_k × k and b_k × k (μ_a, μ_b) are close to 0, it is easy to be explained by fluctuation. The distributions of a_k × k and b_k × k are expressed below

$${a_k} \times k \sim N\left( {0,{\rm{\sigma }}_a^2} \right)\quad ,$$

(11)

$${b_k} \times k \sim N\left( {0,{\rm{\sigma }}_b^2} \right)\quad ,$$

(12)

where σ_a² and σ_b² denote the variance of a_k × k and b_k × k, respectively. From the statistics one can acquire

$${1 \over {{\rm{\sigma }}_a^2}}\sum\limits_{i = 1}^n {a_k^2 \times {k^2} \sim {{\rm{\chi }}^2}\left( n \right)} \to N\left( {n,2n} \right)\quad ,$$

(13)

$${1 \over {{\rm{\sigma }}_b^2}}\sum\limits_{i = 1}^n {b_k^2 \times {k^2} \sim {{\rm{\chi }}^2}\left( n \right)} \to N\left( {n,2n} \right)\quad ,$$

(14)

and the distribution of <∣A(k)∣² × k²> would be

$$\left\langle {{{\left| {A\left( k \right)} \right|}^2} \times {k^2}} \right\rangle \sim N\left[ {\left( {{\rm{\sigma }}_a^2 + {\rm{\sigma }}_b^2} \right),{{2\left( {{\rm{\sigma }}_a^4 + {\rm{\sigma }}_b^4} \right)} \over n}} \right]\quad ,$$

(15)

where n is the number of samples, the value of 4000 in our work. From statistics, one can get the 95% confidence intervals of <∣A(k)∣² × k²> and γ̃(k) combining Eq. (1). Figure 7 shows the error bars of γ̃(k) for three orientations, by averaging the γ̃(k) at different k, the 95% confidence intervals of γ̃ can be acquired, as shown in Table II.

FIG. 7

Error analysis of stiffness of different interface orientations, error bars indicate the 95% confidence intervals. The symbols used here are for different interfaces: ■ for (100)[001] interface, ● for (110)[001] interface, and ▲ for (111) $\left[ {1\bar 10} \right]$ interface.

Moreover, the distribution of ∣A(k)∣² × k² should follow Boltzmann distribution coming from the derivation of Eq. (1) as:

$$p\left( {{{\left| {A\left( k \right)} \right|}^2} \times {k^2}} \right) = {{bL\tilde \gamma } \over {{k_{\rm{B}}}T}}\exp \left( { - {{\left| {A\left( k \right)} \right|}^2} \times {k^2} \times {{bL\tilde \gamma } \over {{k_{\rm{B}}}T}}} \right)\quad .$$

(16)

Indeed, a_k × k and b_k × k should obey the same distribution theoretically, with σ_a² = σ_b² = σ². Therefore, the distribution of ∣A(k)∣² × k² would be written as:

$$\frac{|A(k)|^2\times k^2}{\sigma^2} \sim \chi^2(2).$$

(17)

From Eq. (17), the probability density of ∣A(k)∣² × k² should be:

$$p\left( {{{\left| {A\left( k \right)} \right|}^2} \times {k^2}} \right) = {1 \over {2{{\rm{\sigma }}^2}}}\exp \left( { - {{{{\left| {A\left( k \right)} \right|}^2} \times {k^2}} \over {2{{\rm{\sigma }}^2}}}} \right)\quad .$$

(18)

By comparison of Eq. (18) with Eq. (16), it can be found that 2σ² = k_BT/bLγ̃. At the same time, the distribution of <∣A(k)∣² × k²> would be written as:

$$\left\langle {{{\left| {A\left( k \right)} \right|}^2} \times {k^2}} \right\rangle \sim N\left( {2{{\rm{\sigma }}^2},{{4{{\rm{\sigma }}^4}} \over n}} \right)\quad .$$

(19)

This equation illustrated the Gaussian distribution of <∣A(k)∣² × k²> in the case of σ_a² = σ_b², while the statistical data of these two parameters in our simulations are not necessarily the same (see Table IV). Consequently, we use Eq. (15) instead of (19) for error estimation.