Skip to main content
SpringerLink
Log in

New spectral techniques for systems of fractional differential equations using fractional-order generalized Laguerre orthogonal functions

  • Research Paper
  • Published:
Fractional Calculus and Applied Analysis Aims and scope Submit manuscript

Abstract

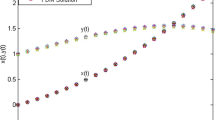
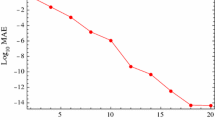
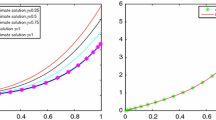
Fractional-order generalized Laguerre functions (FGLFs) are proposed depends on the definition of generalized Laguerre polynomials. In addition, we derive a new formula expressing explicitly any Caputo fractional-order derivatives of FGLFs in terms of FGLFs themselves. We also propose a fractional-order generalized Laguerre tau technique in conjunction with the derived fractional-order derivative formula of FGLFs for solving Caputo type fractional differential equations (FDEs) of order ν (0 < ν < 1). The fractional-order generalized Laguerre pseudo-spectral approximation is investigated for solving nonlinear initial value problem of fractional order ν. The extension of the fractional-order generalized Laguerre pseudo-spectral method is given to solve systems of FDEs. We present the advantages of using the spectral schemes based on FGLFs and compare them with other methods. Several numerical example are implemented for FDEs and systems of FDEs including linear and nonlinear terms. We demonstrate the high accuracy and the efficiency of the proposed techniques.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. A. Ahmadian, M. Suleiman, S. Salahshour, An operational matrix based on Legendre polynomials for solving fuzzy fractional-order differential equations. Abstr. Appl. Anal. 2013 (2013), Article ID 505903, 29 pages.

  2. D. Baleanu, A.H. Bhrawy, T.M. Taha, Two efficient generalized Laguerre spectral algorithms for fractional initial value problems. Abstr. Appl. Anal. 2013 (2013); doi:10.1155/2013/546502.

  3. D. Baleanu, A.H. Bhrawy, T.M. Taha, A modified generalized Laguerre spectral methods for fractional differential equations on the half line. Abstr. Appl. Anal. 2013 (2013); doi:10.1155/2013/413529.

  4. A.H. Bhrawy, M.M. Alghamdi, T.M. Taha, A new modified generalized Laguerre operational matrix of fractional integration for solving fractional differential equations on the half line. Adv. Differ. Equ. 2012 (2012), Article ID 179, 12 pages.

  5. A.H. Bhrawy, D. Baleanu, L.M. Assas, Efficient generalized Laguerrespectral methods for solving multi-term fractional differential equations on the half line. J. Vib. Contr. 20 (2014), 973–985.

    Article  MathSciNet  Google Scholar 

  6. A.H. Bhrawy, M.A. Alghamdi, A shifted Jacobi-Gauss-Lobatto collocation method for solving nonlinear fractional Langevin equation involving two fractional orders in different intervals. Boundary Value Problems 2012 (2012), Article ID 62, 13 pages.

    Google Scholar 

  7. A.H. Bhrawy, A Jacobi-Gauss-Lobatto collocation method for solving generalized Fitzhugh-Nagumo equation with time-dependent coefficients. Appl. Math. Comput. 222 (2013), 255–246.

    Article  MathSciNet  Google Scholar 

  8. A.H. Bhrawy, M.M. Al-Shomrani, A shifted Legendre spectral method for fractional-order multi-point boundary value problems. Adv. Differ. Equ. 2012 (2012), Article ID 8, 19 pages.

  9. C. Canuto, M.Y. Hussaini, A. Quarteroni, T.A. Zang, Spectral Methods in Fluid Dynamics. Springer-Verlag, New York, 1989.

    Google Scholar 

  10. M.D. Choudhury, S. Chandra, S. Nag, S. Das, S. Tarafdar, Forced spreading and rheology of starch gel: Viscoelastic modeling with fractional calculus. Colloid. Surface. A 407 (2012), 64–70.

    Article  Google Scholar 

  11. J. Deng, Z. Deng, Existence of solutions of initial value problems for nonlinear fractional differential equations, Appl. Math. Letters (2014); doi:10.1016/j.aml.2014.02.001.

    Google Scholar 

  12. K. Diethelm, N.J. Ford, Numerical solution of the Bagley-Torvik equation. BIT Numerical Mathematics 42, No 3 (2002), 490–507.

    MATH  MathSciNet  Google Scholar 

  13. E.H. Doha, A.H. Bhrawy, M.A. Abdelkawy, R.A. Van Gorder, Jacobi-Gauss-Lobatto collocation method for the numerical solution of 1 + 1 nonlinear Schrödinger equations. J. Comput. Phys. 261 (2014), 244–255.

    Article  MathSciNet  Google Scholar 

  14. E.H. Doha, A.H. Bhrawy, An efficient direct solver for multidimensional elliptic Robin boundary value problems using a Legendre spectral-Galerkin method. Comput. Math. Appl. 64 (2012), 558–571.

    Article  MATH  MathSciNet  Google Scholar 

  15. E.H. Doha, A.H. Bhrawy, R.M. Hafez, On shifted Jacobi spectral method for high-order multi-point boundary value problems. Commun. in Nonlinear Sci. and Numer. Simulation 17 (2012), 3802–3810.

    Article  MATH  MathSciNet  Google Scholar 

  16. E.H. Doha, A.H. Bhrawy, S.S. Ezz-Eldien, A new Jacobi operational matrix: an application for solving fractional differential equations. Appl. Math. Modell. 36 (2012), 4931–4943.

    Article  MATH  MathSciNet  Google Scholar 

  17. F. Flandoli, C.A. Tudor, Brownian and fractional Brownian stochastic currents via Malliavin calculus. J. Funct. Anal. 258 (2010), 279–306.

    Article  MATH  MathSciNet  Google Scholar 

  18. D. Funaro, Polynomial Approximations of Differential Equations. Springer-Verlag, 1992.

    Google Scholar 

  19. F. Gao, X. Lee, H. Tong, F. Fei, and H. Zhao, Identification of unknown parameters and orders via cuckoo search oriented statistically by differential evolution for noncommensurate fractional-order chaotic systems. Abstract and Applied Analysis 2013 (2013), Article ID 382834, 19 pages.

  20. A. Ghomashi, S. Salahshour, A. Hakimzadeh, Approximating solutions of fully fuzzy linear systems: A financial case study. Journal of Intelligent and Fuzzy Systems 26 (2014), 367–378.

    Google Scholar 

  21. M.H. Heydari, M.R. Hooshmandasl, F. Mohammadi, Legendre wavelets method for solving fractional partial differential equations with Dirichlet boundary conditions. Appl. Math. Comput. 234 (2014), 267–276.

    Article  MathSciNet  Google Scholar 

  22. M. Ishteva, L. Boyadjiev, On the C-Laguerre functions. C.R. Acad. Bulg. Sci. 58, No 9 (2005), 1019–1024.

    MATH  MathSciNet  Google Scholar 

  23. M. Ishteva, L. Boyadjiev, R. Scherer, On the Caputo operator of fractional calculus and C-Laguerre functions. Math. Sci. Res. 9, No 6 (2005), 161–170.

    MATH  MathSciNet  Google Scholar 

  24. Y.L. Jiang, X.L. Ding, Waveform relaxation methods for fractional differential equations with the Caputo derivatives. Comput. Math. Appl. 238 (2013), 51–67.

    Google Scholar 

  25. S. Kazem, S. Abbasbandy, S. Kumar, Fractional-order Legendre functions for solving fractional-order differential equations. Appl. Math. Model. 37 (2013), 5498–5510.

    Article  MathSciNet  Google Scholar 

  26. F. Gao, X. Lee, F. Fei, H. Tong, Y. Deng, H. Zhao, Identification timedelayed fractional order chaos with functional extrema model via differential evolution. Expert Systems with Applications 41 (2014), 1601–1608.

    Article  Google Scholar 

  27. R.L. Magin, C. Ingo, L. Colon-Perez, W. Triplett, T.H. Mareci, Characterization of anomalous diffusion in porous biological tissues using fractional order derivatives and entropy. Micropor. Mesopor. Mat. 178 (2013), 39–43.

    Article  Google Scholar 

  28. A. Saadatmandi, M. Dehghan, A new operational matrix for solving fractional-order differential equations. Comput. Math. Appl. 59 (2010), 1326–1336.

    Article  MATH  MathSciNet  Google Scholar 

  29. G. Szegö, Orthogonal Polynomials. Amer. Math. Soc. Colloq. Pub. 23, 1985.

  30. C. Yang, J. Hou, An approximate solution of nonlinear fractional differential equation by Laplace transform and Adomian polynomials. J. Inf. Comput. Sci. 10 (2013), 213–222.

    Google Scholar 

  31. A.M. Yang, Y.Z. Zhang, C. Cattani, G.N. Xie, M.M. Rashidi, Y.J. Zhou, X.-J. Yang, Application of local fractional series expansion method to solve Klein-Gordon equations on Cantor sets. Abstract and Applied Analysis 2014 (2014), Article ID 372741, 6 pages.

  32. F. Yin, J. Song, Y. Wu, L. Zhang, Numerical solution of the fractional partial differential equations by the two-dimensional fractional-order Legendre functions. Abstr. Appl. Anal. 2013 (2013), Article ID 562140, 13 pages.

  33. F. Yin, J. Song, H. Leng, F. Lu, Couple of the variational iteration method and fractional-order Legendre functions method for fractional differential equations. The Scientific World Journal 2014 (2014), Article ID 928765, 9 pages.

  34. Y. Zhao, D. Baleanu, C. Cattani, D.F. Cheng, X.-J. Yang, Maxwell’s equations on Cantor sets: A local fractional approach. Advances in High Energy Physics 2013 (2013), Article ID 686371, 6 pages.

  35. Y. Zhao, D.F. Cheng, X.-J. Yang, Approximation solutions for local fractional Schrodinger equation in the one-dimensional Cantorian system. Advances in Mathematical Physics 2013 (2013), Article ID 291386, 5 pages.

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Faculty of Science, King Abdulaziz University, Jeddah, 21589, Saudi Arabia

    Ali H. Bhrawy

  2. Department of Mathematics, Faculty of Science, Beni-Suef University, Beni-Suef, Egypt

    Ali H. Bhrawy

  3. Chemical and Materials Engineering Department, Faculty of Engineering, King Abdulaziz University, Jeddah, 21589, Saudi Arabia

    Yahia A. Alhamed, Dumitru Baleanu & Abdulrahim A. Al-Zahrani

  4. Department of Mathematics and Computer Sciences, Cankaya University, Ankara, Turkey

    Dumitru Baleanu

Authors
  1. Ali H. Bhrawy
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yahia A. Alhamed
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Dumitru Baleanu
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Abdulrahim A. Al-Zahrani
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Ali H. Bhrawy.

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Bhrawy, A.H., Alhamed, Y.A., Baleanu, D. et al. New spectral techniques for systems of fractional differential equations using fractional-order generalized Laguerre orthogonal functions. Fract Calc Appl Anal 17, 1137–1157 (2014). https://doi.org/10.2478/s13540-014-0218-9

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.2478/s13540-014-0218-9

MSC 2010

Key Words and Phrases

Search

Navigation