Abstract
Radial stress superposed bending is a sheet metal bending process, which superposes predetermined radial stresses. Stress superposition is mandatory to enable the reduction of the triaxiality in bending, resulting in delayed damage evolution and an improved product performance. The knowledge of the stress state is essential for damage-controlled bending as the triaxiality is the driving force for the void evolution. To control the stress state in radial stress superposed bending, an additional counter force responsible for the pressure in the outer fiber is applied. To predict the effect of the counter force on the radial stress and the triaxiality an analytical model is proposed. The prediction of the reaction forces in the system is required for the process design and for the calculation of the stress superposition. The stress state for plane strain bending with stress superposition is derived, and pressure calculations are made using the theory of Hertz. The model and the assumptions are verified in numerical and experimental studies for various counter pressures and bending ratios. Finally, a discussion of the load path depending on the transient counter pressure is carried out and experimental evidence for a inhibited damage evolution due to stress superposition is given.

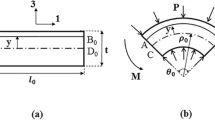
(Adopted from [6])
























Similar content being viewed by others
Abbreviations
- ε :
-
Plastic strain
- σii :
-
Principal stresses
- σf :
-
Flow stress
- σm :
-
Mean stress
- σvM :
-
Von Mises equivalent stress
- I i :
-
Stress invariants
- J i :
-
Deviatoric stress invariants
- η :
-
Stress triaxiality
- ξ :
-
Normalized third stress invariant
- \(\bar{\theta }\) :
-
Lode angle parameter
- L :
-
Lode parameter
- µ i :
-
Friction coefficient
- N i :
-
Normal forces
- T i :
-
Tangential forces
- φ i :
-
Contact angle of forces
- α :
-
Loaded bending angle
- r p :
-
Punch radius
- R bo :
-
Outer bending radius
- s i :
-
Distances between forces
- t :
-
Sheet thickness
- M b :
-
Bending moment
- d r :
-
Contact width of the radial stress
- p r :
-
Contact pressure
- b :
-
Sheet width
- h :
-
Punch stroke
References
McClintock, F. A. (1968). A criterion for ductile fracture by the growth of holes. Journal of Applied Mechanics, 35(2), 363–371.
Rice, J. R., & Tracey, D. M. (1969). On the ductile enlargement of voids in triaxial stress fields. Journal of the Mechanics and Physics of Solids, 17(3), 201–217.
Bao, Y., & Wierzbicki, T. (2004). A comparative study on various ductile crack formation criteria. Journal of Engineering Materials and Technology, 126(3), 314–324.
Wierzbicki, T., Bao, Y., Lee, Y.-W., & Bai, Y. (2005). Calibration and evaluation of seven fracture models. International Journal of Mechanical Sciences, 47(4–5), 719–743.
Anderson, D., Butcher, C., Pathak, N., & Worswick, M. J. (2017). Failure parameter identification and validation for a dual-phase 780 steel sheet. International Journal of Solids and Structures, 124, 89–107.
Roth, C. C., & Mohr, D. (2016). Ductile fracture experiments with locally proportional loading histories. International Journal of Plasticity, 79, 328–354.
Lange, K. (1990). Umformtechnik: Handbuch Für Industrie Und Wissenschaft. Berlin: Springer.
Kaupper, M., & Merklein, M. (2013). Bendability of advanced high strength steels—A new evaluation procedure. CIRP Annals, 62(1), 247–250.
Meier, H., Gänsicke, B., & Dewald, O. (2005). Erweiterung der Formgebungsgrenzen beim Walzrunden für wenig duktile hochfeste Werkstoffe. Wissenschaftliche Zeitschrift der Technischen Hochschule Otto von Guericke.
Cupka, V., Nakagava, T., & Tiyamoto, H. (1973). Fine bending with counter pressure. Annals of the CIRP, 22(22), 73–74.
El Budamusi, M., Isik, K., Chen, L., Clausmeyer, T., Becker, C., Tekkaya, A. E., & Doig, M. (2015). Enhanced damage modelling for sheet metal forming of high strength steels: Part 2—Application to forming processes. In 2nd European steel technology and application days, Düsseldorf, Germany.
Tekkaya, A. E., Ben Khalifa, N., Hering, O., Meya, R., Myslicki, S., & Walther, F. (2017). Forming-induced damage and its effects on product properties. CIRP Annals—Manufacturing Technology, 66(1), 281–284.
Meya, R., Löbbe, C., & Tekkaya, A. E. (2017). Vorrichtung Zum Blechbiegen Mittels Gezielter Druckspannungsüberlagerung. DE 10 2017 006 218.8.
Hertz, H. (1881). Über Die Berührung Fester Elastischer Körper. J.für die reine und angewandte Mathematik, 92, 156–171.
Meya, R., Löbbe, C., & Tekkaya, A. E. (2018). Stress state control by a novel bending process and its effect on damage evolution. In Proceedings of the 2018 manufacturing science and engineering conference MSEC, College Station, Texas.
Meya, R., Löbbe, C., Hering, O., & Tekkaya, A. E. (2017). New bending process with superposition of radial stresses for damage control. Enschede: Forming Technology Forum.
Wolter, K. H. (1952). Freies Biegen von Blechen,VDI-Forschungsheft 435, Düsseldorf, Germany.
Ludwik, P. (1903). Technologische Studie Über Blechbiegung. Technische Blätter (pp. 133–159).
Acknowledgements
The investigations are kindly supported by the German Research Foundation in context of the Collaborative Research Centre CRC/Transregio 188 “Damage-Controlled forming processes”, project A05. We thank Mr. Carl Kusche for his support in recording the SEM-micrographs.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Appendix 1
1.1 Friction Coefficient Declaration
-
µ1 Friction between lower bearing sheet and rotating tool
-
µ2 Friction between sheet and rotating tool
-
µ3 Friction between sheet and die
-
µ4 Friction between upper tools and sheet
-
µ5 Friction between upper tools and shell
Appendix 2
2.1 Determination of the Bending Angle
Determination of applied distances:
Determination of resulting angles:
Appendix 3
3.1 Upper Rotating Tools
The equilibrium of moments at the apparent punch center point C of one upper rotating parts (Fig. 10) leads to equation:
The two rotating upper parts can be considered as one FBD for the equilibrium of vertical forces as they are connected in a cylindrical bearing (Fig. 10). This leads to equation:
3.2 Lower Rotating Tools
The sum of the vertical forces leads to Eq. 31 and the sum of horizontal forces to Eq. 32
The equation for the equilibrium of moments around the apparent punch center point is given in equation:
Sheet
The equilibrium of vertical forces of the sheet leads to the equation:
Appendix 4
As the static system static indefinite, one equation of the elastic deflections is used. The groove in the rotating tool (Fig. 11, magnification) concentrates the force Nr to a pre-defined position, causing an elastic deformation within the bending legs (Fig. 26).
The forces Nb1 and Nb2 cause an elastic deflection in the bending direction, whereas the force Nl,n operates diametrically opposite. Contact points A and B, located between the sheet and the tool, are predetermined by the position in which the lower and upper tools lock the metal sheet. These are the locations in which the elastic deflections fA and fB are determined. The elastic deformation depends on the attacking forces at the metal sheet, the Young’s Modulus, the geometrical moment of inertia and the lever arms, leading to Eq. 38.
Through the preset tool geometry, the deflections fA and fB are predefined in such a way that they are situated on a line \({0\bar{A}B}\) (Fig. 26). With the given lever arms, the following equation arises:
4.1 Calculation of the Deflections
I = Area moment of Inertia
Deflection in Point A:
Deflection in Point B:
Rights and permissions
About this article
Cite this article
Meya, R., Löbbe, C. & Tekkaya, A.E. Stress State Analysis of Radial Stress Superposed Bending. Int. J. Precis. Eng. Manuf. 20, 53–66 (2019). https://doi.org/10.1007/s12541-019-00040-0
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s12541-019-00040-0